68-01 二叉树的最近公共祖先

题目

对于有根树 T 的两个结点 p、q，最近公共祖先表示为一个结点 x，满足 x 是 p、q 的祖先且 x 的深度尽可能大（一个节点也可以是它自己的祖先）。

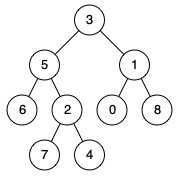
给定如下二叉树: root = [3,5,1,6,2,0,8,null,null,7,4]

LeetCode

C++ 题解

递归思想, 对以root为根的(子)树进行查找p和q, 如果root == null || p || q 直接返回root，表示对于当前树的查找已经完毕, 否则对左右子树进行查找, 根据左右子树的返回值判断:

1. 左右子树的返回值都不为null, 由于值唯一左右子树的返回值就是p和q, 此时root为LCA
2. 如果左右子树返回值只有一个不为null, 说明只有p和q存在与左或右子树中, 最先找到的那个节点为LCA
3. 左右子树返回值均为null, p和q均不在树中, 返回null

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode(int x) : val(x), left(NULL), right(NULL) {}
 * };
 */
class Solution {
public:
    TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {
        
        if(root == nullptr || root == p || root == q) 
            return root;
        
       TreeNode* left = lowestCommonAncestor(root->left,p,q);
       TreeNode* right = lowestCommonAncestor(root->right,p,q);
        
       if(left == nullptr && right == nullptr)
           return nullptr;
       else if(left != nullptr && right != nullptr)
            return root;
        else
        {
            return left == nullptr ? right : left;
        }
        
    }
};

python 题解

# Definition for a binary tree node.
# class TreeNode(object):
#     def __init__(self, x):
#         self.val = x
#         self.left = None
#         self.right = None

class Solution(object):
    def lowestCommonAncestor(self, root, p, q):
        """
        :type root: TreeNode
        :type p: TreeNode
        :type q: TreeNode
        :rtype: TreeNode
        """
        if root == None or root == p or root == q:
            return root
        
        left = self.lowestCommonAncestor(root.left,p,q)
        right = self.lowestCommonAncestor(root.right,p,q)
        
        if left == None and right == None:
            return None
        elif left != None and right !=None:
            return root
        else:
            if right:
                return right
            if left: 
                return left