poj 1654 Area （多边形求面积）

链接：http://poj.org/problem?id=1654

Area

Time Limit: 1000MS		Memory Limit: 10000K
Total Submissions: 14952		Accepted: 4189

Description

You are going to compute the area of a special kind of polygon. One vertex of the polygon is the origin of the orthogonal coordinate system. From this vertex, you may go step by step to the following vertexes of the polygon until back to the initial vertex. For each step you may go North, West, South or East with step length of 1 unit, or go Northwest, Northeast, Southwest or Southeast with step length of square root of 2.

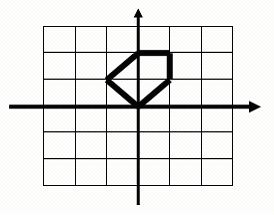
For example, this is a legal polygon to be computed and its area is 2.5:

Input

The first line of input is an integer t (1 <= t <= 20), the number of the test polygons. Each of the following lines contains a string composed of digits 1-9 describing how the polygon is formed by walking from the origin. Here 8, 2, 6 and 4 represent North, South, East and West, while 9, 7, 3 and 1 denote Northeast, Northwest, Southeast and Southwest respectively. Number 5 only appears at the end of the sequence indicating the stop of walking. You may assume that the input polygon is valid which means that the endpoint is always the start point and the sides of the polygon are not cross to each other.Each line may contain up to 1000000 digits.

Output

For each polygon, print its area on a single line.

Sample Input

Sample Output

-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=-=

又看了题解才A掉，记得自己曾对别人说WA不要马上看题解，一道题做两三天很正常，自己却MLE马上看题解

自己定的规则自己都不遵守

 1 #include <stdio.h>
 2 #include <string.h>
 3 #include <stdlib.h>
 4 #include <iostream>
 5 #include <algorithm>
 6 #include <math.h>
 7 
 8 #define MAXX 1000002
 9 #define eps 1e-6
10 typedef struct
11 {
12     double x;
13     double y;
14 } point;
15 
16 double crossProduct(point a,point b,point c)
17 {
18     return (c.x-a.x)*(b.y-a.y)-(c.y-a.y)*(b.x-a.x);
19 }
20 
21 bool dy(double x,double y)
22 {
23     return x>y+eps;
24 }
25 bool xy(double x,double y)
26 {
27     return x<y-eps;
28 }
29 bool dd(double x,double y)
30 {
31     return fabs(x-y)<eps;
32 }
33 
34 int main()
35 {
36     int n,m,i,j,x,y;
37     scanf("%d",&n);
38     char str[MAXX];
39     int move[10][2]= {{0,0},{-1,-1},{0,-1},{1,-1},{-1,0},{0,0},{1,0},{-1,1},{0,1},{1,1}};
40     for(i=0; i<n; i++)
41     {
42         scanf("%s",str);
43         int len=strlen(str);
44         int x1=0,y1=0,x2,y2;
45         long long ans=0;
46         for(j=0; j<len-1; j++)
47         {
48             x2=x1+move[str[j]-'0'][0];
49             y2=y1+move[str[j]-'0'][1];
50             ans+=((x1*y2)-(x2*y1));
51             x1=x2;
52             y1=y2;
53         }
54         ans = ans > 0 ? ans : (-1)*ans;
55         if(ans == 0)
56             printf("0\n");
57             else if(ans % 2 == 0)
58             printf("%lld\n",ans/2);
59             else if(ans % 2 != 0)
60             printf("%lld.5\n",ans/2);
61     }
62 return 0;
63 }