二阶系统为复数特征值时的极坐标表示
在看Hassan K.Khalil的Nonlinear Systems时,对于线性二阶系统特征值为复数时的极坐标表示这一小段,书上没有推导,现在补习一下:
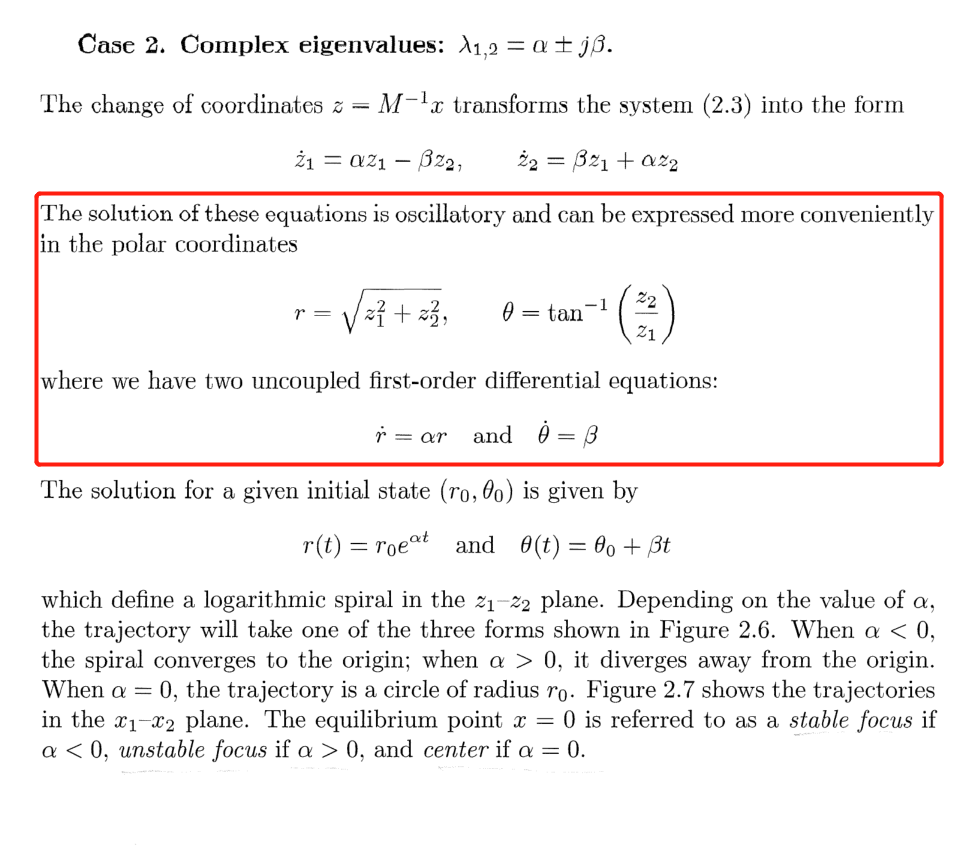
令 \(z_{1}=r\cos\theta\),\(z_{2}=r\sin\theta\),则 \(r=\sqrt{{z_{1}^{2}+z_{2}^{2}}}\),\(\theta=\tan^{-1}(\frac{z_{2}}{z_{1}})\)
代入 \(\dot{z}_{1}=\alpha z_{1}-\beta z_{2}\),\(\dot{z}_{2}=\beta z_{1}+\alpha z_{2}\),得
\[\dot{r}\cos\theta-r\dot{\theta}\sin\theta=\alpha r\cos\theta-\beta r\sin\theta \tag{1}
\]
\[\dot{r}\sin\theta+r\dot{\theta}\cos\theta=\beta r\cos\theta+\alpha r\sin\theta \tag{2}
\]
\((1)\times\cos\theta+(2)\times\sin\theta\) 得
\[\dot{r}=\alpha r
\]
\((2)\times\cos\theta-(1)\times\sin\theta\) 得
\(r\dot{\theta}=\beta r\) 即 $$\dot{\theta}=\beta$$



