827. Making A Large Island

这题很奇怪，一样的解法在中国区力扣就一直超时，在美国账号上就能accept，但就是时间复杂度不怎么好看。

827. Making A Large Island

You are given an n x n binary matrix grid. You are allowed to change at most one 0 to be 1.

Return the size of the largest island in grid after applying this operation.

An island is a 4-directionally connected group of 1s.

Example 1:

Input: grid = [[1,0],[0,1]]
Output: 3
Explanation: Change one 0 to 1 and connect two 1s, then we get an island with area = 3.

Example 2:

Input: grid = [[1,1],[1,0]]
Output: 4
Explanation: Change the 0 to 1 and make the island bigger, only one island with area = 4.

Example 3:

Input: grid = [[1,1],[1,1]]
Output: 4
Explanation: Can't change any 0 to 1, only one island with area = 4.

Constraints:

• n == grid.length
• n == grid[i].length
• 1 <= n <= 500
• grid[i][j] is either 0 or 1.

题意：给一个矩阵，由0和1组成，问最多可以将一个0翻成1，形成岛屿最大面积是多少。

思路：1， 用DFS来做

　　   2， 遍历矩阵，找到所有连通块，并用负整数r记录是否访问过

　　   3， 用hashmap来存每个连通块的面积

　　　4，遍历到每个位置0时，查询与其连通的连通块总面积，trick: 可能与同一个“0”位置相连的是同一个连通块，因此要用一个unordered_set来去重

　　　5，取最大值。

　　   6，时间复杂度为O（4*M*N），不明白为什么中国区力扣会超时，北美区的就不会。

以图为证：

1，原始矩阵：

 2， 遍历后的矩阵

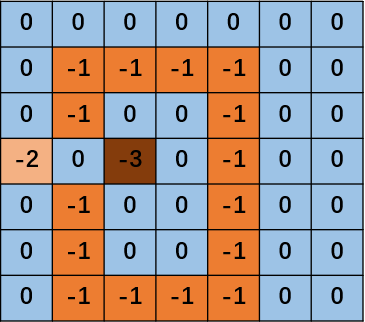
 其中hashmap中记录：m[-1] = 16,  m[-2] = 1, m[-3] = 1

3,遍历到grid[3][2]的时候，深搜到grid[3][1]（即下图红色块）时发现有两个连通块与其相连(next to it)，记录其面积和为next = m[-1]+m[-2]=17

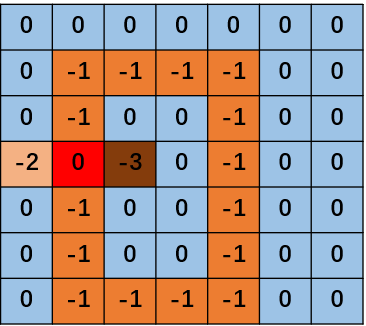
4，因此计算与红色块0连通的面积为1+17=18.

代码：

class Solution {
private:
    unordered_map<int, int> m;
    vector<vector<int>> dir = {{1,0},{-1,0},{0,1},{0,-1}};
public:
    void addNext(vector<vector<int>>& grid, int i, int j, unordered_set<int>& s, int& n){
        if(i<0 || i==grid.size() || j<0 || j==grid[0].size() || grid[i][j] >= 0 || s.count(grid[i][j])) return;
        s.insert(grid[i][j]);
        n += m[grid[i][j]];
    }
    void DFS(vector<vector<int>>& grid, int& r, int i,int j, int& cur, int& next){
        if(i<0 || i==grid.size() || j<0 || j==grid[0].size() || grid[i][j] < 0) return;
        if(grid[i][j] == 0){
            unordered_set<int> s;
            int n = 0;
            for(auto d : dir) addNext(grid, i+d[0], j+d[1], s, n);
            next = max(next, n);
        } else {
            grid[i][j] = r;
            cur++;
            for(auto d : dir) DFS(grid, r, i+d[0], j+d[1], cur, next);
        }
    }
    int largestIsland(vector<vector<int>>& grid) {
        int res = 1, r = 0, size = grid.size()*grid[0].size();
        for(int i=0; i<grid.size(); i++){
            for(int j=0; j<grid[0].size(); j++){
                if(grid[i][j] != 1) continue;
                int cur = 0, next = 0;
                DFS(grid, --r, i, j, cur, next);
                res = max(res, cur+next+1);
                m[r] = cur;
            }
        }
        return min(res, size);
    }
};

　优化了一下，4用vector来代替unordered_set来去重，终于accept了

class Solution {
private:
    unordered_map<int, int> m;
    vector<vector<int>> dir = {{1,0},{-1,0},{0,1},{0,-1}};
public:
    void DFS(vector<vector<int>>& grid, int& r, int i,int j, int& cur, int& next){
        if(i<0 || i==grid.size() || j<0 || j==grid[0].size() || grid[i][j] < 0) return;
        if(grid[i][j] == 0){
            vector<int> v(4, 2);
            int n = 0;
            for(int k=0; k<4; k++){
                int i1=i+dir[k][0], j1 = j+dir[k][1];
                if(i1<0 || i1==grid.size() || j1<0 || j1==grid[0].size() || grid[i1][j1] >=0) continue;
                v[k] = grid[i1][j1];
                if(v[k] != v[(k+1)%4] && v[k] != v[(k+2)%4] && v[k] != v[(k+3)%4]) 
                    n += m[v[k]];
            }
            next = max(next, n);
        } else {
            grid[i][j] = r;
            cur++;
            for(auto d : dir) DFS(grid, r, i+d[0], j+d[1], cur, next);
        }
    }
    int largestIsland(vector<vector<int>>& grid) {
        int res = 1, r = 0, size = grid.size()*grid[0].size();
        for(int i=0; i<grid.size(); i++){
            for(int j=0; j<grid[0].size(); j++){
                if(grid[i][j] != 1) continue;
                int cur = 0, next = 0;
                DFS(grid, --r, i, j, cur, next);
                res = max(res, cur+next+1);
                m[r] = cur;
            }
        }
        return min(res, size);
    }
};