『学习笔记』网络最大流

2018/9/3 14:55 Update

今天做了一道题 Luogu P1231

发现自己的 Dinic 写的很慢，居然超时 7 个点，于是决定加一些优化。

然后就在题解中看到了一种这样的写法

inline int Dinic(int u, int cap) {
    if(u == t || !cap) return cap;
    int delta, ret = 0;
    for(int i=head[u]; i; i=ed[i].nxt) {
        if(ed[i].w > 0 && Depth[ed[i].v] == Depth[u] + 1) {
            delta = Dinic(ed[i].v, min(ed[i].w, cap-ret));
            if(delta > 0) {
                ed[i].w -= delta;
                ed[i^1].w += delta;
                ret += delta;
            }
        }
    }
    return ret;
}

所以来更新一下这篇文章。这样写确实跑的很快。

1231 这道题直接过了。

然后又去试了试网络最大流的模板 Luogu P3376.

已经是无话可说。

两张图为证：

这是改之前的评测结果

这是改之后的评测结果

下面放上完整的模板，底下的那个就留着给大家观赏好了

#include <iostream>
#include <cstring>
#include <cstdio>
#include <queue>
#define INF 2147483647
using namespace std;
const int maxnode = 1e4+3, maxedge = 2e5+3;
inline int read() {
    int x = 0, f = 1; char c = getchar();
    while (c < '0' || c > '9') {if(c == '-') f = -1; c = getchar();}
    while (c <= '9' && c >= '0') {x = x*10 + c-'0'; c = getchar();}
    return x * f;
}
int n, m, s, t, head[maxnode], cnt=1, Depth[maxnode], Ans;
struct Edge {
    int nxt, v, w;
}ed[maxedge];
inline void addedge(int u, int v, int w) {
    ed[++cnt].nxt = head[u];
    ed[cnt].v = v, ed[cnt].w = w;
    head[u] = cnt;
}
inline bool BFS() {
    memset(Depth, 0, sizeof(Depth));
    queue <int> Q;
    Depth[s] = 1, Q.push(s);
    while (!Q.empty()) {
        int u = Q.front();
        Q.pop();
        for(int i=head[u]; i; i=ed[i].nxt) {
            if(ed[i].w > 0 && Depth[ed[i].v] == 0) {
                Q.push(ed[i].v);
                Depth[ed[i].v] = Depth[u] + 1;
                if(ed[i].v == t) return true;
            }
        }
    }
    return false;
}
inline int Dinic(int u, int cap) {
    if(u == t || !cap) return cap;
    int delta, ret = 0;
    for(int i=head[u]; i; i=ed[i].nxt) {
        if(ed[i].w > 0 && Depth[ed[i].v] == Depth[u] + 1) {
            delta = Dinic(ed[i].v, min(ed[i].w, cap-ret));
            if(delta > 0) {
                ed[i].w -= delta;
                ed[i^1].w += delta;
                ret += delta;
            }
        }
    }
    return ret;
}
int main() {
    n = read(), m = read(), s = read(), t = read();
    static int x, y, z;
    for(register int i=1; i<=m; i++) {
        x = read(), y = read(), z = read();
        addedge(x, y, z), addedge(y, x, 0);
    }
    while (BFS()) Ans += Dinic(s, INF);
    printf("%d\n", Ans);
}

以下为原文内容

我知道学这种东西有个图辅助理解更好，所以我尽量把图画好。flag

简介 & 一些定义

网络流，顾名思义是个流。是个怎么样子的流？

下面我们通过一个形象的比喻（fAke）来了解一下。有一种高端大气上档次的操作叫扯淡

就像是有很多管道，既然是管道那肯定有容量，这些管道链接着水厂和用水户。水厂通过管道将水流进用水户家中，不过水厂滞销（好像并不会出现，这只是一个假设），为了不浪费水，老板决定免费送水。而老板只有一个用水户？？！！所以他需要在不撑爆管道的情况下最大化流到用水户家里的水。

呵呵，我都被自己的比喻吓到了，不过说的应该很形象的吧

在上面这个比喻中水厂只能流出水，我们称其为源点，而用水户只能流入水，我们称其为汇点，其他的点既能流入也能流出，我们称其为（对不起，该字体无法显示我也不知道叫啥）。

管道的容量就叫容量，必须保证流过的水的量不能超过容量。但有的时候流过水的量会小于管道的容量，这个管道就会剩余一些容量，我们将这些剩余的容量成为残量。

将这个问题的一个合法解称之为流。一条边上水流过的量称为这条边的流量。

0x01

这个问题如何来解决，我们自然而然的就能想到一种错误的方法。如下：

源点我们用 $S$ 来表示，汇点用 $T$ 表示。

每次任意选择一条 $S\rightarrow T$ 的路径。将这条路径上所有边的容量都减去容量最小值。我们称这样的操作为增广。

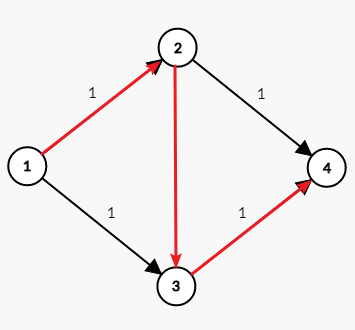
结合图片来理解一下 $0/1$ 代表容量是 $1$ ,水流过的量是 $0$ 。下图中我们选择的是 $1\rightarrow 2\rightarrow 3 \rightarrow 4$ 这条路径。

这条路径的流量为 $1$ 。刚刚已经说过这是个错误的解法，这张图的最大流并不是 $1$ 。而是 $2$ ，走 $1\rightarrow 2\rightarrow 4$ 和 $1\rightarrow 3\rightarrow 4$ 这两条路径。如第二张图所示。

问题就出在 $2\rightarrow 3$ 这条边上。从第三张图可以看出来。

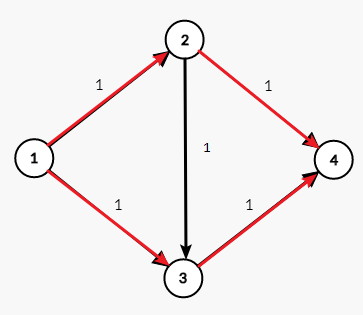
如何解决这个问题，我们需要引入一种很骚气的操作叫做反悔。在走了 $2\rightarrow 3$ 这条边之后我们发现这条路不对，我们反悔再折回去走 $3\rightarrow 2$ 像下面第一张图，那就会有人说了。我们建的不是有向图吗。为啥还能跑回去啊？

其实我们在建有向图的时候同时建立反边。反边具有一个性质，反边流量的相反数等于正边的流量。这一点怎么理解？其实就是反悔的时候把流过去的水又流了回来。

再往下一直找别的路径就找到了这个图的最大流了。

上面就是通常所说的EK算法（全称自己百度吧）。这种算法的时间复杂度并不是很优。

下面看一下EK算法的代码

inline void EK() {
    int cap = INF;
    for(int i=t; i!=s; i=ed[pre[i]].u)    //这个pre数组是记录路径的一个前缀数组
        cap = min(cap, ed[pre[i]].w);     //找出这条路径上的最小的容量
    for(int i=t; i!=s; i=ed[pre[i]].u) {
        ed[pre[i]].w -= cap;              //正向边的容量减
        ed[pre[i]^1].w += cap;            //反向边的容量加
    }
    max_flow += cap;                      //记录最大流
}

0x02

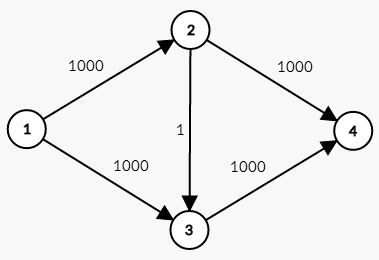
下面看一种针对EK算法的极限数据，如下图。

不难看出这个图的最大流是 $2000$ 。

假设第一次增广是我们选择了 $1\rightarrow 2\rightarrow 3\rightarrow 4$ 这条路径。第一次增广完之后就变成了这个样子

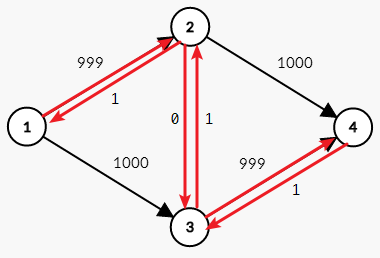
不出意外下一次增广选择了 $1\rightarrow 3\rightarrow 2\rightarrow 4$这条路。

重复这一过程，需要很多次才能将 $2000$ 算出来。为了减少这种无聊的操作。我们引入了分层图的概念

在BFS寻找路径的时候记录节点的深度， $Depth(v)=Depth(u)+1$，这样就将图分为了一层一层的

这就是Dinic算法

先看下代码，还有BFS的代码

inline bool BFS() {
    memset(Depth, 0, sizeof(Depth));
    queue <int> Q;
    Depth[s] = 1, Q.push(s);                           //源点的深度初始化为1
    while (!Q.empty()) {
        int u = Q.front();
        Q.pop();
        for(int i=head[u]; i; i=ed[i].nxt) {
            if(ed[i].w > 0 && Depth[ed[i].v] == 0) {
                Q.push(ed[i].v);                       //记录节点深度
                Depth[ed[i].v] = Depth[u] + 1;
                if(ed[i].v == t) return true;          //还能找到可增广路径
            }
        }
    }
    return false;                                      //没有找的可增广路径
}

inline int Dinic(int u, int cap) {
    if(u == t) return cap;                             //流到了汇点
    int delta;
    for(int i=head[u]; i; i=ed[i].nxt) {
        if(ed[i].w != 0 && Depth[ed[i].v] == Depth[u] + 1) {
            delta = Dinic(ed[i].v, min(cap, ed[i].w));
            if(delta > 0) {                            //增广成功
                ed[i].w -= delta;                      //正边减
                ed[i^1].w += delta;                    //负边加
                return delta;                          //向上传递
            }
        }
    }
    return 0;                                          //没有增广成功
}

完整代码

#include <iostream>
#include <cstring>
#include <cstdio>
#include <queue>
#define INF 2147483647
using namespace std;
const int maxnode = 1e4+3, maxedge = 2e5+3;
inline int read() {
    int x = 0, f = 1; char c = getchar();
    while (c < '0' || c > '9') {if(c == '-') f = -1; c = getchar();}
    while (c <= '9' && c >= '0') {x = x*10 + c-'0'; c = getchar();}
    return x * f;
}
int n, m, s, t, head[maxnode], cnt=1, Depth[maxnode], Ans;
struct Edge {
    int nxt, v, w;
}ed[maxedge];
inline void addedge(int u, int v, int w) {
    ed[++cnt].nxt = head[u];
    ed[cnt].v = v, ed[cnt].w = w;
    head[u] = cnt;
}
inline bool BFS() {
    memset(Depth, 0, sizeof(Depth));
    queue <int> Q;
    Depth[s] = 1, Q.push(s);
    while (!Q.empty()) {
        int u = Q.front();
        Q.pop();
        for(int i=head[u]; i; i=ed[i].nxt) {
            if(ed[i].w > 0 && Depth[ed[i].v] == 0) {
                Q.push(ed[i].v);
                Depth[ed[i].v] = Depth[u] + 1;
                if(ed[i].v == t) return true;
            }
        }
    }
    return false;
}
inline int Dinic(int u, int cap) {
    if(u == t) return cap;
    int delta;
    for(int i=head[u]; i; i=ed[i].nxt) {
        if(ed[i].w != 0 && Depth[ed[i].v] == Depth[u] + 1) {
            delta = Dinic(ed[i].v, min(cap, ed[i].w));
            if(delta > 0) {
                ed[i].w -= delta;
                ed[i^1].w += delta;
                return delta;
            }
        }
    }
    return 0;
}
int main() {
    n = read(), m = read(), s = read(), t = read();
    static int x, y, z;
    for(register int i=1; i<=m; i++) {
        x = read(), y = read(), z = read();
        addedge(x, y, z), addedge(y, x, 0);
    }
    while (BFS()) Ans += Dinic(s, INF);
    printf("%d\n", Ans);
}

posted @ 2018-08-28 11:06 Mystical-W 阅读(527) 评论(1) 编辑收藏举报

刷新页面返回顶部

Mystical-W

『 学习笔记 』网络最大流