Sqrt(x)

Implement int sqrt(int x).

Compute and return the square root of x.

思路：二分查找法解决这道题

class Solution {
public:
    int sqrt(int x) {
        if(x<=1)
            return x;
        int low=1;
        int high=x;
        while(low<=high)
        {
            int mid=low+(high-low)/2;
            if(mid==x/mid)
                return mid;
            else if(mid<x/mid)
                low=mid+1;
            else
                high=mid-1;
        }
        return high;
    }
};

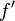 思路二：用牛顿求根法。首先，选择一个接近函数零点的，计算相应的和切线斜率（这里表示函数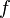的导数）。然后我们计算穿过点并且斜率为的直线和轴的交点的坐标，也就是求如下方程的解：

我们将新求得的点的坐标命名为，通常会比更接近方程的解。因此我们现在可以利用开始下一轮迭代。迭代公式可化简为如下所示：

已经证明，如果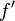是连续的，并且待求的零点是孤立的，那么在零点周围存在一个区域，只要初始值位于这个邻近区域内，那么牛顿法必定收敛。 并且，如果不为0, 那么牛顿法将具有平方收敛的性能. 粗略的说，这意味着每迭代一次，牛顿法结果的有效数字将增加一倍。

class Solution {
public:
    int sqrt(int x) {
        if(x<=1)
            return x;
        double a=x;
        double b=0;
        while(abs(b-a)>1e-6)
        {
            b=a;
            a=(b+x/b)/2;
        }
        return (int)a;
    }
};