遍历树，找出所有叶子路径

一、示例：

树的结构：

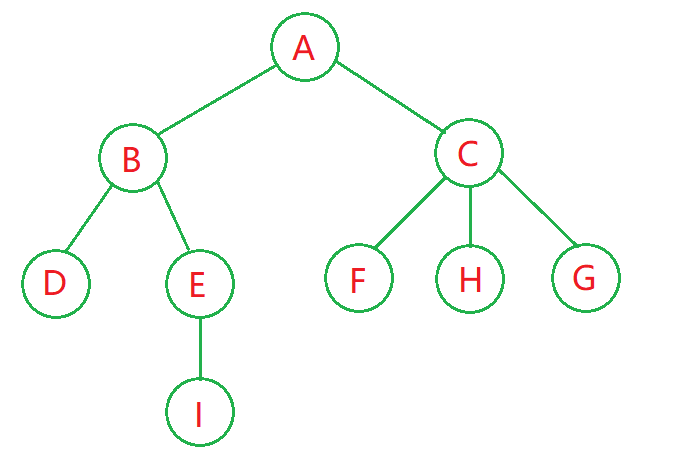
示例中自己构建了图片中的这棵树：

树节点模型：

public class TreeNode {
    String value;
    List<TreeNode> children;

    public TreeNode() {
        children = new ArrayList<>();
    }

    public TreeNode(String value) {
        this.value = value;
        children = new ArrayList<>();
    }

    @Override
    public String toString() {
        // TODO Auto-generated method stub
        return value;
    }
}

构建树：

// 创建一棵树
TreeNode root = new TreeNode("A");
// 第二层
root.children.add(new TreeNode("B"));
root.children.add(new TreeNode("C"));
// 第三层
root.children.get(0).children.add(new TreeNode("D"));
root.children.get(0).children.add(new TreeNode("E"));
root.children.get(1).children.add(new TreeNode("F"));
root.children.get(1).children.add(new TreeNode("H"));
root.children.get(1).children.add(new TreeNode("G"));
// 第四层
root.children.get(0).children.get(1).children.add(new TreeNode("I"));

二、遍历方式

提供三种方式进行遍历：

① 递归形式的深度优先遍历：

/**
     * 深度优先遍历（递归方式） --- 树（Tree）
     */
    public void recurTree(TreeNode root) {
        List<List<String>> result = new ArrayList<>();
        List<String> path = new ArrayList<>();
        path.add(root.value);
        findPath(result, root, path);
        System.out.println(result);
    }

    private void findPath(List<List<String>> result, TreeNode node, List<String> path) {
        if (node.children == null || node.children.size() <= 0) {
            result.add(path);
            return;
        }

        for (int i = 0; i < node.children.size(); i++) {
            TreeNode child = node.children.get(i);
            List<String> cPath = new ArrayList<>();
            cPath.addAll(path);
            cPath.add(child.value);
            findPath(result, child, cPath, target);
        }
    }

② 非递归的深度优先遍历

/**
     * 深度优先遍历（非递归方式） ----- 查找树的所有叶子路径
     * 
     * @param root
     *            根节点
     * @return 叶子路径的集合
     */
    public List<List<TreeNode>> dfsTree(TreeNode root) {
        Stack<TreeNode> nodeStack = new Stack<>();
        Stack<List<TreeNode>> pathStack = new Stack<>();
        List<List<TreeNode>> result = new ArrayList<>();
        nodeStack.push(root);
        ArrayList<TreeNode> arrayList = new ArrayList<>();
        arrayList.add(root);
        pathStack.push(arrayList);

        while (!nodeStack.isEmpty()) {
            TreeNode curNode = nodeStack.pop();
            List<TreeNode> curPath = pathStack.pop();

            if (curNode.children == null || curNode.children.size() <= 0) {
                result.add(curPath);
            } else {
                int childSize = curNode.children.size();
                for (int i = childSize - 1; i >= 0; i--) {
                    TreeNode node = curNode.children.get(i);
                    nodeStack.push(node);
                    List<TreeNode> list = new ArrayList<>(curPath);
                    list.add(node);
                    pathStack.push(list);
                }
            }
        }
        return result;
    }

3. 广度优先遍历，遍历所有叶子路径

/**
     * 广度优先遍历 ---- 查找树的所有叶子路径
     * 
     * @param root
     *            根节点
     * @return 叶子路径的集合
     */
    public List<List<TreeNode>> bfsTree(TreeNode root) {
        Queue<TreeNode> nodeQueue = new LinkedList<>();
        Queue<List<TreeNode>> qstr = new LinkedList<>();
        List<List<TreeNode>> result = new ArrayList<>();
        nodeQueue.add(root);
        ArrayList<TreeNode> arrayList = new ArrayList<>();
        qstr.add(arrayList);

        while (!nodeQueue.isEmpty()) {
            TreeNode curNode = nodeQueue.remove();
            List<TreeNode> curList = qstr.remove();

            if (curNode.children == null || curNode.children.size() <= 0) {
                curList.add(curNode);
                result.add(curList);
            } else {
                for (int i = 0; i < curNode.children.size(); i++) {
                    TreeNode treeNode = curNode.children.get(i);
                    nodeQueue.add(treeNode);
                    List<TreeNode> list = new ArrayList<>(curList);
                    list.add(curNode);
                    qstr.add(list);
                }
            }
        }

        return result;
    }

三种方式的输出：

深度优先遍历（递归）：[[A, B, D], [A, B, E, I], [A, C, F], [A, C, H], [A, C, G]]
广度优先遍历：[[A, B, D], [A, C, F], [A, C, H], [A, C, G], [A, B, E, I]]
深度优先遍历（非递归）：[[A, B, D], [A, B, E, I], [A, C, F], [A, C, H], [A, C, G]]

三、总结

　　示例是查找树的所有叶子节点，举一反三，如果我们是查找树中满足某个条件的路径，也是非常容易了。比如下面中查找 “ E ” 的分支：

public void recurTree(TreeNode root) {
        List<List<String>> result = new ArrayList<>();
        List<String> path = new ArrayList<>();
        path.add(root.value);
        findPath(result, root, path, "E");
        System.out.println(result);
    }

    private void findPath(List<List<String>> result, TreeNode node, List<String> path, String target) {
        if (target.equals(node.value)) {
            result.add(path);
            return;
        }

        if (node.children == null || node.children.size() <= 0) {
            return;
        }

        for (int i = 0; i < node.children.size(); i++) {
            TreeNode child = node.children.get(i);
            List<String> cPath = new ArrayList<>();
            cPath.addAll(path);
            cPath.add(child.value);
            if (result.size() > 0)
                break;
            findPath(result, child, cPath, target);
        }
    }

输出：

[[A, B, E]]