LuoguP3366 【模板】最小生成树

题目描述

如题，给出一个无向图，求出最小生成树，如果该图不连通，则输出orz

输入格式：

第一行包含两个整数N、M，表示该图共有N个结点和M条无向边。（N<=5000，M<=200000）
接下来M行每行包含三个整数Xi、Yi、Zi，表示有一条长度为Zi的无向边连接结点Xi、Yi

输出格式：

输出包含一个数，即最小生成树的各边的长度之和；如果该图不连通则输出orz

输入样例#1：

4 5
1 2 2
1 3 2
1 4 3
2 3 4
3 4 3

输出样例#1：

7

说明:

时空限制：1000ms,128M

数据规模：

对于20%的数据：N<=5，M<=20

对于40%的数据：N<=50，M<=2500

对于70%的数据：N<=500，M<=10000

对于100%的数据：N<=5000，M<=200000

样例解释：

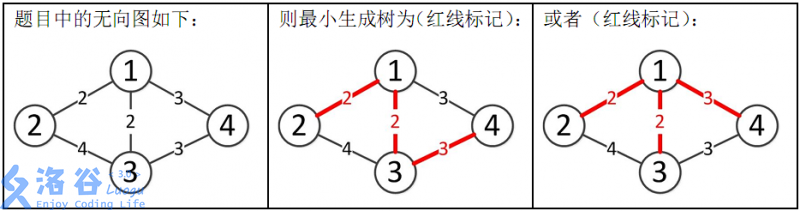
所以最小生成树的总边权为2+2+3=7

#include <iostream>
#include <cstdio>
#include <cstdlib>
#include <algorithm>
#include <cstring>
#include <queue>
#include <cmath>
using namespace std;
typedef long long ll;
struct edge {
    ll u, v, w;
} e[10000001];
bool cmp(const edge &x, const edge &y) { return x.w < y.w; }
ll fa[10000001], n, m, ans;
ll get(int x) {
    if (fa[x] == x)
        return x;
    return fa[x] = get(fa[x]);
}
void kruskal() {
    ll f1, f2, k = 0;
    for (int i = 1; i <= m; i++) {
        f1 = get(e[i].u);
        f2 = get(e[i].v);
        if (f1 != f2) {
            fa[f1] = f2;
            ans += e[i].w;
            k++;
            if (k == n - 1)
                break;
        }
    }
    if (k < n - 1)
        cout << "orz" << endl;
    else
        cout << ans << endl;
}
int main() {
    cin >> n >> m;
    for (int i = 1; i <= n; i++) fa[i] = i;
    for (int i = 1; i <= m; i++) {
        cin >> e[i].u >> e[i].v >> e[i].w;
    }
    sort(e + 1, e + m + 1, cmp);
    kruskal();
    return 0;
}