A natrual way to introduce homotopy groups

Contents

• Introduction
• Definition of homotopy groups
• Construction of cones
• Construction of path spaces
• Long exact sequences
• Cofibrations
• Fibrations
• Reference
• Recognization

Introduction

This post is a note about basis algebraic topology. In some book, given a topological space $X$ with point $x_0$, the $n$-th homotopy group

$$\pi_n(X,x_0)=\{\mathbb{S}^n\stackrel{\textrm{cont. }f}\to X: f(*)=x_0\}\bigg/\simeq, $$

where $f \simeq g$ iff there exists some map $\mathbb{S}^n\times [0,1]\to X$ (which is called homotopy) such that 

$$H(x,0)=f(x)\qquad H(x,1)=g(x)\qquad H(*,t)=x_0. $$Then it may be suprising that there exists long exact sequence of it. 

Notice. Homologic groups and homotopy groups are quit different, we cannot think homologic groups are the `abelization' of homotopy groups. For example, for torus $\mathbb{T}^2=\mathbb{S}^1\times \mathbb{S}^1$, the homologic groups are 

$$H_0(\mathbb{T}^2)=\mathbb{Z},\qquad H_1(\mathbb{T}^2)=\mathbb{Z}\oplus \mathbb{Z}, \qquad H_2(\mathbb{T}^2)=\mathbb{Z},\qquad H_{> 2}(\mathbb{T}^2)=0.$$

but (we omit the point for short)

$$\pi_0(\mathbb{T}^2)=\{*\},\qquad \pi_1(\mathbb{T}^2)=\mathbb{Z}\oplus \mathbb{Z}, \qquad \pi_2(\mathbb{T}^2)=0,  \qquad \pi_{> 2}(\mathbb{T}^2)=0 .$$

This means we should not expect naive relation between homologic groups and homotopy groups. Or, in other word, may be Hurewicz theorem is the only easy relation of them. You may think that they are all tools to ``count holes'', but actually, the homotopical holes are different from homological holes (for example, there is no $2$-dimensional homotopical hole in $\mathbb{T}^2$). 

To simplify the language, we call a pair $(X,x_0)$ a pointed topological space if $X$ is a topological space with $x_0\in X$. The morphism of $(X,x_0)\to (Y,y_0)$ is some continuous map $f:X\to Y$ such that $f(x_0)=y_0$. A homotopy of two morphisms $(X,x_0)\stackrel{f,g}\to (Y,y_0)$ is a continous map $H:X\times I\to Y$ such that $$H(x,0)=f(x)\qquad H(x,1)=g(x)\qquad H(*,t)=y_0. $$and denote (by abuse of notation, but actually common used) $f\stackrel{H}\simeq g$. Now, for any two pointed spaces $(X,x_0)$ and $(Y,y_0)$, we will denote $[X,x_0;Y,y_0]$ the set of homotopical classes of morphisms $(X,x_0)\to (Y,y_0)$. We say a map $f$ is null-homotopic if it is homotopic to constant map, and write by $f\simeq 0$.  

Definitions of homotopy groups

Given a pointed topological space $(X,x_0)$, we can construct the following spaces. 

• The suspension $(SX,*)$ to be the space $X\times \mathbb{S}^1$ after gluing $$X\times\{*\} \,\cup \,\{x_0\}\times \mathbb{S}^1$$ to a point, and pick $*$ to be this point. 

  

• The loop spces $(\Omega X,*)$ to be all the morphisms from $(\mathbb{S}^1,*)\to (X,x_0)$ and put $*$ the constant map. We equip the compact-open topology over this space (some topology good enough to ensure $(*)$ holds). 

   

Note that they are adjoint in this sense

$$[SX,*; Y,y_0]=[X,x_0; \Omega Y,*]\qquad \qquad {(*)}$$

Because 

$$\begin{array}{rll} {}[SX,*;Y,y_0] & =\{\textrm{htop cls of} SX\stackrel{f}\to Y: f(*)=y_0\} \\ & = \{\textrm{htop cls of} X\times  \mathbb{S}^1 \stackrel{f}\to Y: f(X\times\{*\} \,\cup \,\{x_0\}\times \mathbb{S}^1)=\{y_0\}\} \\ & = \{\textrm{htop cls of} X \stackrel{g}\to Y^{\mathbb{S}}: g(x_0)=\textrm{const} \textrm{and} [g(x)](*)=y_0, \} \\ & = \{\textrm{htop cls of} X \stackrel{g}\to \Omega Y: g(x_0)=\textrm{const}\} \\ & =[X,x_0,\Omega Y,y_0]\end{array}$$

Where $Y^{\mathbb{S}}$ means all the continuous map from $\mathbb{S}\to Y$.  Note that the middle $=$ is actually the expotential law,

• Given any function $X\times Y\stackrel{F}\to Z$, for any $x\in X$, $F(x,-)$ is a map $Y\to Z$, and this gives rise to $X\to Z^Y$.
• Conversely, Given $X\to Z^Y$, you can define a map $X\times Y\to Z$ by map $(x,y)$ be the image of $y$ under the map corresponding to $x$. 

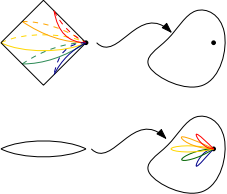
  And, to define the homotopy group, we need these easy to check facts

• $S(\mathbb{S}^{n},*)=(\mathbb{S}^{n+1},*)$.  
• $\mathbb{S}^0=\{-1,1\}$ and pointed by $1$. 

So, given a pointed topological space $(X,x_0)$, we can define

$$\pi_n(X,x_0)=[\mathbb{S}^n,*; X,x_0]. $$

Note that, $\pi_0(X,x_0)$ is just the path-connected component of $X$ (since $1$ is mapped to $x_0$, $-1$ is mapped arbitararily). 

If we denote $S^n =\underbrace{S\circ \ldots \circ S}_{\textrm{$n$ times}}$ and $\Omega^n=\underbrace{\Omega\circ \ldots \circ \Omega}_{\textrm{$n$ times}}$, then

$$\begin{array}{rl} \pi_n(X,x_0) & = [\mathbb{S}^n,*; X,x_0]= [\mathbb{S}^{n-1},*;\Omega X, *] \\ & =\ldots = [\{-1,1\},1; \Omega^n X,* ] \\ & = \textrm{path-conn comp. of $\Omega^n X$}.\end{array}$$

Construction of cones

Given a morphism of pointed topological space $(X,x_0)\stackrel{f}\to (Y,y_0)$, we can construct the following. 

• The cone $(C X,*)$ to be $[0,1]\times X$ after gluing $\{0\}\times X \cup I\times \{x_0\}$ to a point,  pointed by $(1,x_0)$. We use the map $x\mapsto (x,1)$ to identify $X$ as a subspace of $C X$. 

• The mapping cone $(\mathsf{cone} f,*)$ is the space $C X \sqcup Y$ by gluing each $x=(x,1)$ with $f(x)$, and point it by $x_0=(x_0,1)=f(x_0)$. 

   

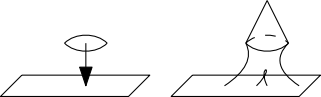
The most important thing is the following two facts 

Thereom. Given a morphism of pointed topological space $(X,x_0)\stackrel{f}\to (Y,y_0)$, 

• If $(X,y_0)\stackrel{g}\to (Z,z_0)$ is null-homotopy, then $g$ can extend to $CX$. 
• If $(Y,y_0)\stackrel{g}\to (Z,z_0)$ such that $g\circ f\simeq 0$, then $g$ can extend to $\mathsf{cone} f$. $$\begin{array}{ccccc}X & \to & Y & \to & \mathsf{cone} f \\ & \searrow & \downarrow &\swarrow  \\ & & Z & & \end{array}$$

Proof. By definition actually. (1) $0\stackrel{H}\simeq g$, then $H:X\times I\to Y$ with $\{0\}\times X \cup I\times \{x_0\}$ mapped to $y_0$. This induces the map $CX$. (2) follows by (1). $\square$

 As a corollary, we can get some ``exact sequence'' in some sense. 

Corollary. Given a morphism of pointed topological space $(X,x_0)\stackrel{f}\to (Y,y_0)$, the following sequence is exact

$$[X,x_0; Z,z_0]\leftarrow [Y,y_0; Z,z_0]\leftarrow [\mathsf{cone} f,*; Z,z_0]. $$

Where the exactness for $\stackrel{\varphi}\to A \stackrel{\psi}\to$ means the image of $\varphi$ is exact the inverse image of the distinguished point under $\psi$. In this case, all the distinguished points are chosen to be the constant map. 

Proof. The composition $\simeq 0$ trivially. And the exactness is just the translation of above theorem. $\square$

 Now, the task is to extend the sequence, so it is inevitible to find the mapping cone of mapping cone and the path space of path space. To be exact, 

Problem. We want to find the mapping cone of $(Y,y_0)\to (\mathsf{cone} f, *)$, and we will refer it by double mapping cone. 

 The answer may be quit striking. 

Answer. The double mapping cone has the same homotopy type with $SX$. 

I will not prove these two results, because it is actually proved by construction of some ugly map. But I want to draw a picture to demonstrate why it is true. 

 

 

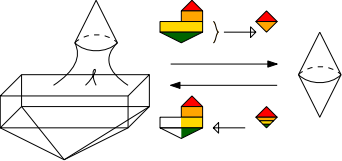
 They gives rise to homotopy equivalent because 

 

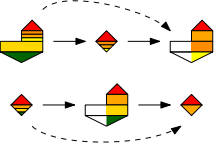
Construction of path spaces

 Given a morphism of pointed topological space $(X,x_0)\stackrel{f}\to (Y,y_0)$, we can construct the following. 

• The path space $(PY,*)$ to be all the morphisms from $(I,0)\to (X,x_0)$ and pointed by the constant map. We have a natural map $p=[w\mapsto w(1)]$ to indicate the end point of path. 

• The path space $(\mathsf{path} f,*)=\{(x,w)\in X\times PY : f(x)=w(1)\}$, that is, $\bigcup_{x\in X}\{\textrm{path in $Y$ from $f(x_0)$ to $f(x)$}\}$. The point is chosen the be $(x_0,\mathrm{cont})$. 

   

 Similarly, we have $[CX,*; Y,y_0]=[X,x_0; PY,*]$, and the following. 

 Thereom. Given a morphism of pointed topological space $(X,x_0)\stackrel{f}\to (Y,y_0)$, 

• If $(W,w_0)\stackrel{h}\to (Y,y_0)$ is null-homotopy, then $h$ can lift to $PY$. 
• If $(W,w_0)\stackrel{h}\to (X,x_0)$ such that $f\circ h \simeq 0$, then $h$ can lift to $\mathsf{path} f$.  $$\begin{array}{ccccc}&& W \\ & \swarrow & \downarrow & \searrow \\ \mathsf{path} f & \to & X & \to & Y \end{array}$$

Proof. (1) is the dual argument of cone argument. (2) follows by (1). $\square$ 

Corollary. Given a morphism of pointed topological space $(X,x_0)\stackrel{f}\to (Y,y_0)$, the following sequence is exact

$$[W,w_0; \mathsf{path} f,*] \to [W,w_0; X,x_0]\to [W,w_0; Y,y_0] . $$

Where the exactness for $\stackrel{\varphi}\to A \stackrel{\psi}\to$ means the image of $\varphi$ is exact the inverse image of the distinguished point under $\psi$. In this case, all the distinguished points are chosen to be the constant map. 

 Now, how about 

Question. What is the path space of $(\mathsf{path} f, *)\to (X,x_0)$, and we will call it by double path space? 

Dually, 

Solution. The double path space has the same homotopy type with $\OmegaY$. 

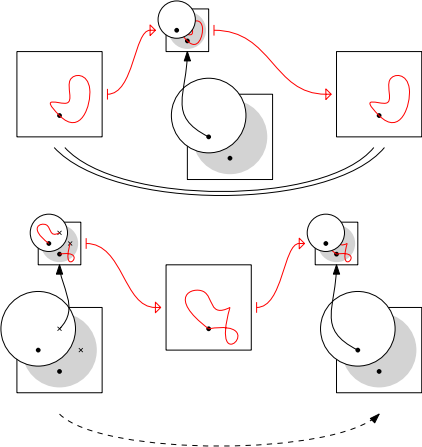
We can show that the double path space is $\{(x,w,w')\in X\times PY\times PX: f(x)=w(1),x=w'(1)\}$, that is, $\bigcup_{x\in X}\{\textrm{path in $Y$ from $f(x_0)$ to $f(x)$}\}\times \{\textrm{path in $X$ from $x_0$ to $x$}\}$. 

The homotopic equivalences are given by

 

 

 

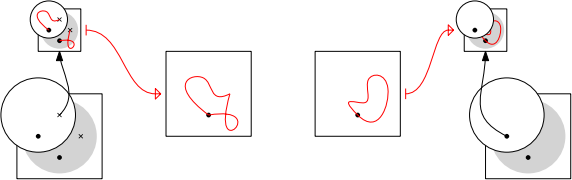
The composition look like 

It is not difficult to believe they work (believe means you believe you can write down the strick proof in finite time by yourself). 

Long exact sequences

Now we can construct long exact sequence use above construction. Given a morphism of pointed topological space $(X,x_0)\stackrel{f}\to (Y,y_0)$, we have the following exact sequence (the meaning of exactness is explained in theorem above) 

$$\begin{array}{ccccccc}& && \cdots & \to & [S^2 X,*;Z,z_0] & \to \\ \to & [S\mathsf{cone} f, * ;Z,z_0] & \to & [SY,*; Z,z_0] & \to &  [SX,*;Z,z_0] & \to \\ \to & [\mathsf{cone} f,*; Z,z_0] &\to & [Y,y_0;Z;z_0]& \to & [X,x_0;Z,z_0]\end{array}$$

and 

$$\begin{array}{ccccccc}& && \cdots & \to & [W,w_0; \Omega^2 Y,*] & \to \\ \to & [W,w_0 ;\Omega \mathsf{path} f,*] & \to & [W,w_0; \Omega X,*] & \to &  [W,w_0;\Omega Y,* ] & \to \\ \to & [W,w_0; \mathsf{path} f,*] &\to & [W,w_0;X;x_0]& \to & [W,w_0; Y,y_0]\end{array}$$

This is nice, but there are some sign problems. To make this clear, when constructing the double cone of $(Y,y_0)\stackrel{i}\to \mathsf{cone} f$, we have the following commuting diagram

$$\begin{array}{ccc}\mathsf{cone}^2 f & \to & \mathsf{cone}^2 i \\ \downarrow && \downarrow \\ SX& \underset{*}\to & SY\end{array}$$

where we write double cone by $\mathsf{cone}^2$ for short, and $*$ is actually homotopic to $\nu\circ Sf\simeq Sf\circ \nu$. where $$\nu: SX\longrightarrow SX \qquad (t,x)\mapsto (1-t,x)$$

the turing over mapping of the suspension. 

 

The similar problem to the path space, the difference is a inversion of path. 

The above construction is useful, we can fix the all morphism into a long exact sequence.

• Let $(A,x_0)\stackrel{i}\to (X,x_0)$ be an inclusion (that is $x_0\in A\subseteq X$), then we can consider 

  $$P(X;x_0,A)=\{[0,1]\stackrel{\textrm{cont.map } f}\to X: f(0)=x_0, f(1)\in A\}\qquad *=\textrm{const}$$

  Since $A\subseteq X$, the above is nothing but $\mathsf{path} i$. We define the ``relative homotopy group'' by $\pi_{n}(X,A,x_0)=\pi_{n-1}(P(X;x_0,A),*)$. Now, take $(W,w_0)=(\mathbb{S}^0,1)$, we get the beautiful long exact sequence 

  $$\begin{array}{ccccccc}& && \cdots & \to & \pi_2(X,x_0) & \to \\ \to & \pi_2(X,A;x_0) & \to & \pi_1(A,x_0) & \to & \pi_1(X,x_0) & \to \\ \to & \pi_1(X,A,x_0) &\to & \pi_0(A,x_0)& \to & \pi_0(X,x_0)\end{array}$$

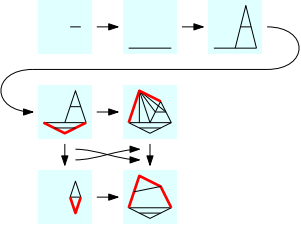
• For general case, $(X,x_0)\stackrel{f}\to (Y,y_0)$, we can construct so-call mapping cylinder. First denote $Cy\, X$ by the space $X\times I$ gluing $\{x_0\}\times I$ to a point, and define $\mathsf{cyl} f$ to be $Cy\, X\cup Y$ by identifying $(1,x)$ with $f(x)$. And $(Y,y_0)$ have same homotopy type with $\mathsf{cyl} f$, and by map $j=[X\ni x\mapsto (x,0)\in \mathsf{cyl} f]$ will homotopic to $f$ up to this homotopic equivalence. Now, $j$ is an inclusion. 

  

Of course, if we want to check it is an exact sequence of groups (at least, for $n\geq 1$), we need to check that the maps are all homomorphic. There are some theory on it, but is too much for this post. 

Cofibrations 

An important concept in algebraic topology is cofibration. You can read the definition in any algebraic topology text book (except Hatcher's), so is wikipedia. The most important fact is the following. 

Theorem. For any cofibration $(A,x_0)\subseteq (X,x_0)$, denote $(X/A,*)$ the space of $X$ gluing $A$ into a point $*$. Then $(X/A,*)$ is of same homotopy type with $\mathsf{cone} i$, with $i:(A,x_0)\to (X,x_0)$ the inclusion map. 

This means, roughly speaking, when $A\subseteq X$ is good enough, then $\pi_n(X,A,x_0)$ is nothing but $\pi_n(X/A,*)$. 

Fibrations

For the definition of fibration, you can read it in any algebraic topology text book (except Hatcher's), so is wikipedia. Note that fibre bundles (see wikipedia) are all weak fibration (may not be fibration, but satisfy all properties afterwards). We need this fact. 

Theorem. If $(E,e_0)\stackrel{p}\to (B,b_0)$ is a fibration and $(B',b_0)\subseteq (B,b_0)$, denote $(E',e_0)$ by $p^{-1}(B',b_0)$. Then the homomorphism $\pi_n(E,E',e_0)\to \pi_n(B,B',b_0)$ induced by $p$ is bijection. 

The proof is like some proofs in covering space. Now, take $B'=b_0$, we have $\pi_n(E,F,e_0)=\pi_n(B,b_0,b_0)$, where $F$ is the fibre of $p$. Note that $(P(B;b_0,b_0),*)=(\Omega B,*)$, thus $$\pi_n(B,b_0,b_0)=[\mathbb{S}^{n-1},*;P(B,b_0,b_0),*]=[\mathbb{S}^{n-1},*,\Omega B,*]=[\mathbb{S}^n,*,B,*]=\pi_n(B,b_0).$$So we have the following beatiful long exact sequence

$$\cdots \longrightarrow \pi_n(F,e_0)\to \pi_n(E,e_0)\to \pi_n(B,b_0)\longrightarrow\pi_{n-1}(F,e_0)\to \pi_{n-1}(E,e_0)\to \pi_{n-1}(B,b_0) \longrightarrow \cdots$$

But now, we need to make the map clear. 

• The first map $ \pi_n(F,e_0)\to \pi_n(E,e_0)$ is just the map induced by $F\subseteq E$. 

  

• The second map $ \pi_n(E,e_0)\to \pi_n(B,b_0)$ is just induced $p$, by the assertion of above. 

   

• The third map $\pi_n(B,b_0)\longrightarrow\pi_{n-1}(F,e_0)$ is misterious. We will discuss it in the rest of this paragrapg. 

Formly, it is 

$$\begin{array}{ccccccc}\pi_n(B,b_0) & = & \pi_n(B,b_0,b_0) & \cong &  \pi_n(E,F,e_0) & \to & \pi_{n-1}(F,e_0)\\ {}[(\mathbb{S}^n,*) \to (B,b_0)] & \mapsto  & [(\mathbb{S}^{n-1},*)\to (\Omega B,*)] & \textrm{lifts to} & [(\mathbb{S}^{n-1},*)\to (P(E; e_0,F),*)] & \mapsto &  [(\mathbb{S}^{n-1},*)\to (F,*)] \end{array}$$

If we view $\mathbb{S}^n$ as the disc $\mathbb{D}^n=[0,1]^n$ gluing along the boundary $\mathbb{S}^{n-1}$, then the map will be much easier. Note that

$$\begin{array}{rl}        {}[\mathbb{S}^{n-1},*; P(E;e_0,F),*] & =\{\textrm{htop cls } (\mathbb{S}^{n-1},*)\to (P(E;e_0,F),*)\}\\        & = \{\textrm{htop cls } \mathbb{S}^{n-1}\stackrel{f}\to F\times P E: \ldots \}\\        & =\{\textrm{htop cls }\mathbb{S}^{n-1}\stackrel{f_1}\to F\textrm{ and } \mathbb{S}^{n-1}\stackrel{f_2}\to PE: f_2(*)=\textrm{const}, \textrm{$f_1(s)$ is the end point of $f_2(s)$}  \}\\        & =\{\textrm{htop cls }\mathbb{S}^{n-1}\stackrel{f_1}\to F\textrm{ and } \mathbb{D}^{n}\stackrel{f_2}\to E: \textrm{the restriction of $f_2$ on $\mathbb{S}^{n-1}$ is $f_1$}  \}\\       & =\{\textrm{htop cls }\mathbb{D}^{n}\stackrel{f}\to E: f(\mathbb{S}^{n-1})\subseteq F\}     \end{array}$$

For the last equality, since all $f_2(s)$ are from $x_0$, so we can gluing $\mathbb{S}^{n-1}\times \{0\}$ in $\mathbb{S}^{n-1}\times [0,1]$ to a point. 

 

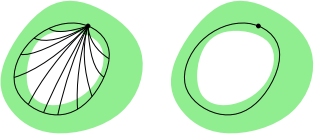
In the same way, we know that

$$[(\mathbb{S}^n,*) \to (B,b_0)]=\{\textrm{htop cls }\mathbb{D}^{n}\stackrel{f}\to B: f_2(\mathbb{S}^{n-1})\subseteq \{e_0\}\}$$

• Now, the mapping $\pi_n(B,b_0)\cong \pi_n(E,F,e_0)$ are given by lifting. More exactly, it is given by $[\mathbb{D}^n\stackrel{f}\to B]\mapsto [\mathbb{D}^n\stackrel{\hat{f}}\to E]$. 
• Now, the mapping $\pi_n(E,F,e_0)  \to  \pi_{n-1}(F,e_0)$ are given by taking boundry. More precisely, it will be like $[\mathbb{D}^n \stackrel{f}\to E]\mapsto [\mathbb{S}^{n-1}\stackrel{f|_{\cdots}}\to F]$. 

Now, all the mappings are clear. Note that the map here is similar to the homological connectioin map. 

Reference

Switzer. Algebraic Topology --- Homotopy and Homology. 

Recognization

Thanks Arsenty Kambalin for discussion.