【学习笔记】AC自动机
AC自动机
其实我将近三个月前就准备写这个并且随笔都建好了,但是一直咕咕到现在才写。其实记忆力好的同学应该意识到这篇其实8月份已经发过了,这次只是更新了一下发布日期而已
概述
AC自动机是 以 Trie 的结构为基础,结合 KMP 的思想建立的。
所以,建立AC自动机一般有两个步骤:
- 将所有的模式串构成一棵Trie。
- 对Trie树上的所有节点构造失配指针。
然后就可以利用它进行多模式串匹配了。
至于多模式串匹配,用不怎么专业的话概述就是:
对于给定的文本串,找出每个模式串在其中各出现了几次。
构建字典树
和Trie树的插入一模一样,就建立一棵普通的Trie树就行。注意当模式串有相同的时候,要对其标记“去重”。
Code
void Insert(char *s, int id){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; if(!ch[u][v]) ch[u][v] = ++sz; u = ch[u][v]; } if(!val[u]) val[u] = id; //上文提及的“去重” pos[id] = val[u]; }
失配指针
AC自动机利用 指针来辅助多模式串的匹配。
那 指针的含义是?
设 到点 的字符串为 ,点 的 指针指向 , 到点 的字符串为 。那 是 的最长后缀。所以, 指针是 当前字符串最长的后缀的末尾编号。
反正记住AC自动机的失配指针指向当前状态的最长后缀状态即可。
构建
构建 指针的 基础思想 (别真的这么写,会T飞的):
参考KMP构建 指针的思想。考虑字典树中当前节点为 ,其父节点为 , 通过字符 的边指向 ,即 ,假设所有深度小于等于 的节点的 指针都已求得。
- 如果 存在,使得 指向 ,相当于在 和 之后都缀上一个字符 ,分别对应 和 。
- 如果 不存在,那一直找 ,继续判断,直到跳到 。
- 真的不存在,啥也没有,让 指针跳到根节点。
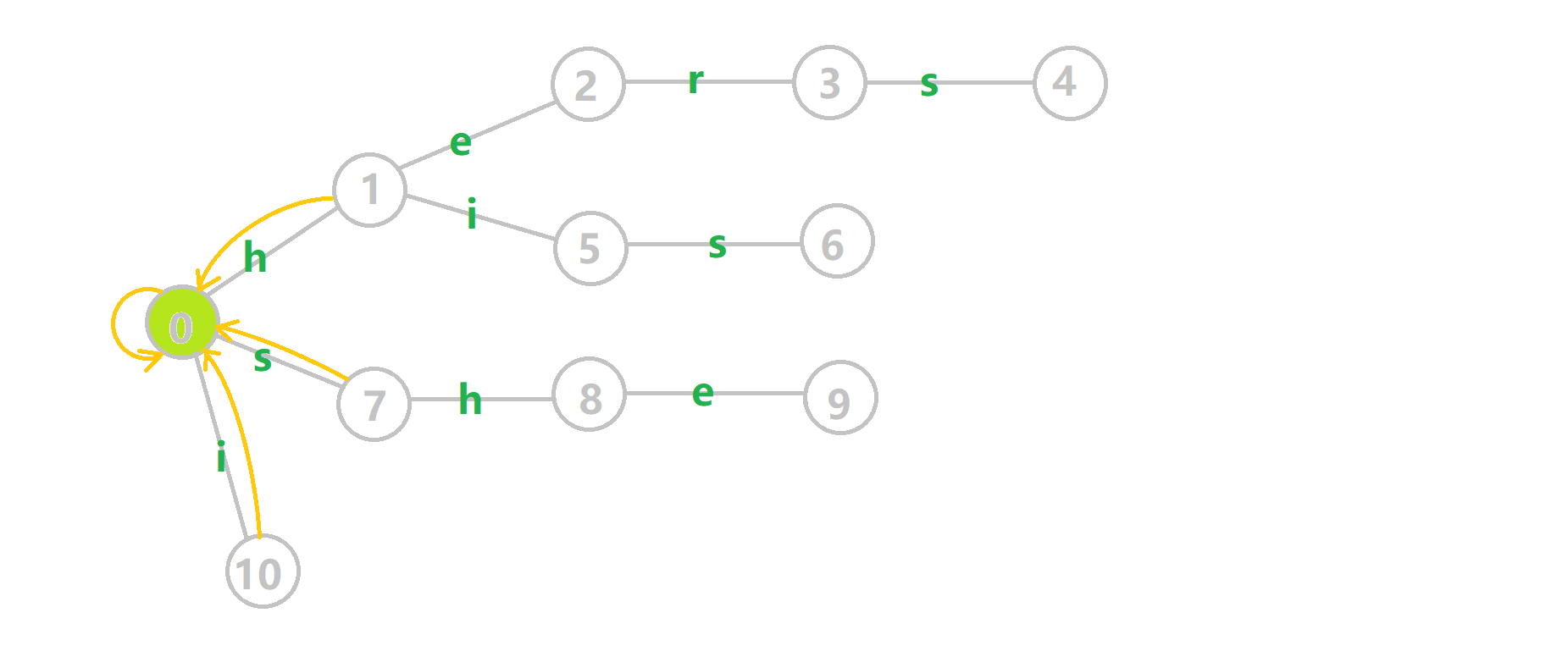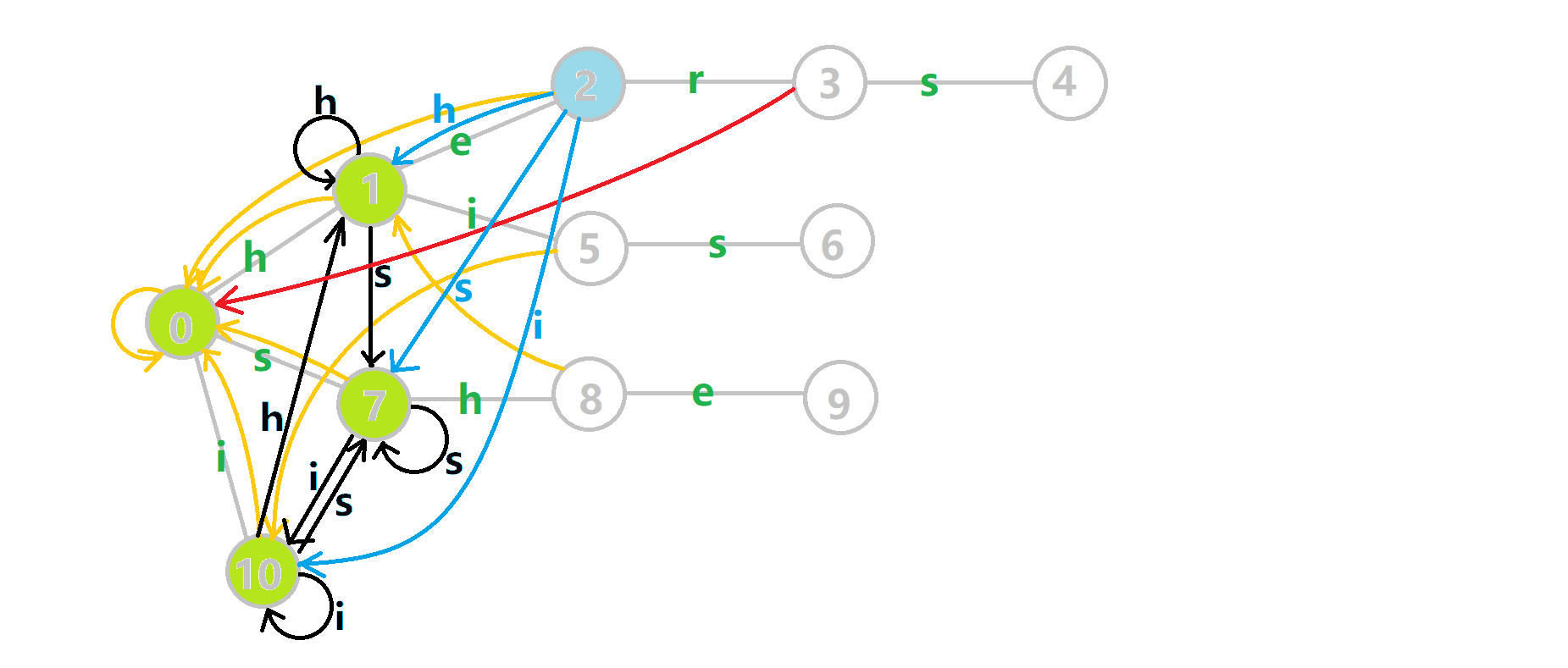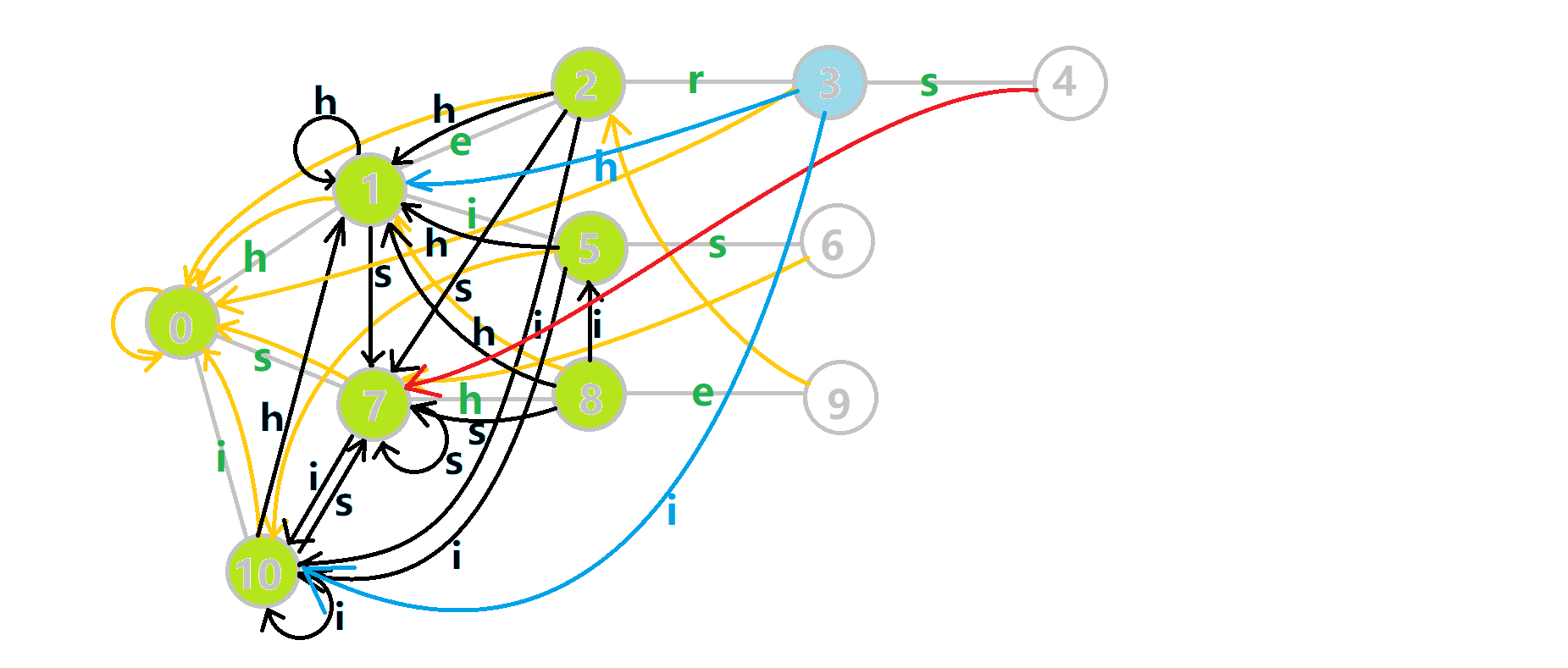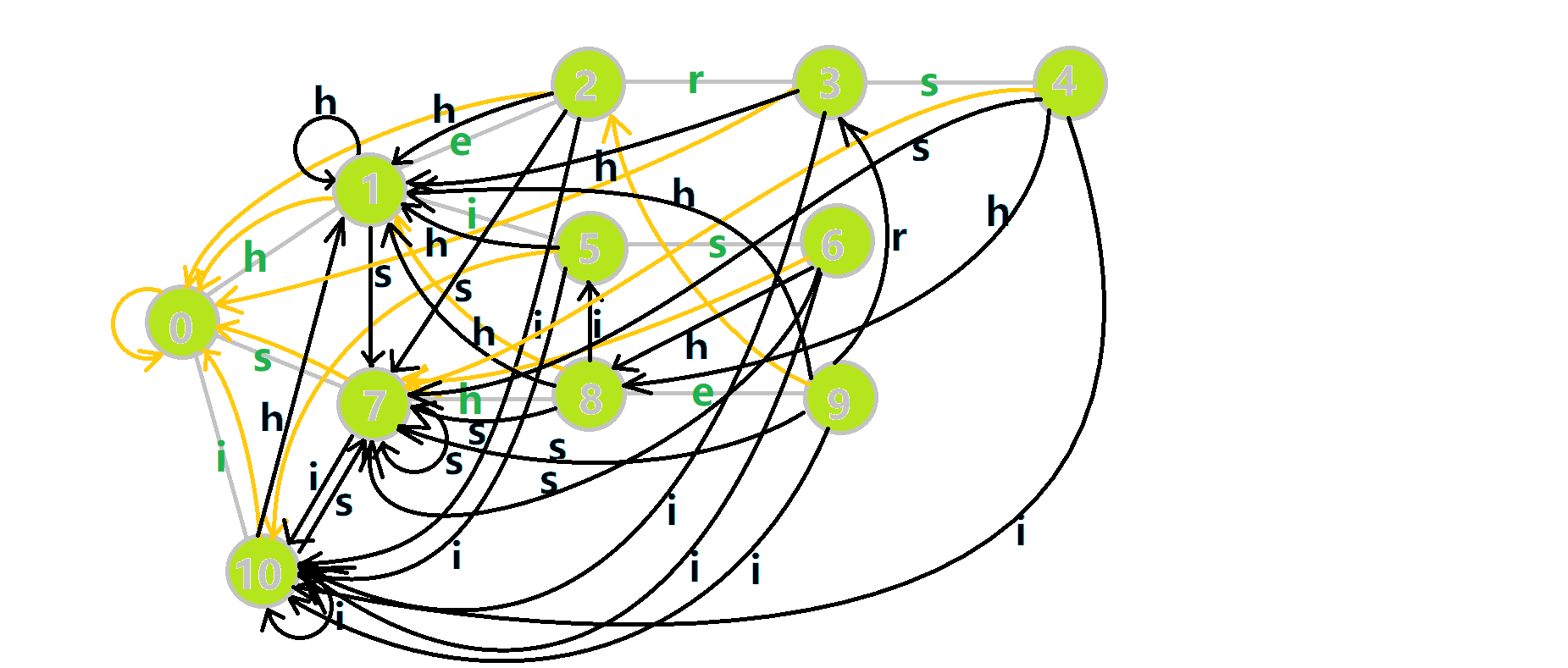
放个OI-Wiki上偷来的例子:
对于模式串 i he his she hers 构建 指针:
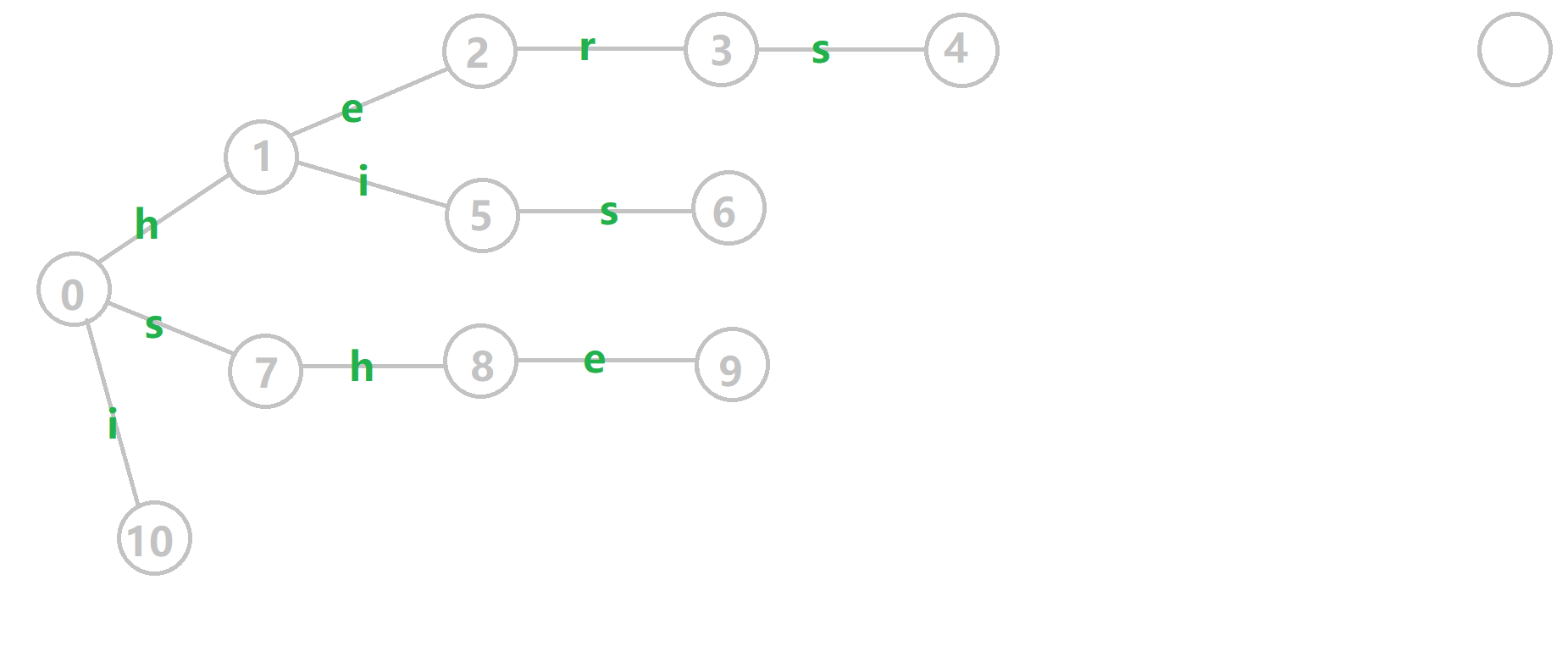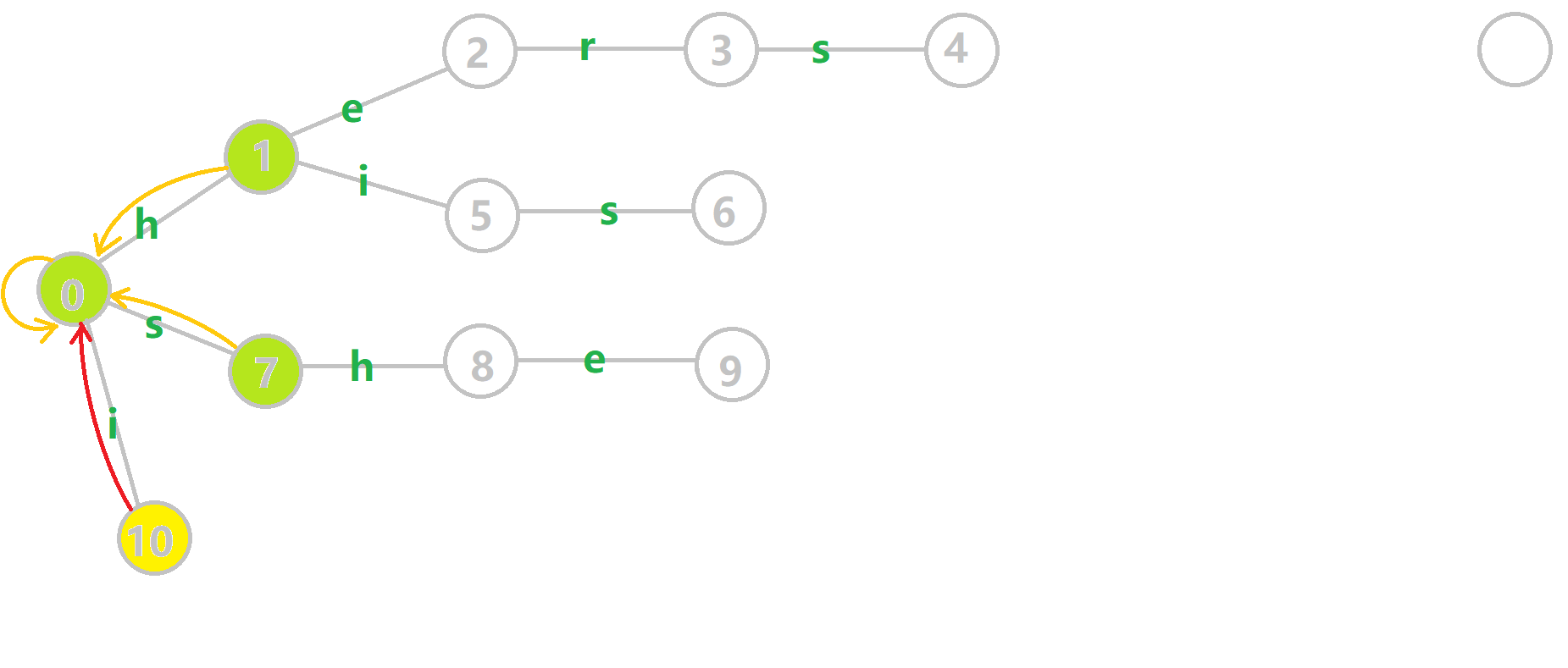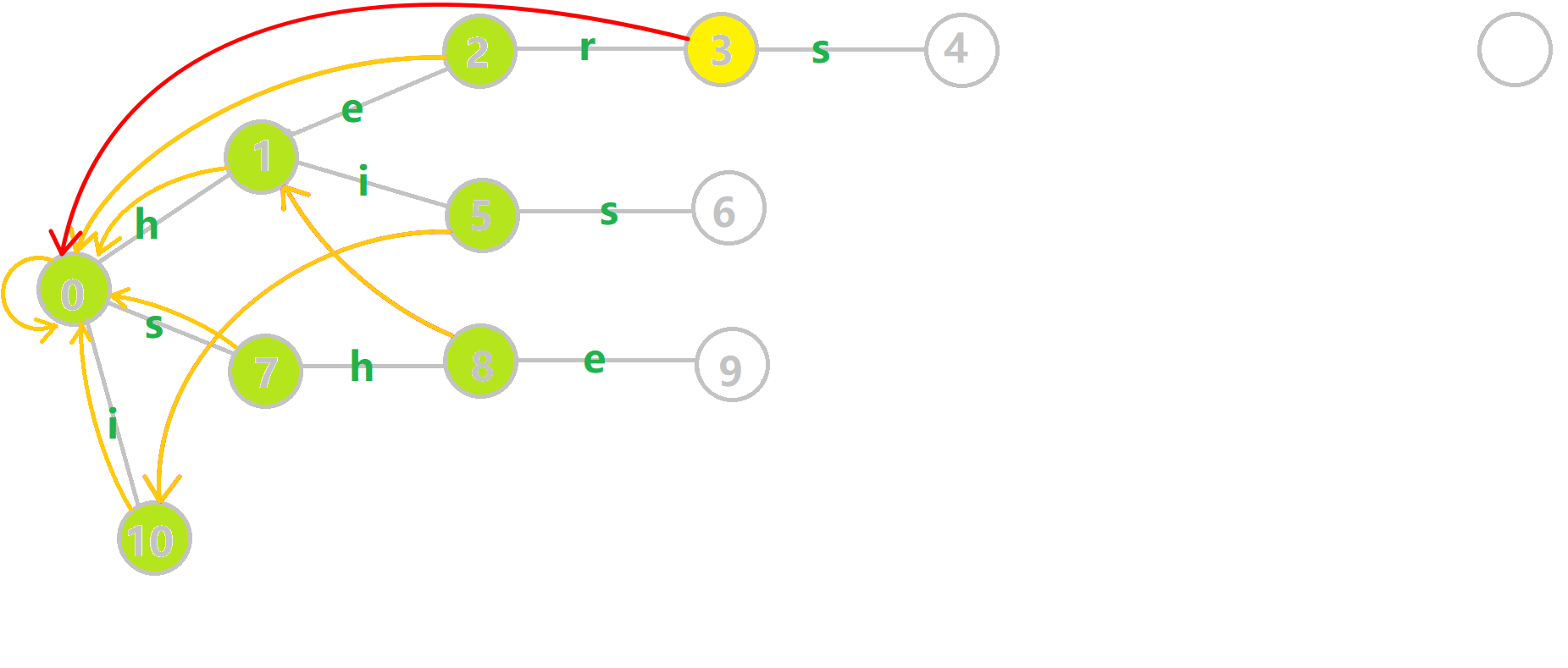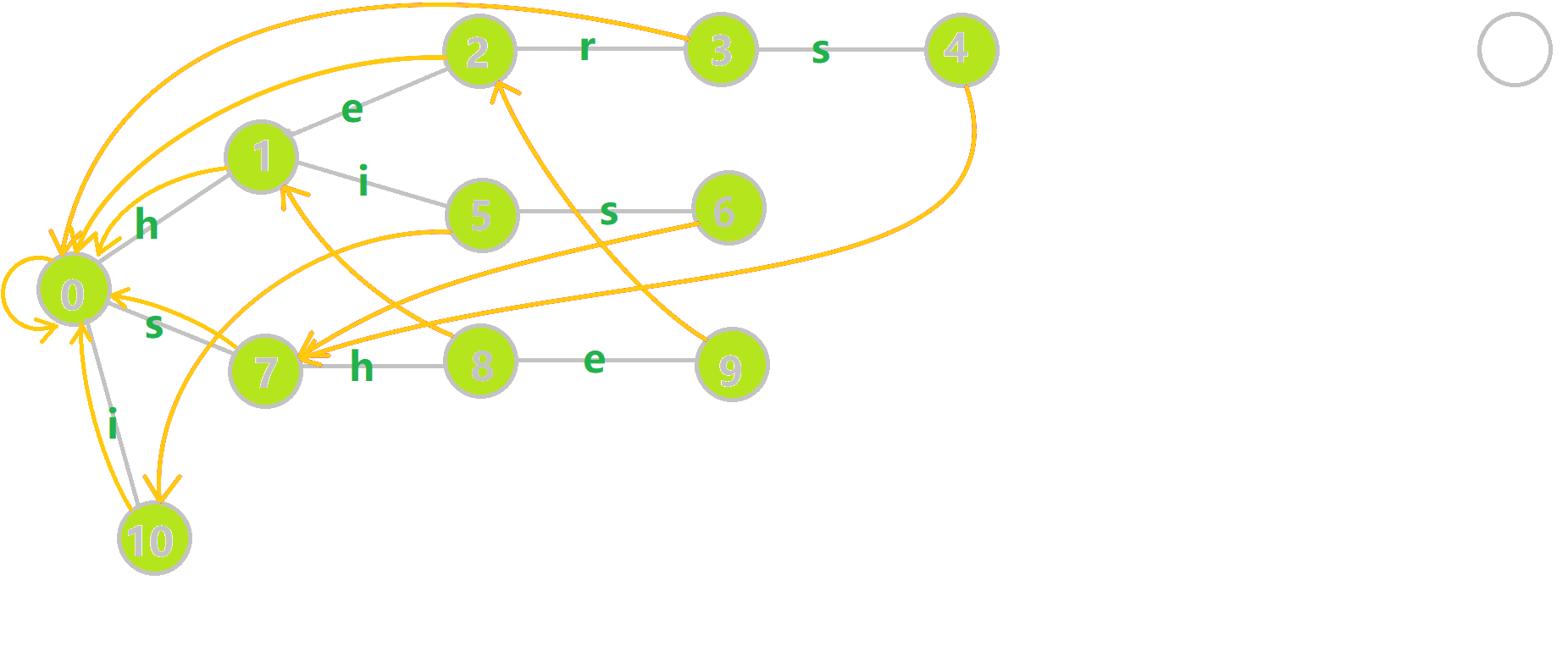
- 黄色结点:当前的结点 。
- 绿色结点:表示已经 BFS 遍历完毕的结点。
- 橙色的边: 指针。
- 红色的边:当前求出的 指针。
我们重点分析结点 的 指针构建:
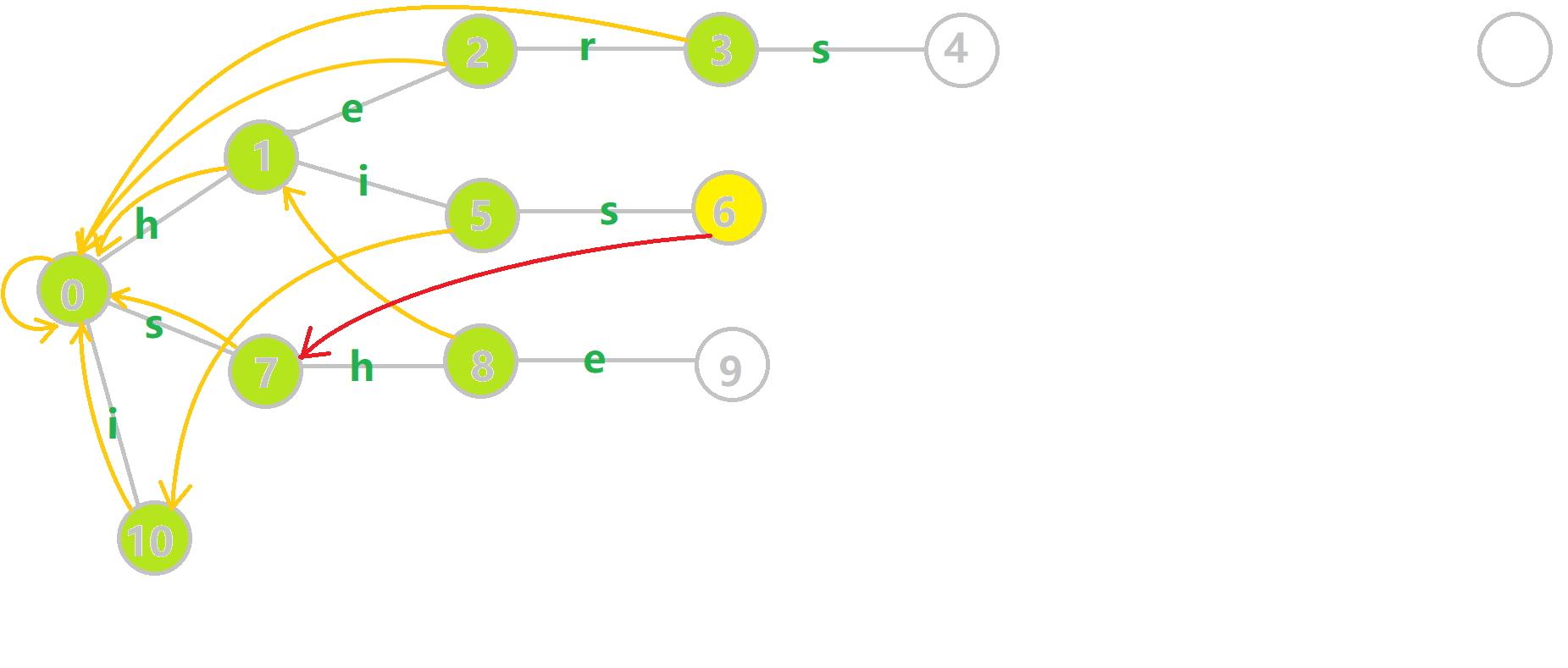
找到 的父结点 ,。然而 结点没有字母 连出的边;继续跳到 的 指针,。发现 结点有字母 连出的边,指向 结点;所以 。最后放一张建出来的图
当然,这只是基本思想,真这么一个个递归找 指针复杂度直接爆炸。
可以想到 指针一定指向深度小于等于它的节点,并且要靠父节点来找自己的 指针,所以考虑 BFS 来实现这个逐层扩展。
实现:
- 预处理出第二层(根节点下第一层) 的 指针,压入队列。
- 广搜,枚举队列中节点的每个子节点。
- 如果子节点存在,子节点的 指针指向父节点的 指针对应的节点的相同子节点,让子节点入队。
- 如果子节点不存在,当前子节点指向父节点的 指针对应的节点的相同子节点。
可能 操作较难理解一些,个人的理解是可以类比并查集的路径压缩,这样如果有其他节点的 指针找到了一个空节点,就不用一下一下的跳 指针了,保证了时间复杂度。
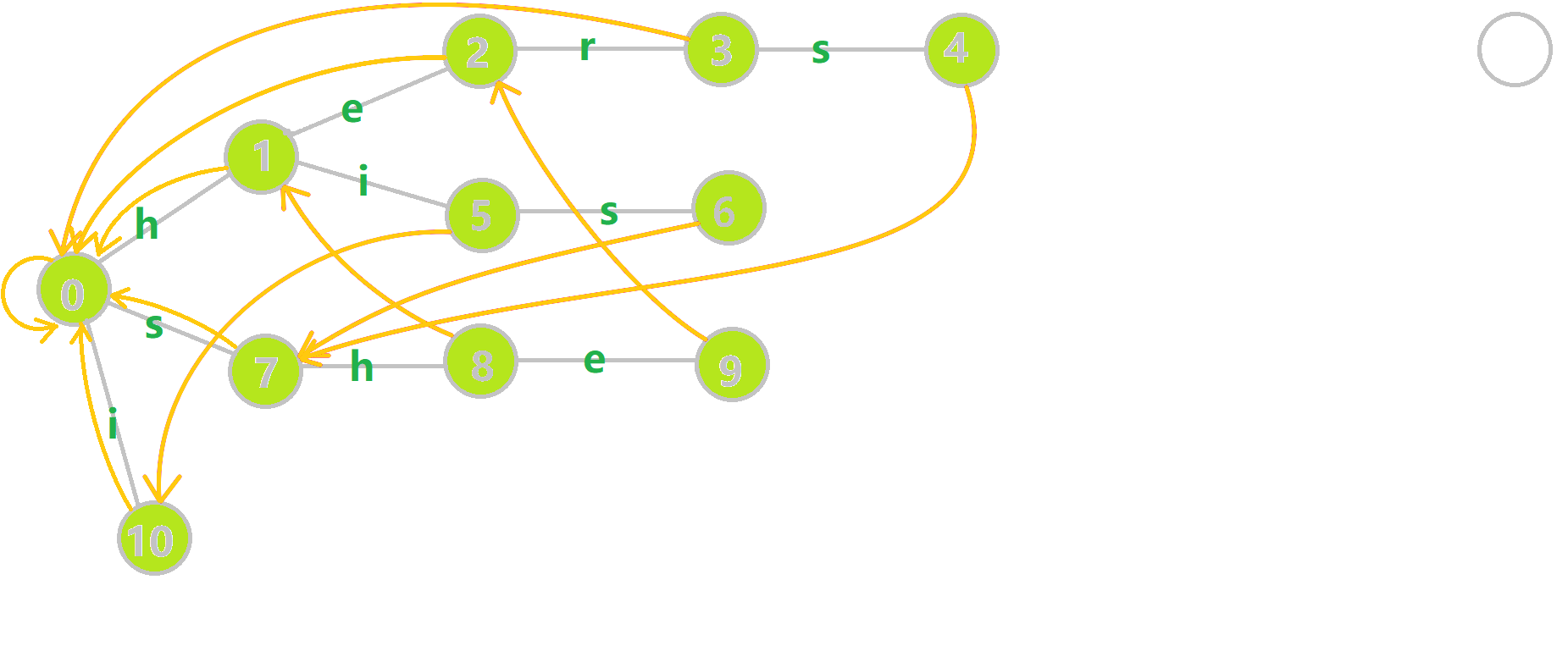
继续偷 OI-Wiki 的图来感受一下:
- 蓝色结点:BFS 遍历到的结点 。
- 蓝色的边:当前结点下,AC自动机修改字典树结构连出的边。
- 黑色的边:AC自动机修改字典树结构连出的边。
- 红色的边:当前结点求出的 指针。
- 黄色的边:fail 指针。
- 灰色的边:字典树的边。
可以发现,众多交错的黑色边将字典树变成了字典图。
Code
void Build(){ queue<int> q; for(register int i = 0; i < SIZE; i++) if(ch[0][i]) q.push(ch[0][i]), fail[ch[0][i]] = 0; //第一层的fail指针指向根节点 while(!q.empty()){ int u = q.front(); q.pop(); for(register int i = 0; i < SIZE; i++){ //枚举每个子节点 if(ch[u][i]){ q.push(ch[u][i]); fail[ch[u][i]] = ch[fail[u]][i]; } else ch[u][i] = ch[fail[u]][i]; } } }
查询
查询就简单一些了,考虑到 指针指向当前状态的最长的后缀,如果当前状态匹配成功的话,它的最长后缀肯定也能匹配成功,所以跳 指针就能找到以当前节点为结尾的模式串的出现次数。
继续用 OI-Wiki 的图来举例(匹配文本串 ushersheishis):
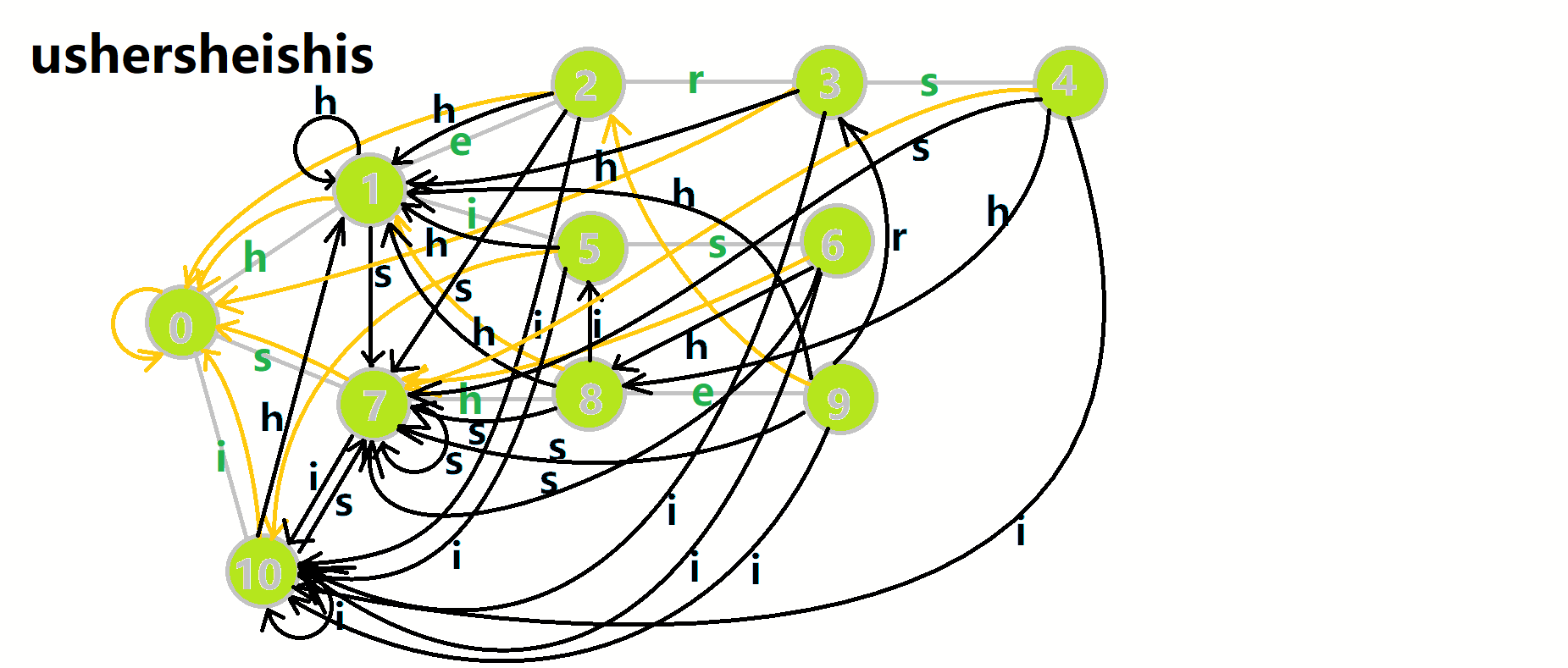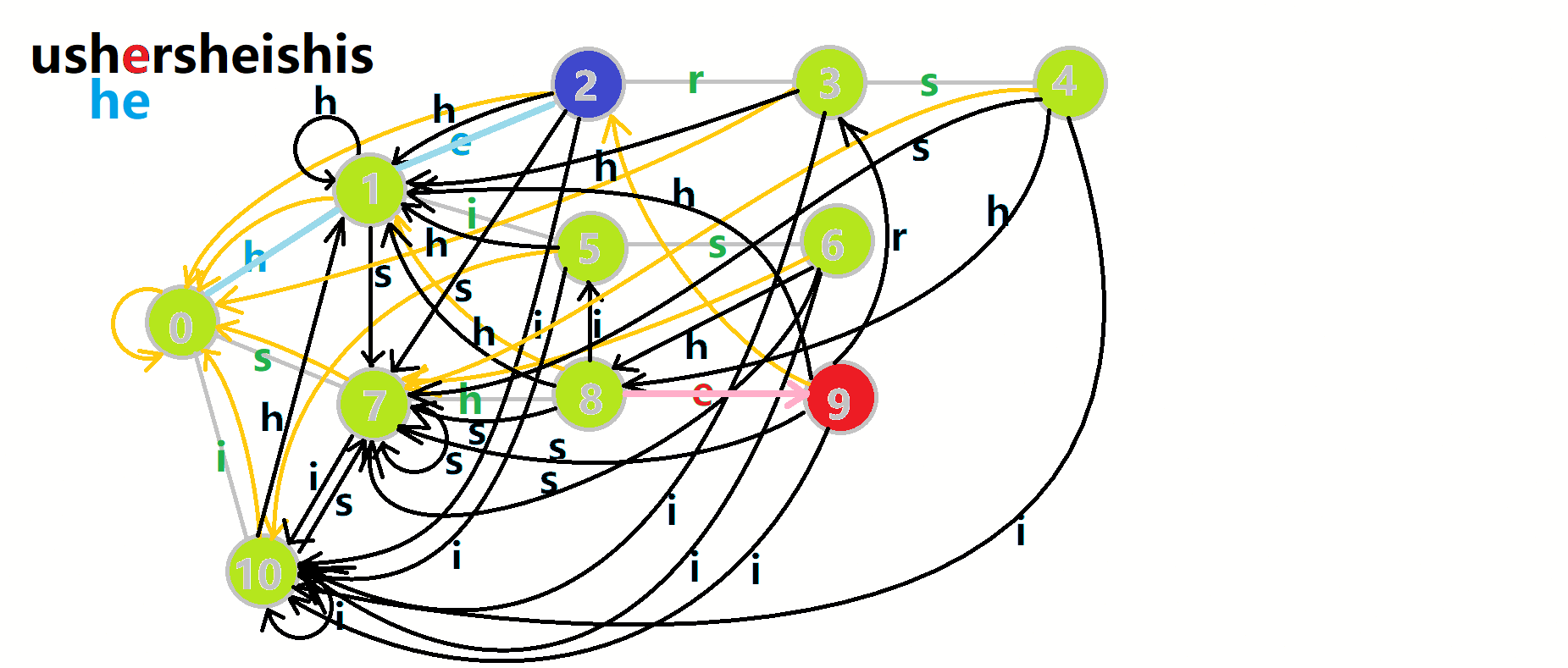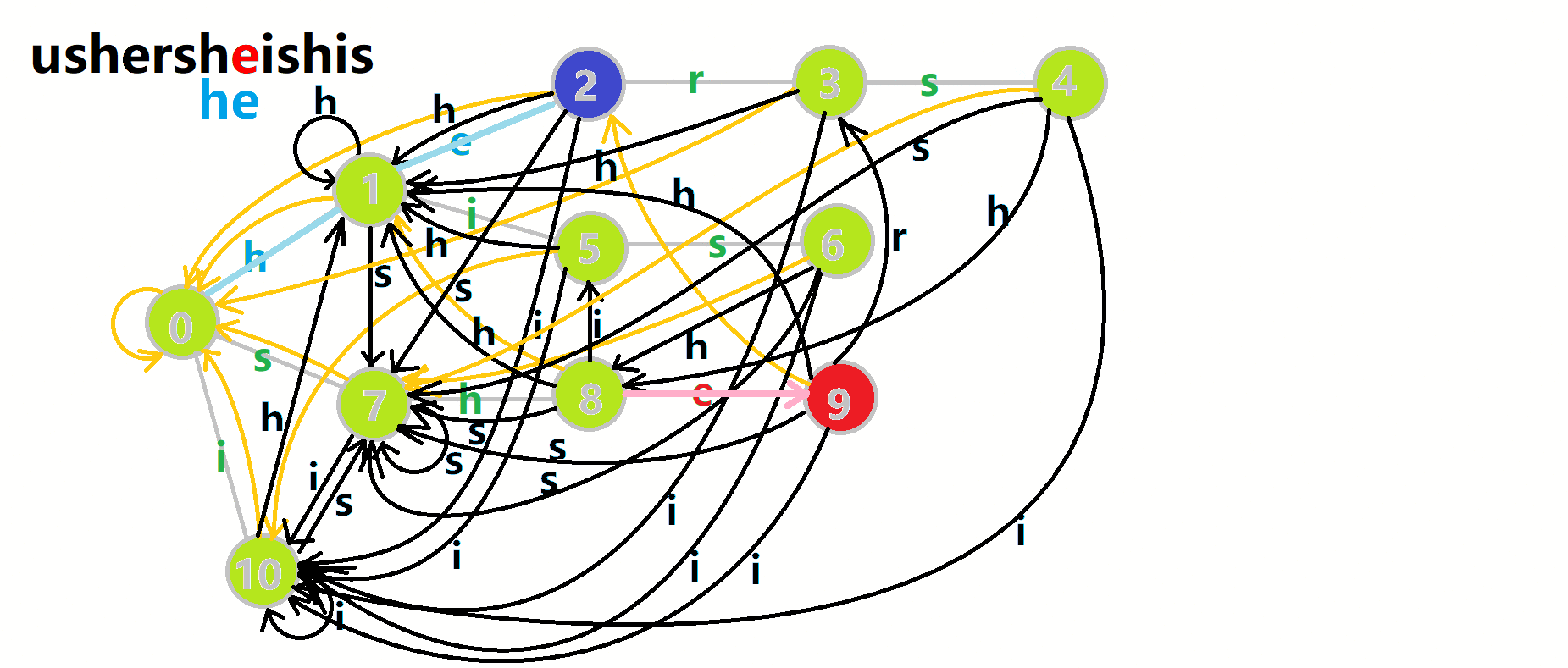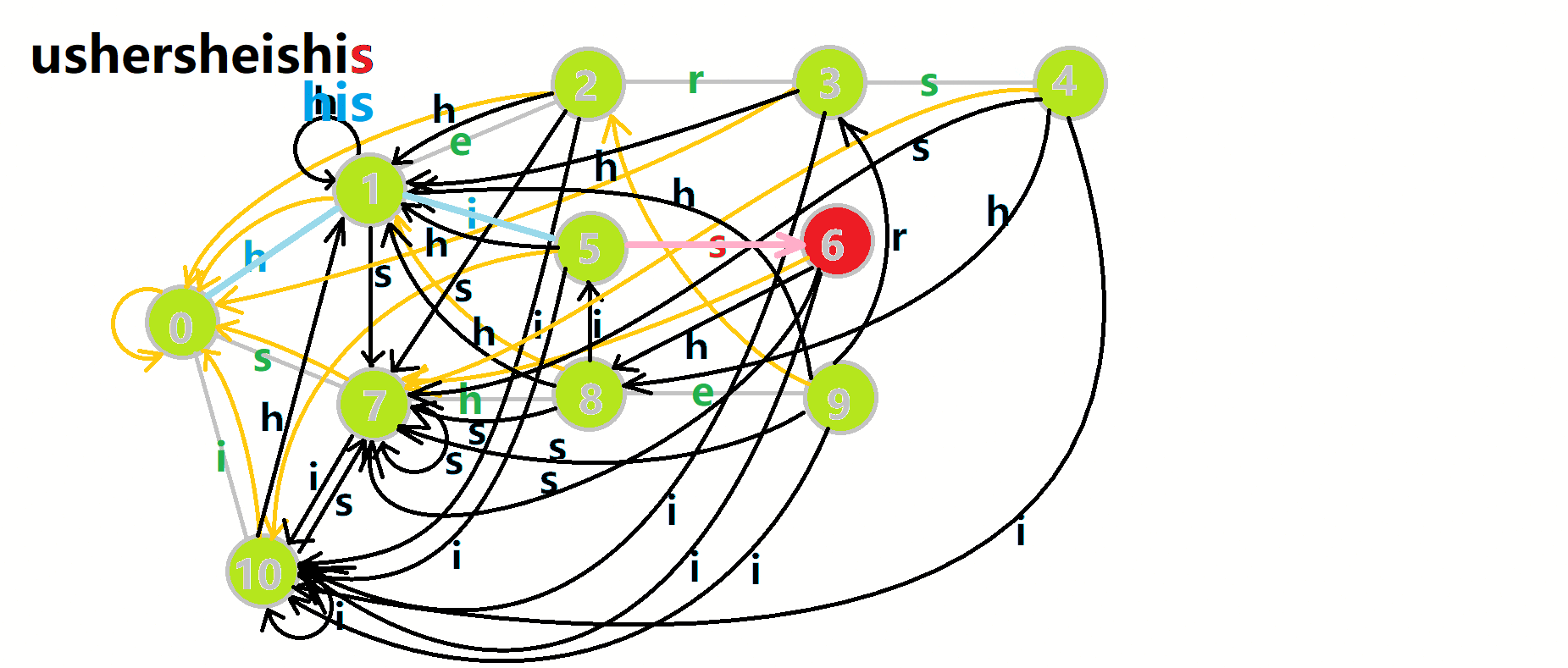
- 红色结点: 结点。
- 粉色箭头: 在自动机上的跳转。
- 蓝色的边:成功匹配的模式串。
- 蓝色结点:示跳 指针时的结点(状态)。
Code
void Query(char *s){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; u = ch[u][v]; for(register int t = u; t; t = fail[t]) ++ans[val[t]]; } }
时间复杂度
设 为模式串的长度, 为文本串的长度, 为字符集的大小,如果连了 trie 图,时间复杂度为 ,其中 为AC自动机中节点的数目。如果不连 trie 图,并且在构建 指针的时候避免遍历到空儿子,时间复杂度就是 。
例题
P3808 【模板】AC 自动机(简单版)
模板题,不过这道题只要求模式串出现过没有,所以每个模式串的结尾只用访问一遍,不然 #1 直接T飞,打个标记就行了。
Code
#include<queue> #include<cstdio> #include<cstring> #include<algorithm> using namespace std; const int MAXN = 1e6 + 10; const int SIZE = 26; int n, tot; int pos[MAXN], ans[MAXN]; char s[MAXN], t[MAXN]; struct Aho_Corasick_Automaton{ int sz; int val[MAXN * 25]; int fail[MAXN * 25]; int ch[MAXN * 25][SIZE]; void Insert(char *s, int id){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; if(!ch[u][v]) ch[u][v] = ++sz; u = ch[u][v]; } if(!val[u]) val[u] = id; pos[id] = val[u]; } void Build(){ queue<int> q; for(register int i = 0; i < SIZE; i++) if(ch[0][i]) q.push(ch[0][i]), fail[ch[0][i]] = 0; while(!q.empty()){ int u = q.front(); q.pop(); for(register int i = 0; i < SIZE; i++){ if(ch[u][i]){ q.push(ch[u][i]); fail[ch[u][i]] = ch[fail[u]][i]; } else ch[u][i] = ch[fail[u]][i]; } } } void Query(char *s){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; u = ch[u][v]; for(register int t = u; t && val[t] != -1; t = fail[t]) ++ans[val[t]], val[t] = -1; } } }A; int main(){ scanf("%d", &n); for(register int i = 1; i <= n; i++){ scanf("%s", s + 1); A.Insert(s, i); } scanf("%s", t + 1); A.Build(), A.Query(t); for(register int i = 1; i <= n; i++) if(ans[pos[i]]) ++tot; printf("%d", tot); return 0; }
P3796 【模板】AC 自动机(加强版)
简简单单的查询,查询出来后给模式串排个序就行了。
Code
#include<queue> #include<cstdio> #include<cstring> #include<algorithm> using namespace std; const int MAXN = 1e6 + 10, MAXM = 155; const int SIZE = 26, LENTH = 75; int n, maxn; char t[MAXN]; char s[MAXM][LENTH]; struct Anser{ int pos, num; }ans[MAXM]; bool cmp(const Anser &a, const Anser &b){ if(a.num != b.num) return a.num > b.num; return a.pos < b.pos; } struct Aho_Corasick_Automaton{ int sz; int val[MAXN]; int fail[MAXN]; int ch[MAXN][SIZE]; void Insert(char *s, int id){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; if(!ch[u][v]) ch[u][v] = ++sz; u = ch[u][v]; } val[u] = id; } void Build(){ queue<int> q; for(register int i = 0; i < SIZE; i++) if(ch[0][i]) q.push(ch[0][i]), fail[ch[0][i]] = 0; while(!q.empty()){ int u = q.front(); q.pop(); for(register int i = 0; i < SIZE; i++){ if(ch[u][i]) q.push(ch[u][i]), fail[ch[u][i]] = ch[fail[u]][i]; else ch[u][i] = ch[fail[u]][i]; } } } void Query(char *s){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; u = ch[u][v]; for(register int t = u; t; t = fail[t]) ++ans[val[t]].num; } } }A; void Clear(){ A.sz = 0; memset(A.ch, 0, sizeof(A.ch)); memset(A.val, 0, sizeof(A.val)); memset(A.fail, 0, sizeof(A.fail)); } int main(){ while(true){ Clear(); scanf("%d", &n); if(n == 0) break; for(register int i = 1; i <= n; i++){ scanf("%s", s[i] + 1); ans[i].pos = i, ans[i].num = 0; A.Insert(s[i], i); } scanf("%s", t + 1); A.Build(); A.Query(t); sort(ans + 1, ans + 1 + n, cmp); maxn = ans[1].num; printf("%d\n", maxn); for(register int i = 1; i <= n; i++){ if(ans[i].num != maxn) break; else puts(s[ans[i].pos] + 1); } } return 0; }
拓扑排序优化
其实暴力跳 指针的最坏时间复杂度是 的。当我们构造出一组数据使得每次跳 指针只使得深度减 ,那每一次跳都要跳深度次,直接T飞。
那怎么才能在统计次数的时候让 trie 上的每个节点都只经过一次?
考虑把 指针看做一条条的有向边,对一个点进行操作,沿着这个点连出去的点也会进行操作,其实就是跳 。
那可不可以给找到的点打一个标记,最后一次性将全部的标记上传来更新路径上的点。答案是肯定的。不难发现这种统计方法和暴力跳 得到的答案一样。然后去考虑用什么方法统计。
明显,打标记后要从深度大的点开始更新,所以使用拓扑排序。同时由于 指针指向的是当前状态的最长后缀,所以整个字典图其实是个 ,可以跑拓扑排序。
由于视 指针为有向边,所以一个点的入度可能很多,但出度最多就是 ,所以不用再另建图了,直接按照 指针跑就行。
实现:
构建 指针的时候我们顺便记录一下入度。
查询的时候只要记录当前节点被文本串经过了几次。
最后再跑一遍拓扑排序就行了。
Code
void Build(){ queue<int> q; for(register int i = 0; i < SIZE; i++) if(ch[0][i]) q.push(ch[0][i]), fail[ch[0][i]] = 0; while(!q.empty()){ int u = q.front(); q.pop(); for(register int i = 0; i < SIZE; i++){ if(ch[u][i]){ q.push(ch[u][i]); fail[ch[u][i]] = ch[fail[u]][i]; ++in[fail[ch[u][i]]]; //记录入度,其余的都相同 } else ch[u][i] = ch[fail[u]][i]; } } } void Query(char *s){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; u = ch[u][v]; ++cnt[u]; //仅仅记录该节点被经过了几次就行 } } void Topsort(){ queue<int> q; for(register int i = 0; i <= sz; i++) if(!in[i]) q.push(i); //没有入度的节点深度一定最深 while(!q.empty()){ int u = q.front(); q.pop(); ans[val[u]] = cnt[u]; //统计答案 int v = fail[u]; --in[v]; cnt[v] += cnt[u]; //累加被经过的次数 if(!in[v]) q.push(v); } }
P5357 【模板】AC 自动机(二次加强版)
粘板子就能过。
Code
#include<queue> #include<cstdio> #include<cstring> #include<algorithm> using namespace std; const int MAXN = 2e5 + 10, MAXM = 2e6 + 10; const int SIZE = 26; int n; int ans[MAXN], pos[MAXN]; char t[MAXN], s[MAXM]; struct Aho_Corasick_Automaton{ int sz; int in[MAXN * 25]; int val[MAXN * 25], cnt[MAXN * 25]; int fail[MAXN * 25]; int ch[MAXN * 25][SIZE]; void Insert(char *s, int id){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; if(!ch[u][v]) ch[u][v] = ++sz; u = ch[u][v]; } if(!val[u]) val[u] = id; pos[id] = val[u]; } void Build(){ queue<int> q; for(register int i = 0; i < SIZE; i++) if(ch[0][i]) q.push(ch[0][i]), fail[ch[0][i]] = 0; while(!q.empty()){ int u = q.front(); q.pop(); for(register int i = 0; i < SIZE; i++){ if(ch[u][i]){ q.push(ch[u][i]); fail[ch[u][i]] = ch[fail[u]][i]; ++in[fail[ch[u][i]]]; } else ch[u][i] = ch[fail[u]][i]; } } } void Query(char *s){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; u = ch[u][v]; ++cnt[u]; } } void Topsort(){ queue<int> q; for(register int i = 0; i <= sz; i++) if(!in[i]) q.push(i); while(!q.empty()){ int u = q.front(); q.pop(); ans[val[u]] = cnt[u]; int v = fail[u]; --in[v]; cnt[v] += cnt[u]; if(!in[v]) q.push(v); } } }A; int main(){ scanf("%d", &n); for(register int i = 1; i <= n; i++){ scanf("%s", t + 1); A.Insert(t, i); } scanf("%s", s + 1); A.Build(), A.Query(s), A.Topsort(); for(register int i = 1; i <= n; i++) printf("%d\n", ans[pos[i]]); return 0; }
P3966 [TJOI2013]单词
也很板,把所有的模式串插进去,每个文本串都在AC自动机里跑一遍,最后再跑一遍拓扑排序统计答案即可。
Code
#include<queue> #include<cstdio> #include<cstring> #include<algorithm> using namespace std; const int MAXN = 210, MAXM = 1e6 + 10; const int SIZE = 26; int n; int ans[MAXN], pos[MAXN]; char s[MAXN][MAXM]; struct Aho_Corasick_Automaton{ int sz; int in[MAXM * 25]; int val[MAXM * 25], cnt[MAXM * 25]; int fail[MAXM * 25]; int ch[MAXM * 25][SIZE]; void Insert(char *s, int id){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; if(!ch[u][v]) ch[u][v] = ++sz; u = ch[u][v]; } if(!val[u]) val[u] = id; pos[id] = val[u]; } void Build(){ queue<int> q; for(register int i = 0; i < SIZE; i++) if(ch[0][i]) q.push(ch[0][i]), fail[ch[0][i]] = 0; while(!q.empty()){ int u = q.front(); q.pop(); for(register int i = 0; i < SIZE; i++){ if(ch[u][i]){ q.push(ch[u][i]); fail[ch[u][i]] = ch[fail[u]][i]; ++in[fail[ch[u][i]]]; } else ch[u][i] = ch[fail[u]][i]; } } } void Query(char *s){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = s[i] - 'a'; u = ch[u][v]; ++cnt[u]; } } void Topsort(){ queue<int> q; for(register int i = 1; i <= sz; i++) if(!in[i]) q.push(i); while(!q.empty()){ int u = q.front(); q.pop(); ans[val[u]] = cnt[u]; int v = fail[u]; --in[v]; cnt[v] += cnt[u]; if(!in[v]) q.push(v); } } }A; int main(){ scanf("%d", &n); for(register int i = 1; i <= n; i++){ scanf("%s", s[i] + 1); A.Insert(s[i], i); } A.Build(); for(register int i = 1; i <= n; i++) A.Query(s[i]); A.Topsort(); for(register int i = 1; i <= n; i++) printf("%d\n", ans[pos[i]]); return 0; }
P5231 [JSOI2012]玄武密码
稍微需要想一下的板子题,我们视文字段为模式串,母串为文本串,建出AC自动机。在匹配文本串时遍历过的节点 代表的状态一定存在于文本串上,所以我们可以给节点 打上标记,再重新遍历每个模式串在AC自动机里的节点,有标记即可更新答案。
Code
#include<queue> #include<cstdio> #include<cstring> #include<algorithm> using namespace std; const int MAXN = 1e7 + 10, MAXM = 1e5 + 10; const int SIZE = 4, LENTH = 110; int n, m; char s[MAXN]; char t[MAXM][LENTH]; int Calc(char c){ if(c == 'E') return 0; if(c == 'S') return 1; if(c == 'W') return 2; if(c == 'N') return 3; } struct Aho_Corasick_Automaton{ int sz; int val[MAXN * 25], cnt[MAXN * 25]; int fail[MAXN * 25]; int ch[MAXN * 25][SIZE]; void Insert(char *s, int id){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = Calc(s[i]); if(!ch[u][v]) ch[u][v] = ++sz; u = ch[u][v]; } val[u] = id; } void Build(){ queue<int> q; for(register int i = 0; i < SIZE; i++) if(ch[0][i]) q.push(ch[0][i]), fail[ch[0][i]] = 0; while(!q.empty()){ int u = q.front(); q.pop(); for(register int i = 0; i < SIZE; i++){ if(ch[u][i]){ q.push(ch[u][i]); fail[ch[u][i]] = ch[fail[u]][i]; } else ch[u][i] = ch[fail[u]][i]; } } } void Query(char *s){ int len = strlen(s + 1), u = 0; for(register int i = 1; i <= len; i++){ int v = Calc(s[i]); u = ch[u][v]; for(register int t = u; t && val[t] != -1; t = fail[t]) cnt[t] = 1, val[t] = -1; } } int Find(char *s){ int len = strlen(s + 1), u = 0, ans = 0; for(register int i = 1; i <= len; i++){ int v = Calc(s[i]); u = ch[u][v]; if(cnt[u]) ans = i; } return ans; } }A; int main(){ scanf("%d%d", &n, &m); scanf("%s", s + 1); for(register int i = 1; i <= m; i++) scanf("%s", t[i] + 1), A.Insert(t[i], i); A.Build(), A.Query(s); for(register int i = 1; i <= m; i++) printf("%d\n", A.Find(t[i])); return 0; }
以下为博客签名,与博文无关。
只要你们不停下来,那前面就一定有我。所以啊,不要停下来~
本文来自博客园,作者:TSTYFST,转载请注明原文链接:https://www.cnblogs.com/TSTYFST/p/16875307.html



【推荐】国内首个AI IDE,深度理解中文开发场景,立即下载体验Trae
【推荐】编程新体验,更懂你的AI,立即体验豆包MarsCode编程助手
【推荐】抖音旗下AI助手豆包,你的智能百科全书,全免费不限次数
【推荐】轻量又高性能的 SSH 工具 IShell:AI 加持,快人一步
· winform 绘制太阳,地球,月球 运作规律
· 震惊!C++程序真的从main开始吗?99%的程序员都答错了
· AI与.NET技术实操系列(五):向量存储与相似性搜索在 .NET 中的实现
· 超详细:普通电脑也行Windows部署deepseek R1训练数据并当服务器共享给他人
· 【硬核科普】Trae如何「偷看」你的代码?零基础破解AI编程运行原理