Forward Algorithm
The goal of the forward algorithm is to compute the joint probability 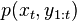 ,
where for notational convenience we have abbreviated
,
where for notational convenience we have abbreviated 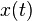 as
as  and
and  as
as  .
Computing
.
Computing 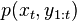 directly would require marginalizing over
all possible state sequences
directly would require marginalizing over
all possible state sequences  ,
the number of which grows exponentially with
,
the number of which grows exponentially with 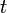 .
Instead, the forward algorithm takes advantage of the conditional independence rules
of the hidden Markov model (HMM) to perform the calculation recursively.
.
Instead, the forward algorithm takes advantage of the conditional independence rules
of the hidden Markov model (HMM) to perform the calculation recursively.
To demonstrate the recursion, let
-
-
 .
.
-
Using the chain rule to expand  ,
we can then write
,
we can then write
-
-
 .
.
-
Because  is conditionally independent of
everything but
is conditionally independent of
everything but  , and
, and  is
conditionally independent of everything but
is
conditionally independent of everything but  ,
this simplifies to
,
this simplifies to
-
-
 .
.
-
Thus, since  and
and  are
given by the model's emission distributions and transition
probabilities, one can quickly calculate
are
given by the model's emission distributions and transition
probabilities, one can quickly calculate  from
from  and
avoid incurring exponential computation time.
and
avoid incurring exponential computation time.
The forward algorithm is easily modified to account for observations from variants of the hidden Markov model as well, such as the Markov jump linear system.



