YBTOJ 5.4树形DP

A.树上求和

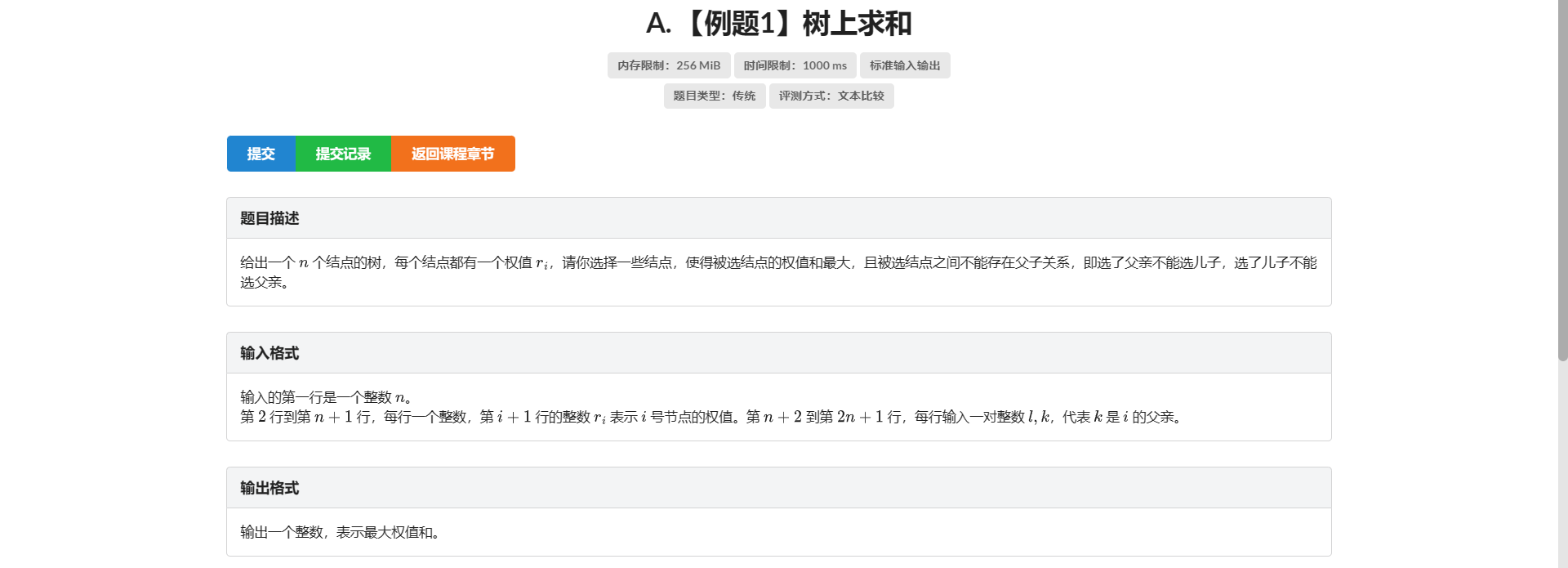
因为它有选/不选的状态我们设状态的时候要考虑进去
所以设 $f [i] [0 / 1]$ 表示第 $i$ 个节点没选/选的最大价值
显然就有:

$f [f a] [0] = \sum m a x (f [s o n] [0], f [s o n] [1])$
$f [f a] [1] = \sum f [s o n] [0]$
因为父亲的状态要从儿子转移过来所以先递归后转移

点击查看代码

#include <bits/stdc++.h>
using namespace std;

const int N = 1e4 + 0721;
int head[N], to[N], nxt[N], cnt;
int f[N][2];
bool rt[N];
int n, root;

inline void cmb(int x, int y) {
	to[++cnt] = y;
	nxt[cnt] = head[x];
	head[x] = cnt;
}

void dfs(int x) {
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		dfs(y);
		f[x][0] += max(f[y][0], f[y][1]);
		f[x][1] += f[y][0];
	}
}

int main() {
	scanf("%d", &n);
	for (int i = 1; i <= n; ++i) scanf("%d", &f[i][1]);
	for (int i = 1; i < n; ++i) {
		int fa, son;
		scanf("%d%d", &son, &fa);
		cmb(fa, son);
		rt[son] = 1;
	}
	
	for (int i = 1; i <= n; ++i) {
		if (!rt[i]) {
			root = i;
			break;
		}
	}
	
	dfs(root);
	
	printf("%d", max(f[root][0], f[root][1]));
	
	return 0;
}

B.节点覆盖

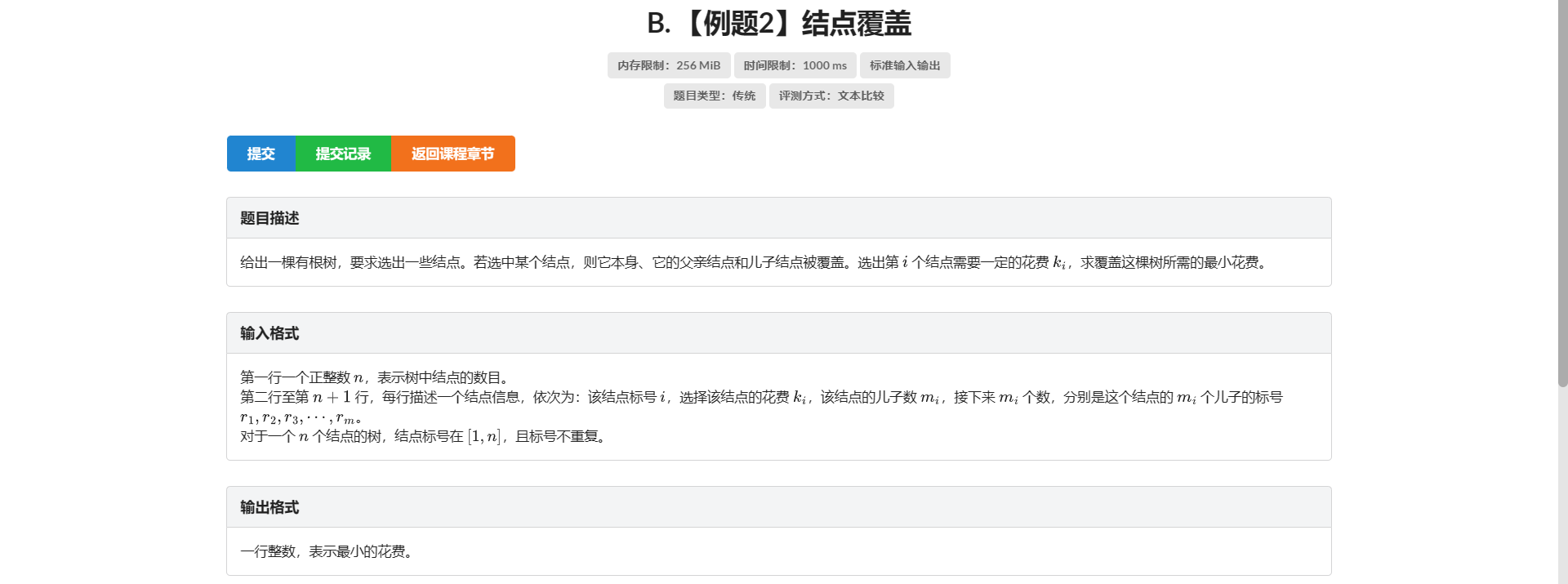
很容易想到的一个思路是用 $1 / 0$ 表示选/不选这个节点
但是有一个问题转移的时候它的父亲和它的儿子必须满足至少选一个没法转移
所以我们考虑设 $0 / 1 / 2$ 表示被父亲/自己/儿子看守
转移很显然可以看代码

点击查看代码

#include <bits/stdc++.h>
using namespace std;

const int N = 0x0d00;
int head[N], nxt[N], to[N], v[N];
int dp[N][3];
int cnt, n, ans;

void cmb(int x, int y) {
    to[++cnt] = y;
    nxt[cnt] = head[x];
    head[x] = cnt;
}

void dfs(int x, int fa) {
    dp[x][1] = v[x];
    int tmp = 0x7ffffff;
    for (int i = head[x]; i; i = nxt[i]) {
        int y = to[i];
        if (y == fa)
            continue;
        dfs(y, x);
        tmp = min(tmp, dp[y][1] - dp[y][2]);
        dp[x][0] += min(dp[y][1], dp[y][2]);
        dp[x][1] += min(dp[y][0], min(dp[y][1], dp[y][2]));
        dp[x][2] += min(dp[y][1], dp[y][2]);
    }
    dp[x][2] += max(tmp, 0);
    if (fa == 0)
        ans = min(dp[x][1], dp[x][2]);
}

int main() {
    scanf("%d", &n);
    for (int i = 1; i <= n; ++i) {
        int x, m;
        cin >> x;
        cin >> v[x];
        cin >> m;
        if (m != 0) {
            for (int j = 1; j <= m; ++j) {
                int u;
                scanf("%d", &u);
                cmb(x, u);
                cmb(u, x);
            }
        }
    }
    //	cout<<cnt ;
    dfs(1, 0);

    //	for( int i = 1 ; i <= cnt ; ++i )
    //	cout<<to[i]<<" " ;

    printf("%d", ans);

    return 0;
}

C.最长距离

详见YBTOJ 5.4例3 最长距离题解

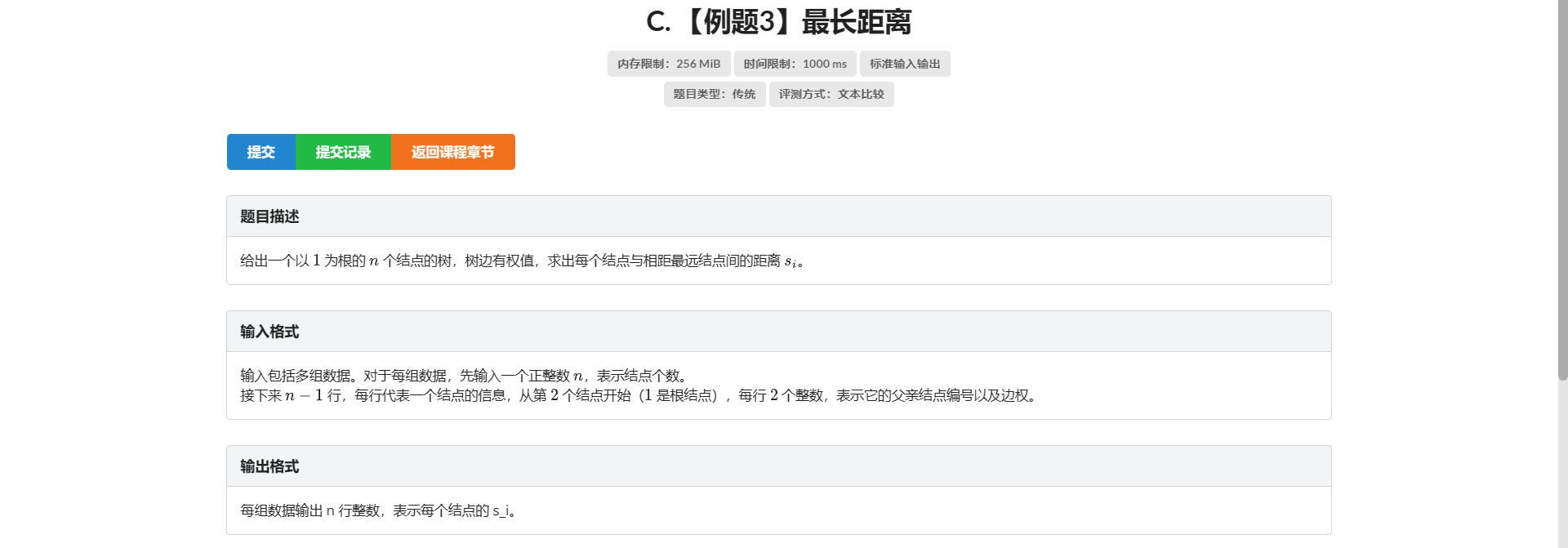
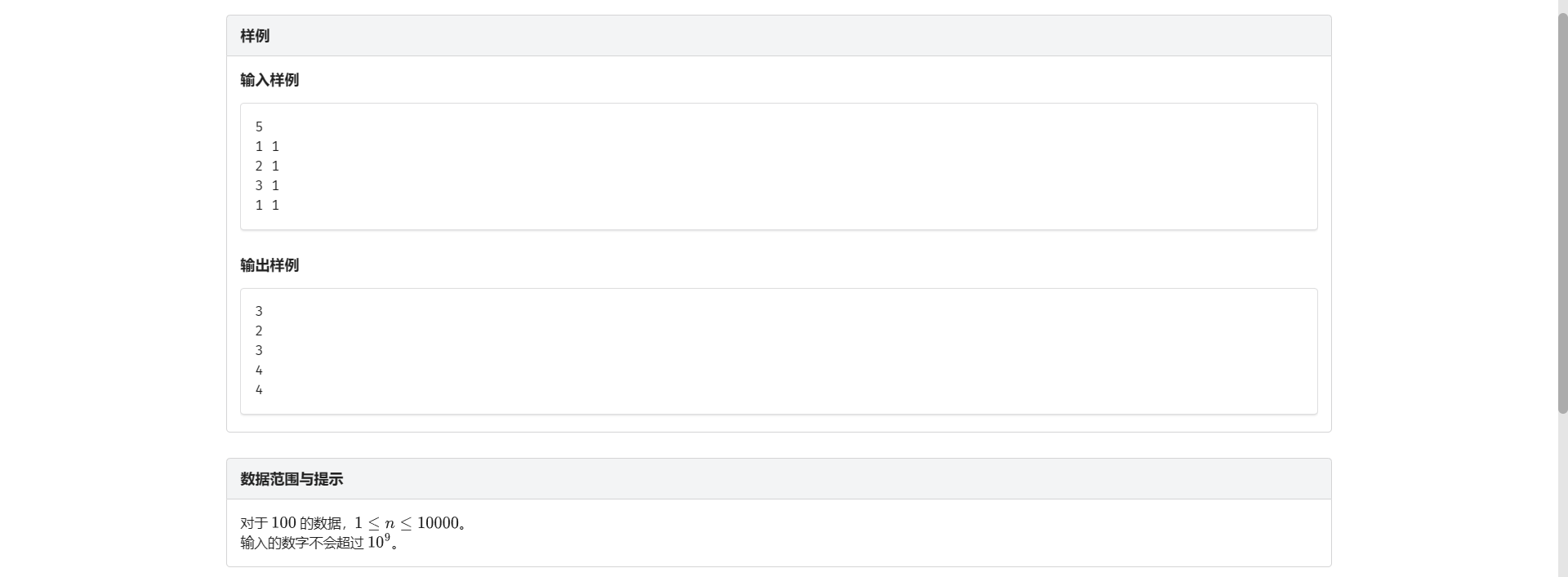
D.选课方案

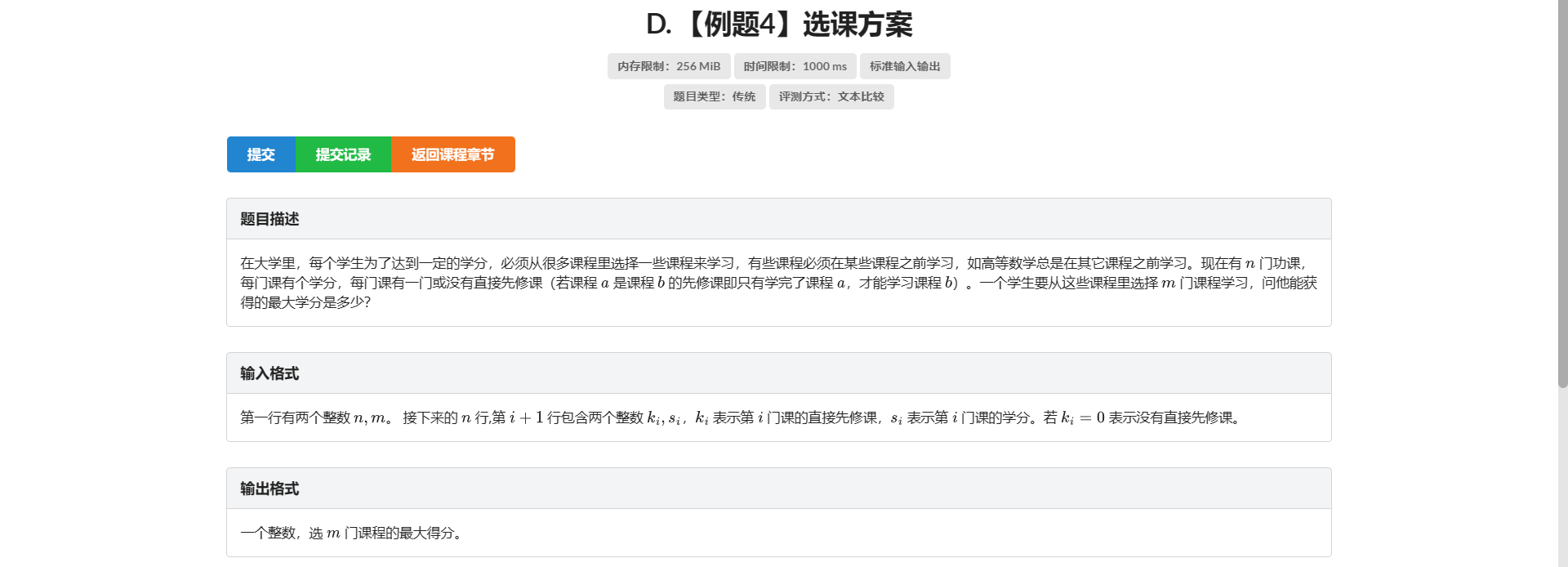
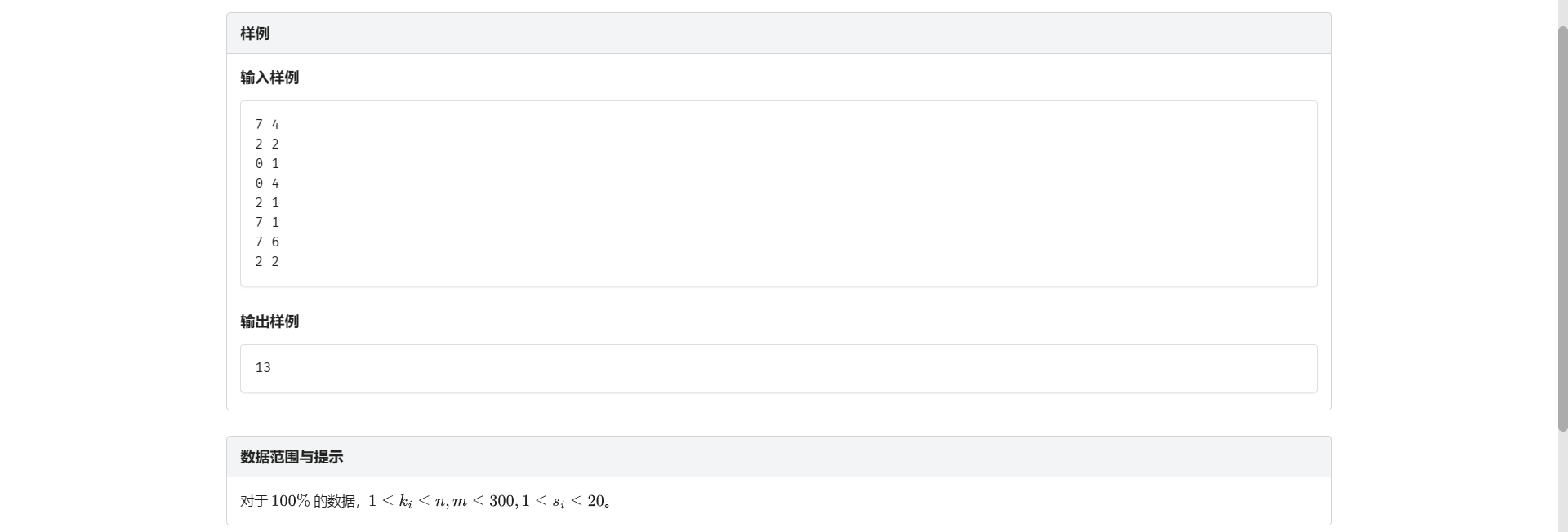
详见P2014 选课（树上背包）
复杂度证明是假的不要看

点击查看代码

#include <bits/stdc++.h>
using namespace std;

const int N = 1e3 + 0721;
int head[N], nxt[N], to[N], cnt;
int dp[N][N];
int m, n;

void cmb(int x, int y) {
    to[++cnt] = y;
    nxt[cnt] = head[x];
    head[x] = cnt;
}

void dfs(int x) {
    for (int i = head[x]; i; i = nxt[i]) {
        int y = to[i];
        dfs(y);
        for (int j = m + 1; j > 0; --j) {
            for (int k = 0; k < j; ++k) dp[x][j] = max(dp[x][j], dp[x][j - k] + dp[y][k]);
        }
    }
}

int main() {
    scanf("%d%d", &n, &m);
    for (int i = 1; i <= n; ++i) {
        int x, y;
        scanf("%d%d", &x, &y);
        cmb(x, i);
        for (int j = 1; j <= m + 1; ++j) dp[i][j] = y;
    }

    dfs(0);

    printf("%d", dp[0][m + 1]);

    return 0;
}

E.路径求和

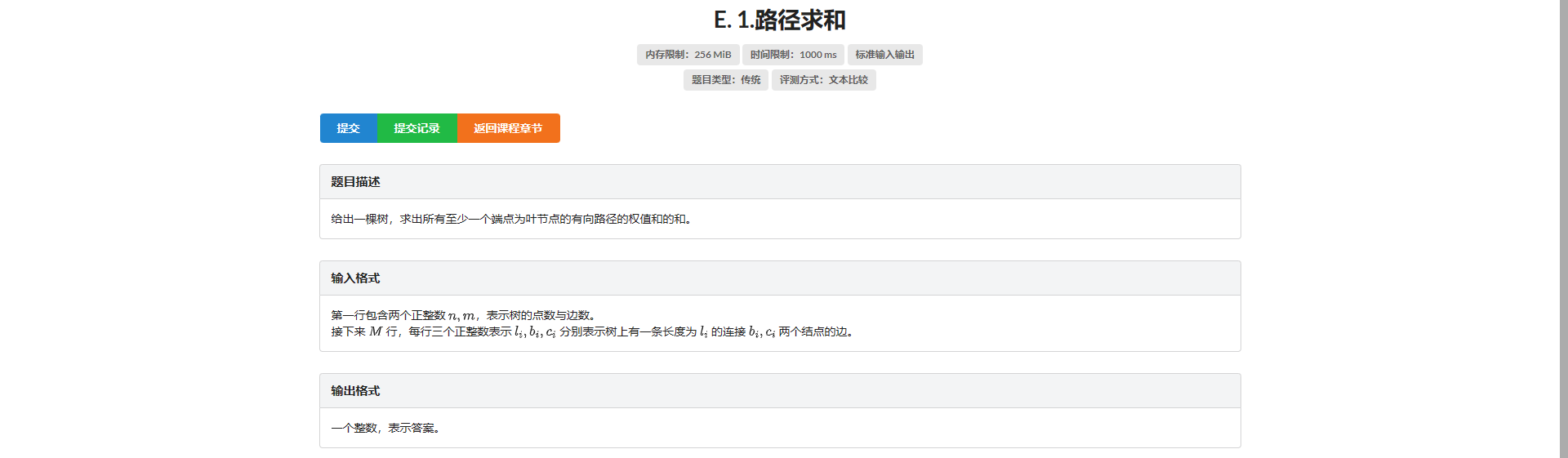
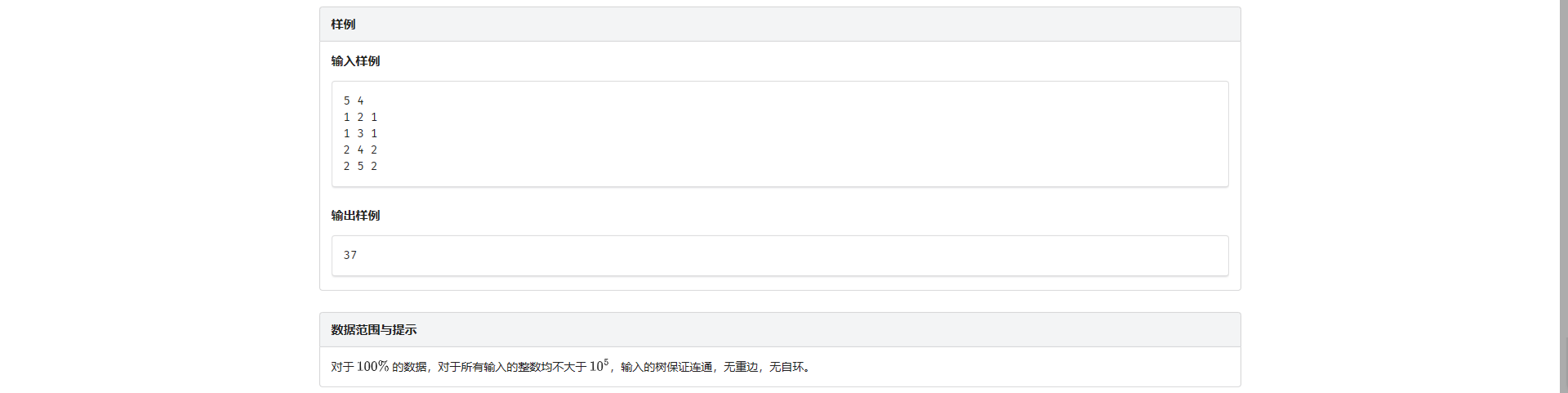
很适合独立思考的一道题但是题面没看懂直接去找题解了可惜了

应该加上一句话：对于无根树我们定义度数为 $1$ 的点为叶节点

暴力的想法是枚举点对 $O (n^{2})$

考虑每一条边对答案的贡献把它断掉把树分为两个子树 $x, y$
那么这条边对答案的贡献就是 $x$ 中的所有叶节点走到 $y$ 中的所有节点 + $y$ 中的所有叶节点走到 $x$ 中的所有节点
所以我们直接维护出每个子树的大小以及叶节点数量即可

理论上要把 $d e g_{i} \neq 1$ 的 $i$ 作根节点开始 dfs
但实际这题直接 dfs(1, 0) 也能过

注意本题是先输入边权再输入两端点 /fn

点击查看代码

#include <bits/stdc++.h>
#define ll long long 
using namespace std;

namespace steven24 {
	
const int N = 1e5 + 0721;
int head[N], to[N << 1], nxt[N << 1], len[N << 1], cnt;
int deg[N], siz[N], num[N];
int totleaf;
int n, m;
ll ans;

inline int read() {
    int xr = 0, F = 1; 
	char cr;
    while (cr = getchar(), cr < '0' || cr > '9') if (cr == '-') F = -1;
    while (cr >= '0' && cr <= '9') 
        xr = (xr << 3) + (xr << 1) + (cr ^ 48), cr = getchar();
    return xr * F;
}

inline void add_edge(int x, int y, int z) {
	to[++cnt] = y;
	nxt[cnt] = head[x];
	head[x] = cnt;
	len[cnt] = z;
}

void dfs(int x, int fa) {
	siz[x] = 1;
	if (deg[x] == 1) {
		++totleaf;
		num[x] = 1;
	}
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		if (y == fa) continue;
		dfs(y, x);
		siz[x] += siz[y];
		num[x] += num[y];
	}
}

void get_ans(int x, int fa) {
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		if (y == fa) continue;
		ans += 1ll * len[i] * (1ll * num[y] * (n - siz[y]) + 1ll * siz[y] * (totleaf - num[y]));
		get_ans(y, x);
	}
}

void main() {
	n = read(), m = read();
//	cerr << n << " " << m << "\n";
	for (int i = 1; i <= m; ++i) {
		int x, y, z;
		z = read(), x = read(), y = read();
//		cerr << x << " " << y << " " << z << "\n";
		add_edge(x, y, z);
		add_edge(y, x, z);
		++deg[x], ++deg[y];
	}
	for (int i = 1; i <= n; ++i) {
		if (deg[i] > 1) {
			dfs(i, 0);
			get_ans(i, 0);
			break;
		}
	}
	printf("%lld\n", ans);
}
	
}

int main() {
	steven24::main();
	return 0;
}
/*
5 4
1 2 1
1 3 1
2 4 2
2 5 2
*/

F.树上移动

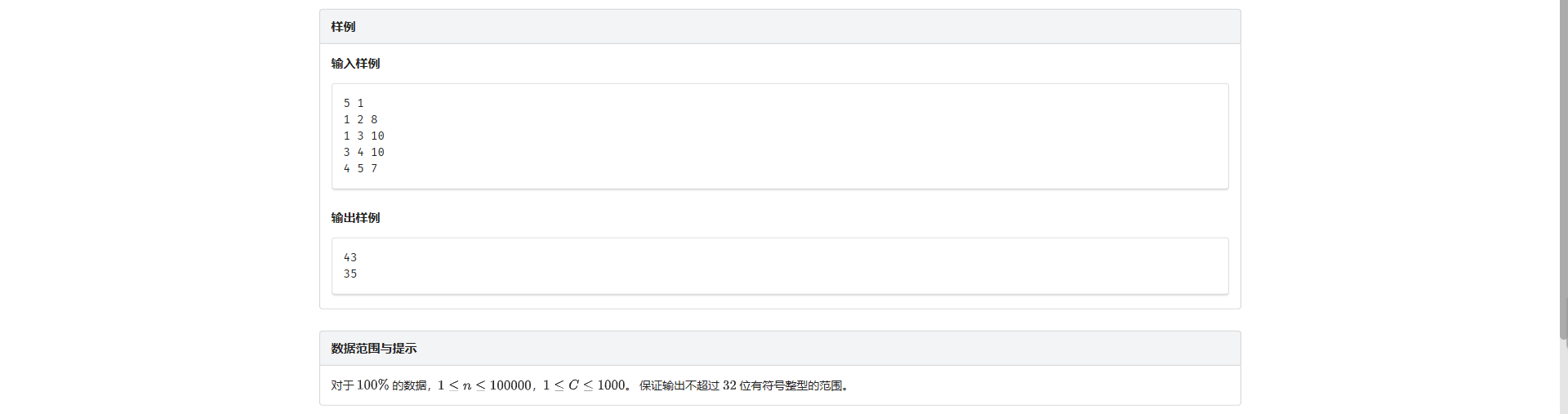
无根树并且 $S$ 是固定的不难想到把 $S$ 作为根节点

设 $f_{i, 0 / 1 / 2}$ 表示从 $i$ 出发遍历子树内所有节点回到 / 一个点出发不回到 / 两个点出发不回到该节点的最短距离
转移的时候对于 $f_{x, 0}$ 有 $f_{x, 0} = \sum_{y \in s o n_{x}} f_{y, 0} + 2 \times d i s_{x, y}$
对于 $f_{x, 1}$ 有 $f_{x, 1} = min_{y \in s o n_{x}} (f_{y, 1} + d i s_{x, y} + \sum_{z \in s o n_{x}, z \neq y} f_{z, 0} + 2 \times d i s_{x, z})$

对于第二个转移我们把 $\sum_{z \in s o n_{x}, z \neq y} f_{z, 0} + 2 \times d i s_{x, z}$ 转化成 $f_{x, 0} - f_{y, 0} - d i s_{x, y} \times 2$ 来保证复杂度

对于 $f_{x, 2}$ 可以是一个儿子的 $f_{y, 2} + 2 \times d i s_{x, y}$ + 剩余儿子的 $f_{y, 0} + 2 \times d i s_{x, y}$
也可以是两个儿子的 $f_{y, 1}$ + 剩余儿子的 $f_{y, 0} + 2 \times d i s_{x, y}$
前面那个转移还是用上面那个优化来保证复杂度
对于下面那个我们考虑取到 $f_{y, 1}$ 与 $f_{x, 0}$ 的差值那就是 $f_{x, 0} - f_{y, 0} + f_{y, 1} - d i s_{x, y}$
那么我们直接维护 $- f_{y, 0} + f_{y, 1} - d i s_{x, y}$ 的最小值和次小值即可

那么对于第一问答案就是 $f_{s, 1}$
对于第二问答案就是 $f_{s, 2}$

点击查看代码

#include <bits/stdc++.h>
#define ll long long 
using namespace std;

namespace steven24 {
	
const int N = 1e5 + 0721;
const ll inf = 0x7ffffffffffffff;
int head[N], to[N << 1], nxt[N << 1], len[N << 1], cnt;
ll f[N][3];
int n, s;

inline int read() {
    int xr = 0, F = 1; 
	char cr;
    while (cr = getchar(), cr < '0' || cr > '9') if (cr == '-') F = -1;
    while (cr >= '0' && cr <= '9') 
        xr = (xr << 3) + (xr << 1) + (cr ^ 48), cr = getchar();
    return xr * F;
}

inline void add_edge(int x, int y, int z) {
	to[++cnt] = y;
	nxt[cnt] = head[x];
	head[x] = cnt;
	len[cnt] = z;
}

void dfs(int x, int fa) {
	bool isleaf = 1;
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		if (y == fa) continue;
		isleaf = 0;
		dfs(y, x);
		f[x][0] += f[y][0] + 2 * len[i];
	}
	
	if (!isleaf) {
		f[x][1] = inf;
		f[x][2] = inf;
	}
	ll min1 = inf, min2 = inf;
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		if (y == fa) continue; 
		f[x][1] = min(f[x][1], f[y][1] - len[i] + f[x][0] - f[y][0]);
		f[x][2] = min(f[x][2], f[y][2] + f[x][0] - f[y][0]);
		
		if (f[y][1] - f[y][0] - len[i] < min1) {
			min2 = min(min2, min1);
			min1 = f[y][1] - f[y][0] - len[i];
		} else min2 = min(min2, f[y][1] - f[y][0] - len[i]);
	}
	if (min1 != inf && min2 != inf) f[x][2] = min(f[x][2], f[x][0] + min1 + min2);
	
} 

void main() {
	n = read(), s = read();
	for (int i = 1; i < n; ++i) {
		int x, y, z;
		x = read(), y = read(), z = read();
		add_edge(x, y, z);
		add_edge(y, x, z);
	}
	dfs(s, 0);
	printf("%lld\n", f[s][1]);
	printf("%lld\n", f[s][2]);
}
	
}

int main() {
	steven24::main();
	return 0;
}
/*
5 1
1 2 8
1 3 10
3 4 10
4 5 7
*/

G.块的计数

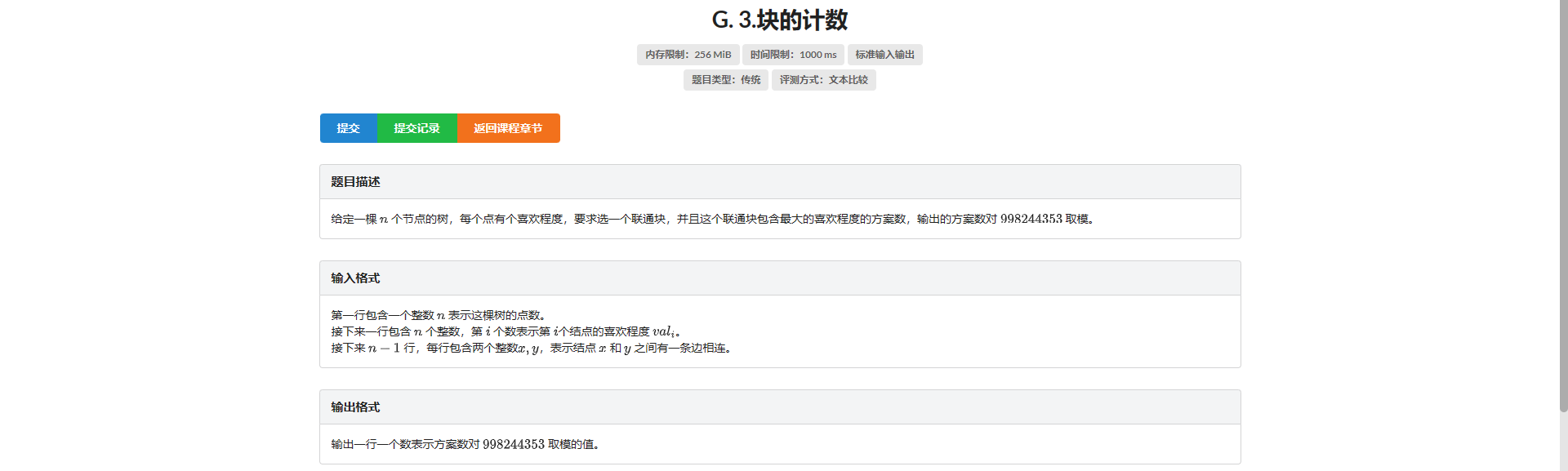
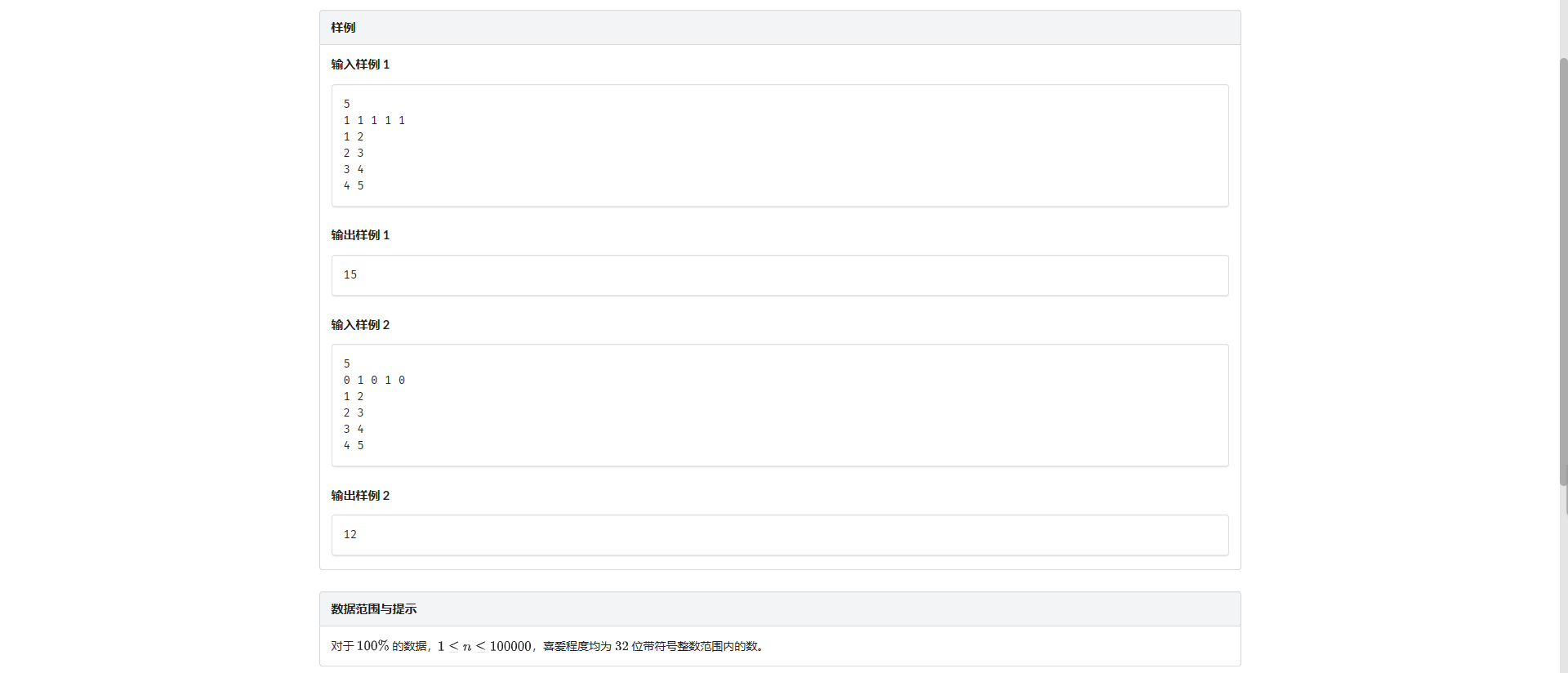
设 $f_{i, 0 / 1}$ 表示以 $i$ 为根节点的合法 / 不合法联通块个数
下意识觉得不合法联通块个数不是很好转移
进而考虑设 $f_{i, 0 / 1}$ 表示以 $i$ 为根节点的合法 / 合不合法都行的联通块个数剩下那个减一下就行了
然后写假了寄。

实际上不好转移的是合法连通块个数
所以设 $f_{i, 0 / 1}$ 表示以 $i$ 为根节点的不合法 / 合不合法都行的连通块个数
那么就有 $f_{x, 0} = \prod_{y \in s o n_{x}} (f_{y, 0} + 1)$ （可以不选）
$f_{x, 1} = \prod_{y \in s o n_{x}} (f_{y, 1} + 1)$ （可以不选）
答案就是 $\sum_{i = 1}^{n} (f_{i, 1} - f_{i, 0})$

点击查看代码

#include <bits/stdc++.h>
#define ll long long

using namespace std;

namespace steven24 {
	
const int N = 1e5 + 0721;
const int mod = 998244353;

ll f[N][2];
int val[N], maxn;
int head[N], nxt[N << 1], to[N << 1], cnt;
int n;	

inline int read() {
    int xr = 0, F = 1; 
	char cr;
    while (cr = getchar(), cr < '0' || cr > '9') if (cr == '-') F = -1;
    while (cr >= '0' && cr <= '9') 
        xr = (xr << 3) + (xr << 1) + (cr ^ 48), cr = getchar();
    return xr * F;
}

inline void add_edge(int x, int y) {
	to[++cnt] = y;
	nxt[cnt] = head[x];
	head[x] = cnt;
}
void dfs(int x, int fa) {
	f[x][1] = 1;
	if (val[x] != maxn) f[x][0] = 1;
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		if (y == fa) continue;
		dfs(y, x);
		f[x][1] = f[x][1] * (f[y][1] + 1) % mod;
		f[x][0] = f[x][0] * (f[y][0] + 1) % mod;
	}
}

void main() {
	n = read();
	for (int i = 1; i <= n; ++i) val[i] = read();
	maxn = *max_element(val + 1, val + 1 + n);
	for (int i = 1; i < n; ++i) {
		int x, y;
		x = read(), y = read();
		add_edge(x, y);
		add_edge(y, x);
	}
	dfs(1, 0);
	ll ans = 0;
	for (int i = 1; i <= n; ++i) ans = (mod + ans + f[i][1] - f[i][0]) % mod;

	printf("%lld\n", ans);
}
	
}

int main() {
	steven24::main();
	return 0;
}
/*
5
1 1 1 1 1
1 2
2 3
3 4
4 5
*/

H.树的合并

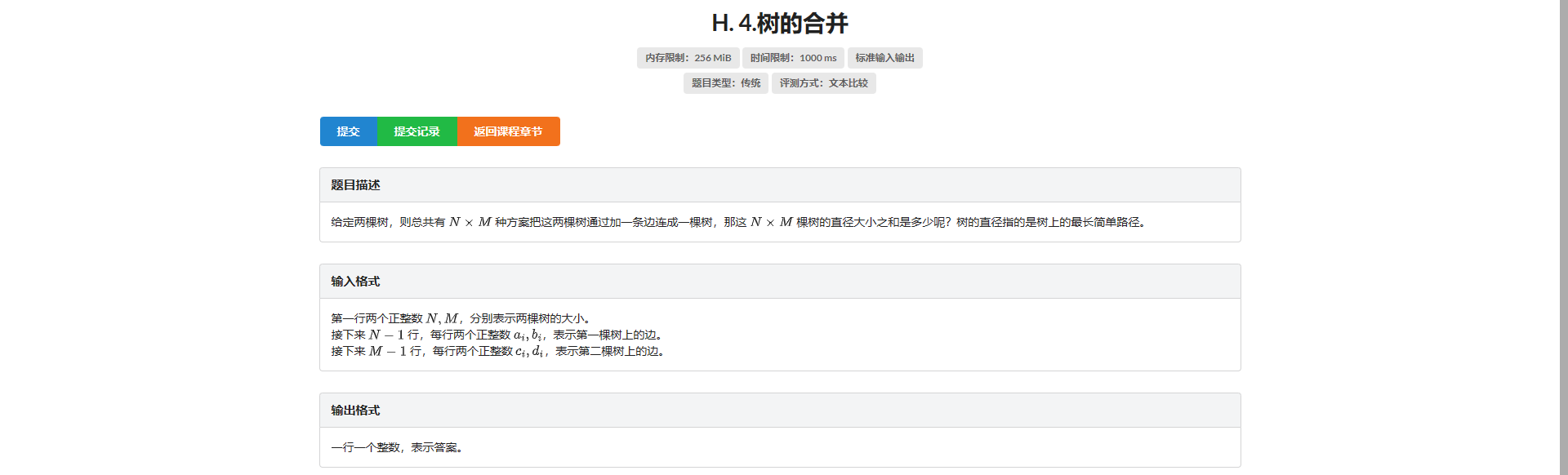
考虑枚举第一颗树上的点把它们和第二颗树上所有点连边的情况
设两点为 $u, v$ 设 $f_{i}$ 表示 $i$ 在它所在树里能走到的最远点距离
那么显然新树直径就是 $max (第一棵树直径, 第二棵树直径, f_{u} + f_{v} + 1)$

我们枚举 $u$ 点那么我们就需要讨论 $f_{u} + f_{v} + 1$ 与 $max (第一棵树直径, 第二棵树直径)$ 的大小
$f_{u}$ 是给定的那么如果我们把 $f_{v}$ 排序我们就可以二分一个位置使它前面的 $v$ 都取 $max (第一棵树直径, 第二棵树直径)$ 后面的 $v$ 都取 $f_{u} + f_{v} + 1$

那么前面那些直接就是点数 $\times max (第一棵树直径, 第二棵树直径)$
后面那些预处理一个后缀和即可

总复杂度 $O (n \log n + n \log m + m \log m)$

如果用 C 题那个做法来预处理 $f$ 数组的话就是 $O (n \log m + m \log m)$
然后如果 $m > n$ 的话就把两棵树 swap 一下就可以做到极致复杂度虽然最开始那个复杂度就随便过了（

注意二分边界为 $[1, m + 1]$ 炸了一发

点击查看代码

/*
两次dfs求出直径两端点
维护树上距离
求出每个点的最长距离 然后全拿下来放到两个数组里
在b中二分找f[i] + g[j] + 1>max(d1, d2)的最小j 
给g弄个后缀和
ans+=max(d1,d2)*(j-1)+h[j]+(f[i]+1)*(n2-j+1) 
*/
#include <bits/stdc++.h>
#define ll long long

using namespace std;

inline int read() {
	int xr = 0, F = 1;
	char cr;
	while (cr = getchar(), cr < '0' || cr > '9') if (cr == '-') F = -1;
	while (cr >= '0' && cr <= '9')
		xr = (xr << 3) + (xr << 1) + (cr ^ 48), cr = getchar();
	return xr * F;
}

void write(ll x) {
	char ws[51];
	int wt = 0;
	if (x < 0) putchar('-'), x = -x;
	do {
		ws[++wt] = x % 10 + '0';
		x /= 10;
	} while (x);
	for (int i = wt; i; --i) putchar(ws[i]);
}

namespace steven24 {

const int N = 1e5 + 0721;
int f[N], g[N];
ll h[N], ans;
int d;

struct tree {

int dis[N], dep[N], fa[21][N];
int head[N], nxt[N << 1], to[N << 1], cnt;
int u1, u2, d;
int n;	

inline void add_edge(int x, int y) {
	to[++cnt] = y;
	nxt[cnt] = head[x];
	head[x] = cnt;
}

void dfs1(int x, int f) {
	dep[x] = dep[f] + 1;
	fa[0][x] = f;
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		if (y == f) continue;
		dfs1(y, x);
	}
}

void dfs2(int x, int f, bool opt) {
	dis[x] = dis[f] + 1;
	if (!opt) {
		if (dis[x] > dis[u1]) u1 = x;
	}
	else {
		if (dis[x] > dis[u2]) u2 = x;
	}
	
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		if (y == f) continue;
		dfs2(y, x, opt);
	}
}

void init() {
	for (int j = 1; j <= 20; ++j) {
		for (int i = 1; i <= n; ++i) fa[j][i] = fa[j - 1][fa[j - 1][i]];
	}
}

int lca(int x, int y) {
	if (dep[x] < dep[y]) swap(x, y);
	for (int j = 20; j >= 0; --j) if (dep[fa[j][x]] >= dep[y]) x = fa[j][x];
	if (x == y) return x;
	for (int j = 20; j >= 0; --j) if (fa[j][x] != fa[j][y]) x = fa[j][x], y = fa[j][y];
	return fa[0][x];
}

int query(int x, int y) {
	return dep[x] + dep[y] - (dep[lca(x, y)] << 1);
}
	
} tr1, tr2;


void init(tree &x) {
	x.dfs1(1, 0);
	x.init();
	x.dfs2(1, 0, 0);
	memset(x.dis, 0, sizeof x.dis);
	x.dfs2(x.u1, 0, 1);
	x.d = x.query(x.u1, x.u2);
}

int binary_search(int val, int l, int r) {
	int mid, ret = -1;
	while (l <= r) {
		mid = (l + r) >> 1;
		if (g[mid] + val + 1 > d) {
			ret = mid;
			r = mid - 1;
		} else 
			l = mid + 1;
	}
	if (ret == -1) return r + 1;
	else return ret;
}

void main() {
	tr1.n = read(), tr2.n = read();
	int n1 = tr1.n, n2 = tr2.n;
	for (int i = 1; i < n1; ++i) {
		int x = read(), y = read();
		tr1.add_edge(x, y);
		tr1.add_edge(y, x); 
	}
	for (int i = 1; i < n2; ++i) {
		int x = read(), y = read();
		tr2.add_edge(x, y);
		tr2.add_edge(y, x);
	}
	init(tr1);
	init(tr2);
	for (int i = 1; i <= n1; ++i) f[i] = max(tr1.query(i, tr1.u1), tr1.query(i, tr1.u2));
	for (int i = 1; i <= n2; ++i) g[i] = max(tr2.query(i, tr2.u1), tr2.query(i, tr2.u2));
	sort(g + 1, g + 1 + n2);
	for (int i = n2; i; --i) h[i] = h[i + 1] + g[i];
	
	d = max(tr1.d, tr2.d);
	for (int i = 1; i <= n1; ++i) {
		int loc = binary_search(f[i], 1, n2);
		ans += 1ll * d * (loc - 1) + h[loc] + 1ll * (f[i] + 1) * (n2 - loc + 1);
	}
	write(ans), putchar('\n');
}

}

int main() {
	steven24::main();
	return 0;
}
/*
4 3
1 2
2 3
2 4
1 3
2 3
*/

I.权值统计

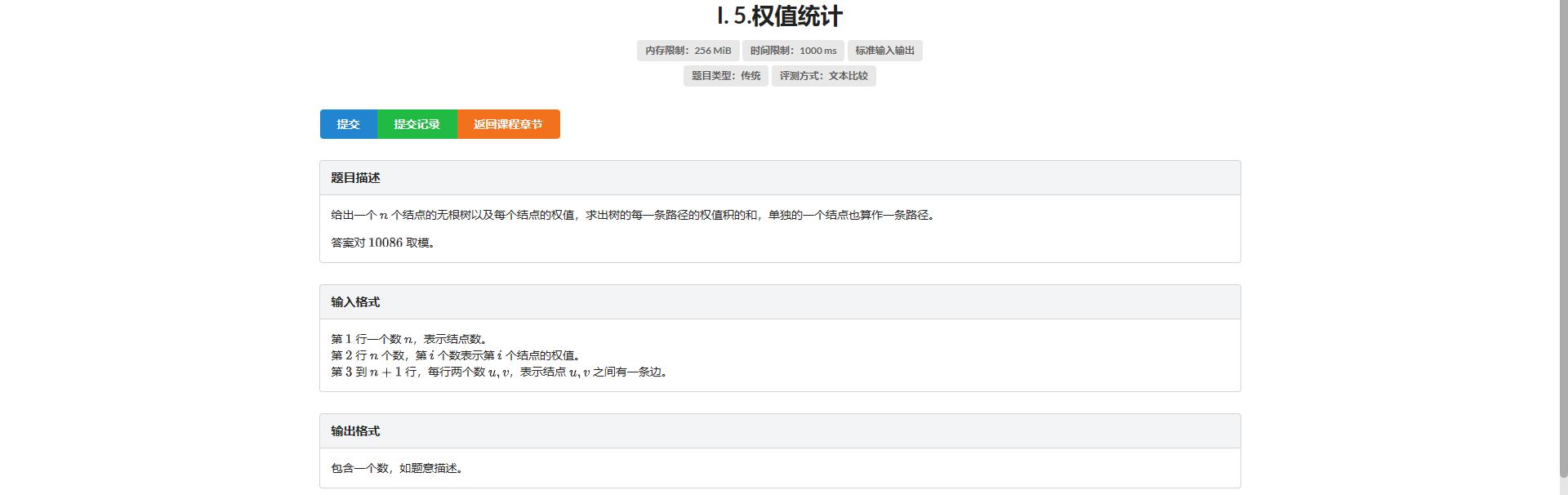
想到个换根的做法结果被卡模数怎么会是呢

设 $f_{i}$ 表示以 $i$ 为根的子树内所有以 $i$ 为终点的路径权值和
设 $g_{i}$ 表示以 $i$ 为根的子树内所有经过 $i$ 的路径权值和

考虑转移
对于 $f_{i}$ 显然就是所有以它儿子为终点的路径都加上它自己还有只包含它自己一个点的路径
有 $f_{i} = (\sum f_{s o n_{i}} + 1) \times v_{i}$

对于 $g_{i}$ 需要额外统计从一个子树出来经过 $i$ 再进入另一个子树的路径权值和
实际上这部分就是 $(\sum 儿子的 f 值两两相乘) \times v_{i}$ 具体可以自己拆一下
注意到 $2 (x_{1} x_{2} + x_{2} x_{3} + x_{1} x_{3}) = (x_{1} + x_{2} + x_{3})^{2} - (x_{1}^{2} + x_{2}^{2} + x_{3}^{2})$ 并且这个柿子对于更多的 $x_{i}$ 也成立
所以就可以换成 $\frac{(\sum f_{s o n_{i}})^{2} - \sum f_{s o n_{i}}^{2}}{2}$
那么就有 $g_{i} = f_{i} + \frac{(\sum f_{s o n_{i}})^{2} - \sum f_{s o n_{i}}^{2}}{2} \times v_{i}$

最终答案为 $\sum_{i = 1}^{n} g_{i}$

点击查看代码

/*
*/
#include <bits/stdc++.h>
#define ll long long


using namespace std;

inline int read() {
	int xr = 0, F = 1;
	char cr;
	while (cr = getchar(), cr < '0' || cr > '9') if (cr == '-') F = -1;
	while (cr >= '0' && cr <= '9')
		xr = (xr << 3) + (xr << 1) + (cr ^ 48), cr = getchar();
	return xr * F;
}

void write(ll x) {
	char ws[51];
	int wt = 0;
	if (x < 0) putchar('-'), x = -x;
	do {
		ws[++wt] = x % 10 + '0';
		x /= 10;
	} while (x);
	for (int i = wt; i; --i) putchar(ws[i]);
}

namespace steven24 {

const int N = 1e5 + 0721;
const int mod = 10086;	
ll f[N];
int v[N];
int head[N], nxt[N << 1], to[N << 1], cnt;
int n;
ll ans;

inline void add_edge(int x, int y) {
	to[++cnt] = y;
	nxt[cnt] = head[x];
	head[x] = cnt;
}

void dfs(int x, int fa) {
	ll sum = 0, tot = 0;
	for (int i = head[x]; i; i = nxt[i]) {
		int y = to[i];
		if (y == fa) continue;
		dfs(y, x);
		sum += f[y];
		tot += f[y] * f[y];
	}
	f[x] = 1ll * (sum + 1) * v[x] % mod;
	ans = (ans + f[x] + (sum * sum - tot) / 2 * v[x]) % mod;
}

void main() {
	n = read();
	for (int i = 1; i <= n; ++i) v[i] = read();
	for (int i = 1; i < n; ++i) {
		int x = read(), y = read();
		add_edge(x, y);
		add_edge(y, x);
	}
	dfs(1, 0);
	write(ans), putchar('\n');
}

}

int main() {
	steven24::main();
	return 0;
}
/*
4 3
1 2
2 3
2 4
1 3
2 3
*/

posted @ 2023-07-02 21:52 Steven24 阅读(70) 评论(0) 编辑收藏举报

刷新页面返回顶部

登录后才能查看或发表评论，立即登录或者逛逛博客园首页

相关博文：

· YBTOJ 5.4例3 最长距离题解

· YBTOJ 4.5LCA问题

· ybtoj树形DP习题

· dls的树形dp

· 动态规划·树形DP

阅读排行：
· 地球OL攻略 —— 某应届生求职总结
· 周边上新：园子的第一款马克杯温暖上架
· Open-Sora 2.0 重磅开源！
· 提示词工程——AI应用必不可少的技术
· .NET周刊【3月第1期 2025-03-02】

公告

昵称： Steven24
园龄： 2年2个月
粉丝： 22
关注： 23

+加关注

2025年3月

日

一

二

三

四

五

六

Steven24

YBTOJ 5.4树形DP

A.树上求和

B.节点覆盖

C.最长距离

D.选课方案

E.路径求和

F.树上移动

G.块的计数

H.树的合并

I.权值统计

公告

搜索

常用链接

随笔分类

随笔档案

阅读排行榜

评论排行榜

推荐排行榜

最新评论

一言（ヒトコト）