HDU100题简要题解(2030~2039)
HDU2030 汉字统计
Problem Description
统计给定文本文件中汉字的个数。
Input
输入文件首先包含一个整数n,表示测试实例的个数,然后是n段文本。
Output
对于每一段文本,输出其中的汉字的个数,每个测试实例的输出占一行。
[Hint:]从汉字机内码的特点考虑~
Sample Input
2
WaHaHa! WaHaHa! 今年过节不说话要说只说普通话WaHaHa! WaHaHa!
马上就要期末考试了Are you ready?
Sample Output
14
9
给的这个hint就很友好哇,百度得知:特点为汉字是由两个负数组成的。那就很好说了
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int n;
char a[1001];
int main() {
while (scanf("%d", &n) != EOF) {
getchar();
while (n--) {
int cnt = 0;
gets(a);
int len = strlen(a);
for (int i = 0; i < len; i++)
if (a[i] < 0) cnt++;
cout << cnt / 2 << endl;
}
}
return 0;
}
HDU2021 进制转换
Problem Description
输入一个十进制数N,将它转换成R进制数输出。
Input
输入数据包含多个测试实例,每个测试实例包含两个整数N(32位整数)和R(2<=R<=16, R<>10)。
Output
为每个测试实例输出转换后的数,每个输出占一行。如果R大于10,则对应的数字规则参考16进制(比如,10用A表示,等等)。
Sample Input
7 2
23 12
-4 3
Sample Output
111
1B
-11
十分朴实的进制转化问题,注意一下负数的处理
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int n, r;
int main() {
while (scanf("%d%d", &n, &r) != EOF) {
if (n < 0) {
cout << "-";
n = - n;
}
if (n == 0) cout << 0 << endl;
else {
int cnt = 0, a[101];
while (n) {
a[cnt++] = n % r;
n /= r;
}
for (int i = cnt - 1; i >= 0; i--) {
if (a[i] >= 10) printf("%c", 'A' + a[i] - 10);
else printf("%d", a[i]);
}
cout << endl;
}
}
return 0;
}
HDU2032 杨辉三角
Problem Description
还记得中学时候学过的杨辉三角吗?具体的定义这里不再描述,你可以参考以下的图形:
1
1 1
1 2 1
1 3 3 1
1 4 6 4 1
1 5 10 10 5 1
Input
输入数据包含多个测试实例,每个测试实例的输入只包含一个正整数n(1<=n<=30),表示将要输出的杨辉三角的层数。
Output
对应于每一个输入,请输出相应层数的杨辉三角,每一层的整数之间用一个空格隔开,每一个杨辉三角后面加一个空行。
Sample Input
2 3
Sample Output
1
1 1
1
1 1
1 2 1
十分经典的杨辉三角问题,递推关系:dp[i][j] = dp[i - 1][j - 1] + dp[i - 1][j]
但不要忘记初始化两边上的数都为1
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int n, dp[101][101];
int main() {
while (scanf("%d", &n) != EOF) {
for (int i = 1; i <= n; i++) {
dp[i][1] = 1;
dp[i][i] = 1;
}
for (int i = 3; i <= n; i++)
for (int j = 2; j < i; j++)
dp[i][j] = dp[i - 1][j - 1] + dp[i - 1][j];
for (int i = 1; i <= n; i++) {
cout << dp[i][1];
for (int j = 2; j <= i; j++)
cout << " " << dp[i][j];
cout << endl;
}
cout << endl;
}
return 0;
}
HDU2033 人见人爱A+B
Problem Description
HDOJ上面已经有10来道A+B的题目了,相信这些题目曾经是大家的最爱,希望今天的这个A+B能给大家带来好运,也希望这个题目能唤起大家对ACM曾经的热爱。
这个题目的A和B不是简单的整数,而是两个时间,A和B 都是由3个整数组成,分别表示时分秒,比如,假设A为34 45 56,就表示A所表示的时间是34小时 45分钟 56秒。
Input
输入数据有多行组成,首先是一个整数N,表示测试实例的个数,然后是N行数据,每行有6个整数AH,AM,AS,BH,BM,BS,分别表示时间A和B所对应的时分秒。题目保证所有的数据合法。
Output
对于每个测试实例,输出A+B,每个输出结果也是由时分秒3部分组成,同时也要满足时间的规则(即:分和秒的取值范围在0~59),每个输出占一行,并且所有的部分都可以用32位整数表示。
Sample Input
2
1 2 3 4 5 6
34 45 56 12 23 34
Sample Output
5 7 9
47 9 30
钟表版A+B,用三个数分别记录hour,minute,second,大于60就向上进位即可
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int n;
int main() {
while (scanf("%d", &n) != EOF) {
while (n--) {
int ah, am, as, bh, bm, bs;
scanf("%d%d%d%d%d%d", &ah, &am, &as, &bh, &bm, &bs);
int ansh, ansm, anss;
ansh = ansm = anss = 0;
anss = as + bs;
ansm = am + bm;
ansh = ah + bh;
if (anss >= 60) {
anss -= 60;
ansm++;
}
if (ansm >= 60) {
ansm -= 60;
ansh++;
}
printf("%d %d %d\n", ansh, ansm, anss);
}
}
return 0;
}
HDU2034 人见人爱A-B
Problem Description
参加过上个月月赛的同学一定还记得其中的一个最简单的题目,就是{A}+{B},那个题目求的是两个集合的并集,今天我们这个A-B求的是两个集合的差,就是做集合的减法运算。(当然,大家都知道集合的定义,就是同一个集合中不会有两个相同的元素,这里还是提醒大家一下)
呵呵,很简单吧?
Input
每组输入数据占1行,每行数据的开始是2个整数n(0<=n<=100)和m(0<=m<=100),分别表示集合A和集合B的元素个数,然后紧跟着n+m个元素,前面n个元素属于集合A,其余的属于集合B. 每个元素为不超出int范围的整数,元素之间有一个空格隔开.
如果n=0并且m=0表示输入的结束,不做处理。
Output
针对每组数据输出一行数据,表示A-B的结果,如果结果为空集合,则输出“NULL”,否则从小到大输出结果,为了简化问题,每个元素后面跟一个空格.
Sample Input
3 3 1 2 3 1 4 7
3 7 2 5 8 2 3 4 5 6 7 8
0 0
Sample Output
2 3
NULL
用两个数组记录集合A和集合B,然后把两者重合的字的flag赋值为1。然后再用一个c数组记录a数组中flag为0的数字(也就是与B不重合的数字),排序一下然后输出~
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int n, m, a[101], b[101], c[101], flag[101];
int main() {
while (scanf("%d%d", &n, &m) != EOF) {
memset(flag, 0, sizeof(flag));
int cnt = 0;
int num = 0;
if (n == 0 && m == 0) break;
for (int i = 1; i <= n; i++) scanf("%d", &a[i]);
for (int i = 1; i <= m; i++) scanf("%d", &b[i]);
for (int i = 1; i <= n; i++)
for (int j = 1; j <= m; j++)
if (a[i] == b[j]) {
flag[i] = 1;
cnt++;
}
if (cnt == n) cout << "NULL" << endl;
else {
for (int i = 1; i <= n; i++)
if (flag[i] == 0)
c[++num] = a[i];
sort(c + 1, c + 1 + num);
for (int i = 1; i <= num; i++) cout << c[i] << " ";
cout << endl;
}
}
return 0;
}
HDU2035 人见人爱A^B
Problem Description
求A^B的最后三位数表示的整数。
说明:A^B的含义是“A的B次方”
Input
输入数据包含多个测试实例,每个实例占一行,由两个正整数A和B组成(1<=A,B<=10000),如果A=0, B=0,则表示输入数据的结束,不做处理。
Output
对于每个测试实例,请输出A^B的最后三位表示的整数,每个输出占一行。
Sample Input
2 3
12 6
6789 10000
0 0
Sample Output
8
984
1
就求A的B次方,大于1000了就模一下
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int a, b;
int main() {
while (scanf("%d%d", &a, &b) != EOF) {
if (a == 0 && b == 0) break;
int num = a;
for (int i = 1; i < b; i++) {
a *= num;
if (a > 1000) a %= 1000;
}
printf("%d\n", a);
}
return 0;
}
HDU2036 改革春风吹满地
Problem Description
“ 改革春风吹满地,
不会AC没关系;
实在不行回老家,
还有一亩三分地。
谢谢!(乐队奏乐)”
话说部分学生心态极好,每天就知道游戏,这次考试如此简单的题目,也是云里雾里,而且,还竟然来这么几句打油诗。
好呀,老师的责任就是帮你解决问题,既然想种田,那就分你一块。
这块田位于浙江省温州市苍南县灵溪镇林家铺子村,多边形形状的一块地,原本是linle 的,现在就准备送给你了。不过,任何事情都没有那么简单,你必须首先告诉我这块地到底有多少面积,如果回答正确才能真正得到这块地。
发愁了吧?就是要让你知道,种地也是需要AC知识的!以后还是好好练吧...
Input
输入数据包含多个测试实例,每个测试实例占一行,每行的开始是一个整数n(3<=n<=100),它表示多边形的边数(当然也是顶点数),然后是按照逆时针顺序给出的n个顶点的坐标(x1, y1, x2, y2... xn, yn),为了简化问题,这里的所有坐标都用整数表示。
输入数据中所有的整数都在32位整数范围内,n=0表示数据的结束,不做处理。
Output
对于每个测试实例,请输出对应的多边形面积,结果精确到小数点后一位小数。
每个实例的输出占一行。
Sample Input
3 0 0 1 0 0 1
4 1 0 0 1 -1 0 0 -1
0
Sample Output
0.5
2.0
数学题啊...看到题目的我:“阿巴阿巴阿巴”
求多边形的面积,百度“多边形面积公式”,出来这玩意
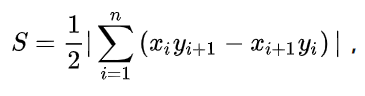
继续阿巴,最后又搜到了S=0.5 * ( (x0 * y1-x1 * y0) + (x1 * y2-x2 * y1) + ... + (xn * y0-x0 * yn) ),虽然意思一样,但第二个看起来就慈祥了许多
得到代码:
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int n;
int main() {
while (scanf("%d", &n) != EOF) {
if (n == 0) break;
int x1, x2, y1, y2;
double ans = 0;
scanf("%d%d", &x1, &y1);
int x = x1;
int y = y1;
for (int i = 0; i < n - 1; i++) {
scanf("%d%d", &x2, &y2);
ans += x1 * y2 - x2 * y1;
x1 = x2;
y1 = y2;
}
ans += x1 * y - y1 * x;
ans /= 2.0;
printf("%.1lf\n", ans);
}
return 0;
}
HDU2037 今年暑假不AC
Problem Description
“今年暑假不AC?”
“是的。”
“那你干什么呢?”
“看世界杯呀,笨蛋!”
“@#$%^&*%....
确实如此,世界杯来了,球迷的节日也来了,估计很多ACMer也会抛开电脑,奔向电视了。
作为球迷,一定想看尽量多的完整的比赛,当然,作为新时代的好青年,你一定还会看一些其它的节目,比如新闻联播(永远不要忘记关心国家大事)、非常6+7、超级女生,以及王小丫的《开心辞典》等等,假设你已经知道了所有你喜欢看的电视节目的转播时间表,你会合理安排吗?(目标是能看尽量多的完整节目)
Input
输入数据包含多个测试实例,每个测试实例的第一行只有一个整数n(n<=100),表示你喜欢看的节目的总数,然后是n行数据,每行包括两个数据Ti_s,Ti_e (1<=i<=n),分别表示第i个节目的开始和结束时间,为了简化问题,每个时间都用一个正整数表示。n=0表示输入结束,不做处理。
Output
对于每个测试实例,输出能完整看到的电视节目的个数,每个测试实例的输出占一行。
Sample Input
12
1 3
3 4
0 7
3 8
15 19
15 20
10 15
8 18
6 12
5 10
4 14
2 9
0
Sample Output
5
很经典的贪心问题,白书上的例题我忘了是哪一个了,依稀记得是什么流星雨,找了一个洛谷上的P1803 凌乱的yyy/线段覆盖相似题
以结束时间为基准从小到大排序,结束时间越早后面能够容纳的比赛也就越多,后面的比赛开始时间≥目前比赛结束时间的话,可观看场数加一,记录此比赛结束时间,依次往后查找更新即可
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int n;
struct node{
int s;
int e;
}a[101];
int cmp(node x, node y) {
if (x.e != y.e) return x.e < y.e;
else return x.s < y.s;
}
int main() {
while (scanf("%d", &n) != EOF) {
if (n == 0) break;
int num = 0;
int cnt = 0;
for (int i = 1; i <= n; i++)
scanf("%d%d", &a[i].s, &a[i].e);
sort(a + 1, a + 1 + n, cmp);
for (int i = 1; i <= n; i++) {
if (i == 1) {
num = a[i].e;
cnt++;
} else {
if (a[i].s >= num) {
cnt++;
num = a[i].e;
}
}
}
printf("%d\n", cnt);
}
return 0;
}
HDU2039 三角形
Problem Description
给定三条边,请你判断一下能不能组成一个三角形。
Input
输入数据第一行包含一个数M,接下有M行,每行一个实例,包含三个正数A,B,C。其中A,B,C <1000;
Output
对于每个测试实例,如果三条边长A,B,C能组成三角形的话,输出YES,否则NO。
Sample Input
2
1 2 3
2 2 2
Sample Output
NO
YES
判断能否组成三角形,小学数学
#include <iostream>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;
int m;
int main() {
while (scanf("%d", &m) != EOF) {
for (int i = 1; i <= m; i++) {
double a, b, c;
scanf("%lf%lf%lf", &a, &b, &c);
if (a + b > c && a + c > b && c + b > a) printf("YES\n");
else printf("NO\n");
}
}
return 0;
}


