已知椭圆\(C:\dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1\) \((a>b>0)\)的左右焦点分别是\(F_1,F_2\),椭圆\(C\)的离心率为\(\dfrac{1}{2}\),且椭圆\(C\)过点\(\left(1,-\dfrac{3}{2}\right)\).
\((1)\) 求椭圆\(C\)的标准方程;
\((2)\) 若直线\(l\)过椭圆\(C\)的左顶点\(M\),且与椭圆\(C\)的另一个交点为\(N\),直线\(NF_2\)与椭圆\(C\)的另一个交点为\(P\),若\(PF_1\perp MN\),求直线\(l\)的方程.
解析:
\((1)\) 由题易得关于\(a,b\)的方程组$$
\dfrac{1}{a2}+\dfrac{9}{4b2}=1,\dfrac{b2}{a2}=\dfrac{3}{4}.
\[ 解得$\left(a,b\right)=\left(2,\sqrt{3}\right)$.从而所求椭圆方程为$\dfrac{x^2}{4}+\dfrac{y^2}{3}=1$.
$(2)$ 由题,设$\angle NF_2x=\theta$,则由椭圆的焦半径公式$\rm{II}$可得
$|NF_2|=\dfrac{3}{2+\cos\theta}$,从而<p style="text-align:center;">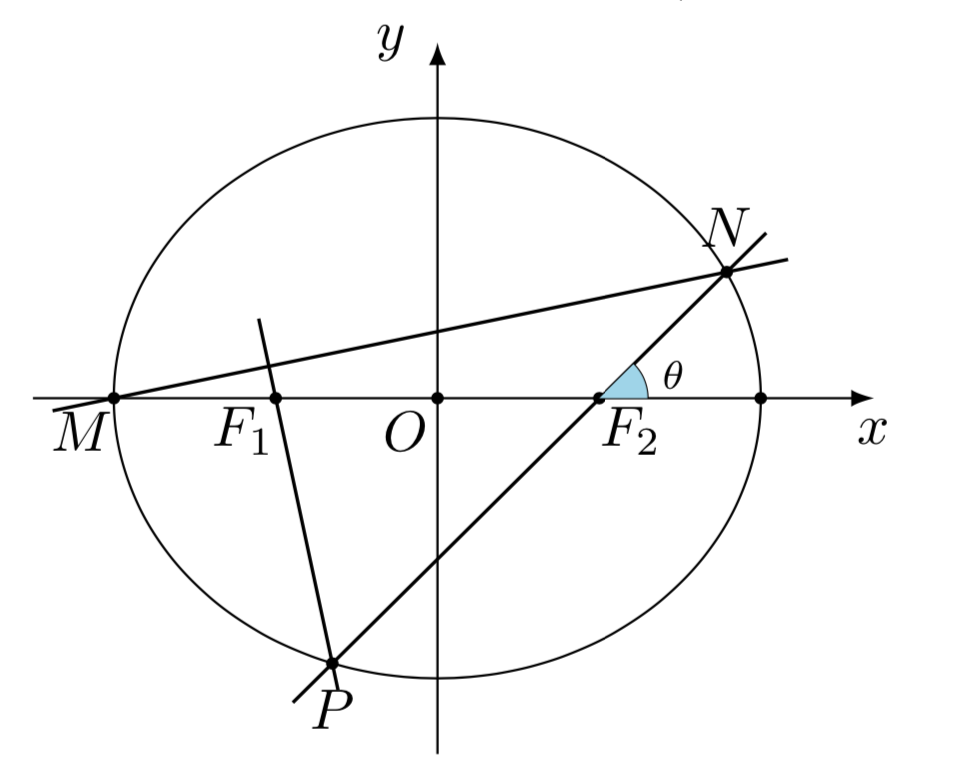</p>可得$N$点的坐标$N\left(\dfrac{3\cos\theta}{2+\cos\theta}+1,\dfrac{3\sin\theta}{2+\cos\theta}\right)$,同理可得$P$点坐标$P\left(\dfrac{-3\cos\theta}{2-\cos\theta}+1,\dfrac{-3\sin\theta}{2-\cos\theta}\right)$,由于$$PF_1\perp MN \Rightarrow
\overrightarrow{PF_1}\cdot \overrightarrow{MN}=0.\]
整理可得关于$\theta$的方程$$
(7\cos\theta-5)\left(\cos\theta+1\right)=0.$$
由于$M,N$两点不重合,因此$\cos\theta+1\neq 0$,从而当且仅当$\cos\theta=\dfrac{5}{7}$对应的直线$l$即为所求.且该直线方程为$$
l: \sqrt{6}x-12y+2\sqrt{6}=0.$$


