Chapter 9 Measurement Bias

目录

• 9.1 Measurement Error
• The structure of measurement error
• 9.3 Mismeasured confounders
• 9.4 Intention-to-treat effect: the effect of a misclassified treatment
• 9.5 Per-protocol effect
• Fine Point
  • The strength and direction of measurement bias
  • Per-protocol analyses
  • Pseudo-intention-to-treat analyses
  • Effectiveness versus efficacy
• Technical Point
  • Independence and nondifferentiality
  • The exclusion restriction

Hern\(\'{a}\)n M. and Robins J. Causal Inference: What If.

已经介绍过两个bias: confounding和selection, 这里介绍第三个, measurement bias.
这个measurement bias 不是指样本数目过少导致的误差, 用记录误差更为准确, 比如明明是\(A=1\), 但是记录成了\(A=0\).

9.1 Measurement Error

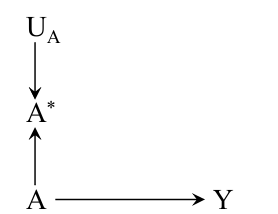
如上图所示, 我们所希望的实际上只有\(A \rightarrow Y\)这一条先, 但是由于measurement error \(U_A\)导致我们最后得到的只有\(A^*\), 此时想要计算\(A\)关于\(Y\)的causal effect就比较麻烦了.

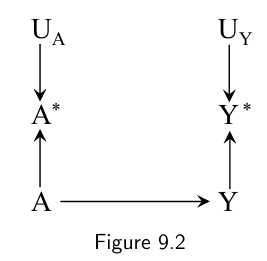
不光是intervention, outcome \(Y\)实际上也可能会有measurement error.

The structure of measurement error

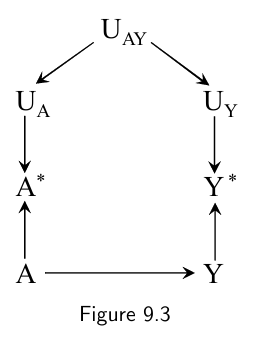
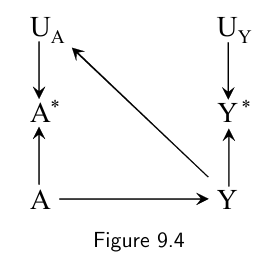
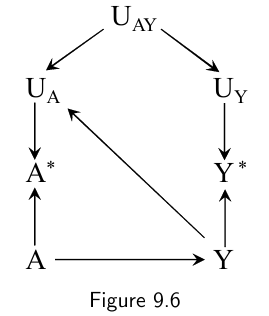
measurement error 可以分成四种:

• independent nondifferential: Figure 9.2;
• dependent nondifferential: Figure 9.3;
• independent differential: Figure 9.4;
• dependent differential: Figure 9.6.

9.3 Mismeasured confounders

这里介绍了另外一种特殊的情况, \(A, Y\)都没有测量误差, 但是\(L\)是存在测量误差的, 此时因为缺乏\(L\), 我们没法依赖其获得想要的条件可交换性.

同样的, 类似的情况也可能导致selection bias.

9.4 Intention-to-treat effect: the effect of a misclassified treatment

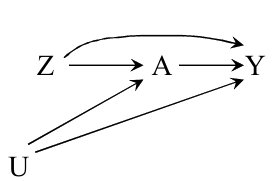
这一节讨论的是, 预先打算的策略\(Z\)对\(Y\)的causal effect和实际进行的\(A\)关于\(Y\)的causal effect之间的差别.

9.5 Per-protocol effect

主要讲了什么时候Intention-to-treat effect会是一个不错的选择.

Fine Point

The strength and direction of measurement bias

Per-protocol analyses

• as treated: 只管\(A\), 不管\(Z\)(所以是不考虑\(Z\)的存在?).
• per protocol: 只在\(A=Z\)的子集上讨论, 所以这种很有可能会导致selection bias. 如Figure 9.14 所示.

Pseudo-intention-to-treat analyses

Effectiveness versus efficacy

Technical Point

Independence and nondifferentiality

The exclusion restriction

指的是没有直接的箭头由assign treatment \(Z\) 指向 outcome \(Y\).