[LeetCode] 236. Lowest Common Ancestor of a Binary Tree_ Medium tag: DFS, Divide and conquer

Given a binary tree, find the lowest common ancestor (LCA) of two given nodes in the tree.

According to the definition of LCA on Wikipedia: “The lowest common ancestor is defined between two nodes p and q as the lowest node in T that has both p and q as descendants (where we allow a node to be a descendant of itself).”

Given the following binary tree:  root = [3,5,1,6,2,0,8,null,null,7,4]

 

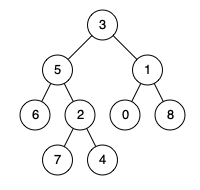
Example 1:

Input: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 1
Output: 3
Explanation: The LCA of nodes 5 and 1 is 3.

Example 2:

Input: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 4
Output: 5
Explanation: The LCA of nodes 5 and 4 is 5, since a node can be a descendant of itself according to the LCA definition.

 

Note:

• All of the nodes' values will be unique.
• p and q are different and both values will exist in the binary tree.

这个题目还是用Divide and Conquer的思想，然后分别在左右两边tree里面找是否有p，或者g，一旦有了，就分别往上面传，只有在left tree， right tree 各有一个点时，就是lowest common ancestor。

 

Time：O(n),   S: O(n)

Code：

class Solution:
    def LCA(self, root, p, g):
        if not root or root == p or root == g:
            return root
        left = self.LCA(root.left, p, g)
        right = self.LCA(root.right, p, g)
        if left and right:
            return root
        elif left:
            return left
        elif right:
            return right
        return 

 

=> 更简洁

class Solution:
    def LCA(self, root, p, g):
        if root in [None, p, g]: return root
        left, right = self.LCA(root.left, p, g), self.LCA(root.right, p, g)
        return root if left and right else left or right