弹性波动力学笔记(四) 应力张量简介上

3. The Stress Tensor

The development of the theory of elasticity took about two centuries, beginning with Galileo in the 1600s. The most difficult problem was to gain an understanding of the force involved in an elastic body. This problem was addressed by assuming the existence of attractive and repulsive forces between the molecules of a body.

The most successful of the theories based on this assumption was that of Navier, who in 1821 presented the equations of motion for an elastic isotropic solid (Hudson, 1980; Timoshenko, 1953). Navier's results were essentially correct, but because of the molecular assumptions made, only one elastic constant was required, as opposed to the two that characterize an isotropic by the earlier researchers can be obtained by setting the ratio of P- to S-wave velocities to \(\sqrt{3}\) in the more general results derived later.

Navier's work attracted the attention of the famous mathematician Cauchy, who in 1822 introduced the concept of stress as we know it today. Instead of considering intermolecular forces, Cauchy introduced the idea of pressure on surfaces internal to the body, with the pressure not perpendicular to the surface, as it would be in the case of hydrostatic pressure. This led to the concept of stress, which is much more complicated than that of strain, and which requires additional continuum mechanics concepts for a full study. The relevant results are summarized below and in several problems. Very readable presentations of this material are provided by Atkin and Fox (1980), Hunter (1976) and Mase (1970).

3.1 Additional Continuum Mechanics Concepts

Let us use the Eulerian description of motion, and let \(p(\bf{r},t)\) indicate the value of some property of the medium (e.g., pressure, temperature, velocity) at given point \(\mathbf{r}\) at time \(t\). As \(t\) varies, different particles (identified by different values of \(\mathbf{R}\)) occupy the same spatial point \(\mathbf{r}\). Now let us concentrate on a single \(\mathbf{R}\). Using

\[\mathbf{r}=\mathbf{r}(\mathbf{R},t) \tag{3.1.1} \]

we find

\[P(\mathbf{R},t)=p(\mathbf{r}(\mathbf{R},t),t) \tag{3.1.2} \]

Note that, in general, \(P\) and \(p\) will have different functional forms.

When a body is in motion, the description of the time rate of change of a given property depends on how the motion is described. To motivate the definitions below, consider the following situation. Assume that we are interested in measuring the time rate of change of some property (such as the temperature) of a river as a function of position and tome. We can do at least two things. One is to conduct the measurements at a point that remains fixed with respect to the shoreline. This pint will have a position given by \(\mathbf{r}\) (in some coordinate system). The local time rate of change obtained in this way is the partial derivative of \(p(\mathbf{r},t)\) with respect to \(t\), indicated by \(\part{p}/\part{t}\).

A second thing we can do is to measure the property from a canoe that floats along the river. The canoe (which is representative of a particle in a continuous medium) is identified by the vector \(\mathbf{R}\) (having the same origin as the vector \(\mathbf{r}\) of the fixed point referred to above). The time rate of change determined from these measurements is known as the material derivative of \(P\). More specifically, the material time derivative of a quantity P, indicated by \(D{P}/D{t}\), is the time rate of change of \(P\) as would be recorded by an observer moving with the particle identified by \(\mathbf{R}\) and is written as

\[\frac{D{P}}{D{t}}=\frac{\part{P}(\mathbf{R},t)}{\part{t}}\rvert_{\mathbf{R}fixed} \tag{3.1.3} \]

The quantity \(P\) represents any scalar, vector, or tensor property of the medium.

If \(P\) is expressed in terms of \(\mathbf{r}\) the material derivative becomes

\[\frac{D{P}}{D{t}}=\frac{\part{p(\mathbf{r},t)}}{\part{t}}\lvert_{\mathbf{r}fixed}+\frac{\part{p(\mathbf{r},t)}}{\part{x_k}}\frac{\part{x_k}}{\part{t}}\lvert_{\mathbf{R}fixed} \tag{3.1.4} \]

Here equation(3.1.1) and the chain rule of partial derivatives have been used. With two exceptions, in the following the following the subscripts \(\mathbf{r}\) and \(\mathbf{R}\) and the label 'fixed' will be dropped to simplify the notation.

The first term on the right-hand side of (3.1.4) is the local time rate of change defined above. The second term is known as the convective time rate of change, and arises from the motion of the particles in the medium. The material derivative is also known as the substantial derivative.

The velocity of a particle is defined as the material time rate of change of the position of the particle

\[\mathbf{v}=\frac{D\mathbf{r}}{D{t}}=\frac{\part \mathbf{r}(\mathbf{R},t)}{\part{t}}\lvert_{\mathbf{R}} \tag{3.1.5} \]

or, in component form,

\[v_k=\frac{\part{x_k}}{\part{t}} \tag{3.1.6} \]

As defined, \(\mathbf{v}\) is a function of a particular particle (identified by \(\mathbf{R}\)) and \(t\), which is a material description. Therefore, we should have used \(\mathbf{V}\) instead of \(\mathbf{v}\), but this distinction is not always made explicitly. With this caveat we can use and write

\[\mathbf{v}=\mathbf{V}(\mathbf{R},t)=\mathbf{V}(\mathbf{R}(\mathbf{r},t),t)=\mathbf{v}(\mathbf{r},t) \tag{3.1.7} \]

where \(\mathbf{v}(\mathbf{r},t)\) represents the velocity field field in spatial description. In this description, \(\mathbf{v}\) is known for all points in the medium. The particle \(\mathbf{R}\) that happens to be at a point \(\mathbf{r}\) at given time \(t\) will have velocity \(\mathbf{v}(\mathbf{r},t)\).

A comparison of equation (3.1.4) and (3.1.6) shows that the second term on the right-hand side of (3.1.4) is the scalar product of \((\nabla p)_k\) and \(v_k\). Therefore, equation(3.1.4) can be rewritten as

\[\frac{D{p}}{D{t}}=\frac{\part{p}}{\part{t}}+(\mathbf{v}\cdot\nabla)p. \tag{3.1.8} \]

Expressing \(\mathbf{r}\) in terms of \(\mathbf{u}\) equation (3.1.5) gives

\[\mathbf{v}=\frac{D(\mathbf{u+R})}{D{t}}=\frac{\part(\mathbf{u}(\mathbf{R},t)+\mathbf{R})}{\part{t}}\lvert_{\mathbf{R}}=\frac{\part\mathbf{u}(\mathbf{R},t)}{\part{t}} \tag{3.1.9} \]

Because \(\mathbf{R}\) is independent of time, \(\part{\mathbf{R}}/\part{t}=0\).

If \(\mathbf{u}\) is given in the spatial description, then \(v_k\) is obtained using equation (3.1.4) gives

\[v_k=\frac{\part{u_k}}{\part{t}}+\frac{\part{u_k}}{\part{x_l}}\frac{\part{x_l}}{\part{t}}=\frac{\part{u_k}}{\part{t}}+(\mathbf{v}\cdot\nabla)u_k \tag{3.1.10} \]

or, in vector form,

\[\mathbf{v}=\frac{\part{\mathbf{u}}}{\part{t}}+(\mathbf{v}\cdot\nabla)\mathbf{u} \tag{3.1.11} \]

Note that the velocity is given in implicit form.

The acceleration of a particle is the material time rate of change of the velocity of a particle:

\[\mathbf{a}=\frac{D{\mathbf{v}}}{D{t}} \tag{3.1.12} \]

In the Eulerian description we have

\[a_k=\frac{\part{v_k}}{\part{t}}+\frac{\part{v_k}}{\part{x_l}}\frac{\part{x_l}}{\part{t}}=\frac{\part{v_k}}{\part{t}}+(\mathbf{v}\cdot\nabla)v_k \tag{3.1.13} \]

or, in vector form,

\[\mathbf{a}=\frac{\part{\mathbf{v}}}{\part{t}}+(\mathbf{v}\cdot\nabla)\mathbf{v} \tag{3.1.14} \]

These definitions of velocity and acceleration will become clear with the example below. To complete this section it is necessary to add a number of basic definitions and principles, as follows.

Mass. The mass \(m\) of a volume \(V\) of a body having variable density \(\rho\) is given by

\[m=\int_{v}\rho dV. \tag{3.1.15} \]

Linear momentum:

\[ \mathbf{P}=\int_{V}\rho\mathbf{v}d{V}\tag{3.1.16} \]

Angular momentum:

\[\mathbf{M}=\int_{V}\mathbf{r}\times\rho\mathbf{v}d{V} \tag{3.1.17} \]

The last two definitions can be viewed as extensions of similar concepts in classical mechanics.

Conservation of mass:

\[\frac{dm}{dt}=0 \tag{3.1.18} \]

Balance of linear momentum:

\[\frac{d{\mathbf{P}}}{d{t}}= \int_V\mathbf{f}dV \tag{3.1.19} \]

Balance of angular momentum:

\[\frac{d{\mathbf{M}}}{dt}=\int_V\tau(v)dV \tag{3.1.20} \]

The last two principles have classical mechanics counterparts, and are due to Euler, another famous mathematician of the eighteenth century. The balance of linear momentum, in particular, is the equivalent of Newton’s second law. It is important to note, however, that these principles should be viewed as axioms justified by the usefulness of the theories based on them.

3.2 The Stress Vector

In continuum mechanics two different types of forces are recognized, body forces, which act at a distance within a body or between bodies, and surface forces, which only depend on the surface of contact of either two bodies in contact or any two portions of a body separated by an imaginary surface. Examples of body forces are the gravitational forces, which, as noted, may be the result of the action of the particles within a body, or may originate in another body. The effect of gravitation is generally ignored in wave propagation studies that do not involve the whole Earth. An example of a surface force distributions is the hydrostatic pressure on the surface of a body immersed in a fluid. Other forces, such as the magnetic force, and force distribution, such as surface or volume distributions of couples are possible, but they are not required in elasticity studies.

To introduce the concept of stress vector we follow Love (1927) and Ben-Menahem and Singh (1981). Consider any plane surface \(\Delta{S}\) within a body and a point \(P\) on the surface. Let \(\mathbf{n}\) be one of the two possible normals to \(\Delta S\). The Surface divides the body into two portions, to be called media \(\text{I}\) and \(\text{II}\), where medium I contains \(\mathbf{n}\). Now assume that medium \(\text{I}\) exerts a force on medium \(\text{II}\) across the surface \(\Delta{S}\), and this force is equivalent to a force \(\Delta{\mathbf{F}}\) acting at \(P\) plus a couple \(\Delta{\bf{C}}\) about some axis. Furthermore, assume that as the surface is continuously contracted around \(P\) , both \(\Delta{\bf{F}}\) and \(\Delta{\bf{C}}\) go to zero, with the direction of \(\Delta{\bf{F}}\) reaching some limiting direction. Finally, assume that the ratio \(\Delta{\bf{C}}/\Delta{S}\) goes to zero while the \(\Delta{\bf{F}}/\Delta{S}\) has a finite limit, known as the stress vector or traction, which can be written as

\[\mathbf{T}=\lim \limits_{\Delta{S}\rightarrow{0}}\frac{\Delta{\mathbf{F}}}{\Delta{S}} \tag{3.2.1} \]

where \(\mathbf{T}(\mathbf{n})\) is the stress vector at point P associated with the normal vector \(\mathbf{n}\). Note that changing \(\mathbf{n}\) will change \(\mathbf{T}(\mathbf{n})\), and that, in general, \(\mathbf{T}{(\bf{n})}\) may depend on the coordinates of the point P and the time t, but to simplify the notation we will not use them explicitly. As \(\bf{T}\) is a force per unit area, it has the dimensions of pressure. The projection of \(\bf{T}\) on \(\bf{n}\) is given by \(\bf{T}\cdot \bf{n}\). If this projection is positive, it corresponds to a tension; if negative to a compression. For the case of hydrostatic pressure, the force and the normal are in opposite directions. For solids, they are usually in different directions. From equation (3.2.1) we also see that when \(\bf{T}\) is given as a function of position, then the force across any infinitesimal surface element \(dS\) will be equal to \(\bf{T}\cdot d{S}\) .

​

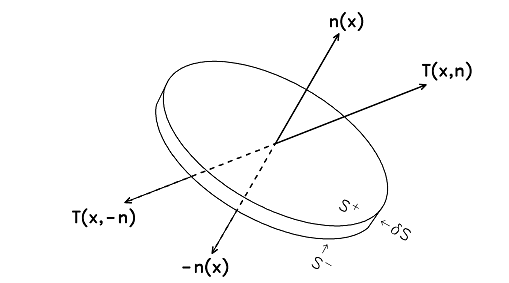
Fig 2. Disk-shaped volume element used to show that\(\mathbf{T(x,-n)}=-\mathbf{T(x,n)}\).

Before proceeding two comments are in order. First, the assumptions that lead to equation (3.2.1) are justified by the success of the theory of elastic materials (Hudson, 1980). Secondly, we have implicitly used an Eulerian approach, i.e., \(\bf{n}\) and \(\Delta{S}\) are referred to the deformed state. When the undeformed state is used as a reference, the analysis of stress is considerably more complicated. A major difference is that in that case the stress tensor, known as the Piola-Kirchhoff stress tensor, is not symmetric. These questions, however, can be ignored in most wave propagation applications.

Now we will use the principle of linear momentum to show that \(\bf{T}(-\bf{n})=-\bf{T({\bf{n}})}\). Let \(S\) be the surface of a body with volume \(V\), and let \(\bf{f}\) be the body force per unit mass, so that \(\rho\bf{f}\) is a force per unit volume. To apply to an arbitrary body of volume \(V\) and surface \(S\) it is necessary to find the total surface forces, represented by integrals below. Then, form () and () we find

\[\frac{d}{dt}{\int_{V}{\rho}\bf{v}}dV=\int_S{\bf{T}}dS+\int_V{\rho\bf{f}}dV \tag{3.2.2} \]

Another result we need is

\[ \frac{d}{dt}{\int_V{\rho\bf{v}}}dV=\int_{V}\rho\frac{D\bf{v}}{Dt}dV \tag{3.2.3} \]

Equations (3.2.2) and (3.2.3) will be applied to a disk-shaped volume having a thickness that allowed to go zero. Under these conditions the integrals and the integral over the surface \(\Delta{S}\) go to zero, and equation (3.2.2) becomes

\[ \int_{S^+}\bf{T}(\bf{n})dS^{+}+\int_{S^{-}}\bf{T}(-\bf{n})dS^{-}=0,\tag{3.2.4} \]

where \(S^{+}\) and \(S^{-}\) are the two surfaces to the disk, and \(\bf{n}\) is the normal to \(S^{+}\). As the thickness of the disk goes to zero, \(S^{-}\) approaches \(S^{+}\), and because \(S^{+}\) is arbitrary， implies that

\[\mathbf{T}(-\bf{n})=-T(\mathbf{\bf{n}}) \tag{3.2.5} \]

as long as \(\bf{T}\) is continuous on \(S^{+}\) (Hudson,1980). Equation (3.2.5) is similar to Newton's law of action and reaction.

3.3 The Stress Tensor

Here we will find a functional relationship between \(\bf{T}(\bf{n})\) and \(\bf{n}\), which automatically introduces the stress tensor. Consider an infinitesimal tetrahedron with three faces along the coordinate planes. \(\bf{T}(\bf{n})\) is the stress tensor across the plane ABC having a normal \(\bf{n}\). \(d{S_i}\) is the surface normal to coordinate axis \(x_i\). Therefore, the normal to \(dS_i\) is \(-\bf{e}_i\) and the normal to \(d{S_n}\) is \(\bf{n}\). Note that all the normals are directed outwards.

Fig 3. Tetrahedron used to introduce the stress tensor.

Now apply (3.3.2) and (3.3.3) to the tetrahedron. Because the volume integrals go to zero faster than the surface integral as the volume of the tetrahedron goes to zero, we have to consider surface forces only. Let \(S\) be the surface of the tetrahedron, so that \(S=d{S_n}+d{S_1}+d{S_2}+d{S_3}\). Therefore,

\[0=\int_{S}\mathbf{T(n)}dS=\mathbf{T(n)}dS_n+\mathbf{T(-e_1)}dS_1+\mathbf{T(-e_2)}dS_2+\mathbf{T(-e_3)}dS_3 \tag{3.3.1} \]

Strictly speaking, on the right-hand side of equation (3.3.1) we should have written the integrals over the faces of the tetrahedron, but because they are assumed to be infinitesimal surfaces, each integral can be replaced by the product of the stress tensor at point \(P\) and surface area. Then, using equation (3.2.5) we obtain

\[\mathbf{T(n)}dS_n=\mathbf{T(e_1)}dS_1+\mathbf{T(e_2)}dS_2+\mathbf{T(e_3)}dS_3 \tag{3.3.2} \]

Equation (3.3.2) can be simplified even further because

\[dS_i=(\mathbf{n}\cdot\mathbf{e}_{i})dS_n=n_idS_n; \space i=1,2,3 \tag{3.3.3} \]

Therefore,

\[\mathbf{T(n)}=n_1\mathbf{T(e_1)}+n_2\mathbf{T(e_2)}+n_3\mathbf{T(e_3)} \tag{3.3.4} \]

The vectors \(\mathbf{T(e_i)}\) can be written in terms of the unit vectors:

\[\begin{equation} \begin{aligned} \mathbf{T}(\mathbf{e}_1)=\tau_{11}\mathbf{e}_1+\tau_{12}\mathbf{e}_2+\tau_{13}\mathbf{e}_3 \\ \mathbf{T}(\mathbf{e}_2)=\tau_{21}\mathbf{e}_1+\tau_{22}\mathbf{e}_2+\tau_{23}\mathbf{e}_3 \\ \mathbf{T}(\mathbf{e}_3)=\tau_{31}\mathbf{e}_1+\tau_{32}\mathbf{e}_2+\tau_{33}\mathbf{e}_3 \end{aligned} \tag{3.3.5} \end{equation} \]

where \(\tau_{ij}\) is the \(x_j\) component of the stress vector corresponding to the plane having \(\mathbf{e_i}\) as a normal. \(\tau_{ij}\) constitute the elements of the stress tensor.

Using the summation convention, equations （3.3.4）and (3.3.5) can be rewritten as

\[\mathbf{T}(\mathbf{n})=n_i\mathbf{T}(\mathbf{e}_i) \tag{3.3.6} \]

\[\mathbf{T}(\mathbf{e}_i)=\sum_{j=1}^{3}\tau_{ij}\mathbf{e}_{j}=\tau_{ij}\mathbf{e}_j;\space i=1,2,3 \tag{3.3.7} \]

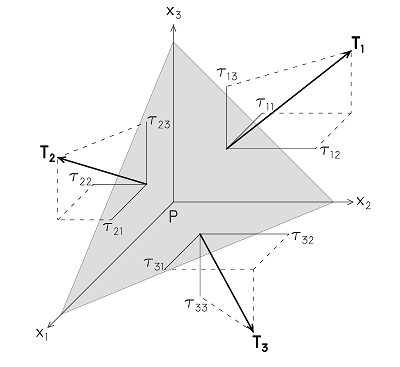
Fig 4. The components of the stress tensor \(\tau_{ij}\) are the components of the stress vector

Then, from (3.3.6) and (3.3.7) we find

\[\mathbf{T}(\mathbf{n})=n_i\tau_{ij}\mathbf{e_j} \tag{3.3.8} \]

The vector \(T(\mathbf{n})\) can also be written as

\[\mathbf{T}(\mathbf{n})=T_1(\mathbf{n})\mathbf{e}_1+T_2(\mathbf{n})\mathbf{e}_2+T_3(\mathbf{n})\mathbf{e}_3=T_j(\mathbf{n})\mathbf{e}_j \tag{3.3.9} \]

show that comparison with equation (3.3.8) shows that

\[T_j(\mathbf{n})=n_i\tau_{ij}; \space j=1,2,3 \tag{3.3.10} \]

As for the case of the strain tensor, one should show that \(\tau_{ij}\) is a tensor, but here this is not necessary because (3.3.10) was used to motivate the definition of a second-order tensor.

Now, using

\[n_i=\mathbf{n}\cdot\mathbf{e}_i \tag{3.3.11} \]

equation (3.3.6) can be written as

\[ \mathbf{T}(\mathbf{n})=(\mathbf{n}\cdot\mathbf{e}_j)\mathbf{T}(\mathbf{e}_i)=\mathbf{n}\cdot(\mathbf{e}_i\mathbf{T}(\mathbf{e}_i)) \tag{3.3.12} \]

The factor in parentheses is known as the stress dyadic:

\[\Tau=\sum_{i=1}^{3}\mathbf{e}_i\mathbf{T(\mathbf{e}_i)}=\sum_{i=1}^{3}\sum_{j=1}^{3}\tau_{ij}\mathbf{e}_{i}\mathbf{e}_{j} \tag{3.3.13} \]

In term of the stress dyadic, the stress vector can be written as

\[ \mathbf{T}=\mathbf{n}\cdot\rm T \tag{3.3.14} \]

Note that although the components \(\tau_{ij}\) depend on the coordinate system used, the relationship indicated by (3.3.15) is independent of the reference frame. Also note that when \(\tau_{ij}\), or \(\rm T\) , and \(\mathbf{n}\) are given, the stress vector may be computed using (3.3.10) or(3.3.14). The operations involved may be clearer when written in matrix form:

\[\left( {\begin{array}{*{20}{c}} {{T_1}}&{{T_2}}&{{T_3}} \end{array}} \right) = \left( {\begin{array}{*{20}{c}} {{n_1}}&{{n_2}}&{{n_3}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{\tau _{11}}}&{{\tau _{12}}}&{{\tau _{13}}}\\ {{\tau _{21}}}&{{\tau _{22}}}&{{\tau _{23}}}\\ {{\tau _{31}}}&{{\tau _{32}}}&{{\tau _{33}}} \end{array}} \right) \tag{3.3.15} \]

The diagonal elements of this matrix are know as normal stresses, while the off-diagonal elements are known as shearing stresses.