HDU4372 Count the Buildings —— 组合数 + 第一类斯特林数

题目链接：https://vjudge.net/problem/HDU-4372

 

Count the Buildings

Time Limit: 2000/1000 MS (Java/Others)    Memory Limit: 65536/65536 K (Java/Others)
Total Submission(s): 2509    Accepted Submission(s): 815

Problem Description

There are N buildings standing in a straight line in the City, numbered from 1 to N. The heights of all the buildings are distinct and between 1 and N. You can see F buildings when you standing in front of the first building and looking forward, and B buildings when you are behind the last building and looking backward. A building can be seen if the building is higher than any building between you and it.
Now, given N, F, B, your task is to figure out how many ways all the buildings can be.

 

 

Input

First line of the input is a single integer T (T<=100000), indicating there are T test cases followed.
Next T lines, each line consists of three integer N, F, B, (0<N, F, B<=2000) described above.

 

 

Output

For each case, you should output the number of ways mod 1000000007(1e9+7).

 

 

Sample Input

2 3 2 2 3 2 1

 

 

Sample Output

2 1

 

 

Source

2012 Multi-University Training Contest 8

 

 

题意：

有n幢高度不一的楼房位于一条直线上，问有多少种方案数，使得人从第一幢往前看时看到f幢，从最后一幢往后看时看到b幢？

 

题解：

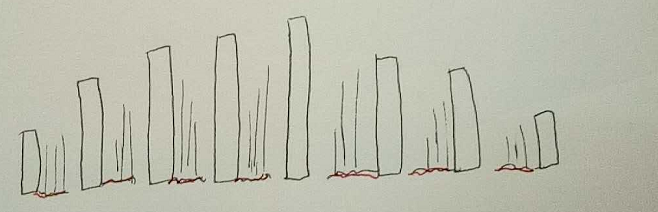
1.可知最高的那栋必定能够看到，于是就分成了左边和右边。

2.对于左边的楼而言，需要把他们分成f-1组，每一组的最高楼在最左边，这样就把组内其他的楼遮住了，于是就看到f-1栋。对于右边的也如此。

3.那怎么分组呢？首先，求出求出第一类斯特林数 S[n-1][f-1+b-1]，即把除了最高楼之外的楼房排成f-1+b-1个圈。由于每一组中最高的楼房固定在左边或右边，这样就对应了圈。换句话说，对排列好的一个圈选定一栋楼房，而这栋楼房就是最高的那栋，然后再把这个圈展开成一列，这样就对应了一组。所以可以用第一类斯特林数求出分组的方案数。

4.分好组后，就直接从 f+b-2组中抽取f-1组放在左边（由于要求递增，所以选出来之后他们的位置就固定了，不需要再排列），总有 C[f+b-2][f-1]种选择。

5.综上，总共有 S[n-1][f-1+b-1] * C[f+b-2][f-1] 种方案数。注意， 当n-1<f+b-2时, 问题无解。

 

 

代码如下：

#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
#include <vector>
#include <cmath>
#include <queue>
#include <stack>
#include <map>
#include <string>
#include <set>
using namespace std;
typedef long long LL;
const int INF = 2e9;
const LL LNF = 9e18;
const int MOD = 1e9+7;
const int MAXN = 2e3+10;

LL S[MAXN][MAXN], C[MAXN][MAXN];

void init()
{
    for(int i = 0; i<MAXN; i++)
    {
        C[i][0] = 1;
        for(int j = 1; j<=i; j++)
            C[i][j] = (C[i-1][j-1]+C[i-1][j])%MOD;
    }

    memset(S, 0, sizeof(S));
    for(int i = 1; i<MAXN; i++)
    {
        S[i][0] = 0; S[i][i] = 1;
        for(int j = 1; j<i; j++)
            S[i][j] = (((i-1)*S[i-1][j])%MOD + S[i-1][j-1])%MOD;
    }
}

int main()
{
    init();
    int T, n, f, b;
    scanf("%d", &T);
    while(T--)
    {
        scanf("%d%d%d", &n, &f, &b);
        LL ans;
        if(n-1<f+b-2) ans = 0;
        else ans = (1LL*S[n-1][f+b-2]*C[f+b-2][f-1])%MOD;
        printf("%lld\n", ans);
    }
}