[BZOJ] 1712: [Usaco2007 China]Summing Sums 加密

1712: [Usaco2007 China]Summing Sums 加密

Time Limit: 5 Sec  Memory Limit: 64 MB
Submit: 526  Solved: 205
[Submit][Status][Discuss]

Description

    那N只可爱的奶牛刚刚学习了有关密码的许多算法，终于，她们创造出了属于奶牛的加密方法．由于她们并不是经验十足，她们的加密方法非常简单：第i只奶牛掌握着密码的第i个数字，起始的时候是Ci(0≤Ci<90000000).加密的时候，第i只奶牛会计算其他所有奶牛的数字和，并将这个数字和除以98765431取余．在所有奶牛计算完毕之后，每一只奶牛会用自己算得的数字代替原有的数字．也就是说，

这样，她们就完成了一次加密．    在十一月，奶牛们把这个加密法则告诉了驼鹿卡门，卡门惊呆了．之后，在一个浓雾弥漫的平安夜，卡门与奶牛们：“你们的算法十分原始，很容易就被人破解．所以你们要重复这个加密过程T(1≤T≤1414213562)次，才能达到加密效果．”    这回轮到奶牛们惊呆了．很显然，奶牛们特别讨厌做同样的无聊的事情很多次．经过了漫长的争论，卡门和奶牛们终于找到的解决办法：你被刚来加密这些数字．

Input

    第1行输入N和T，之后N行每行一个整数表示初始的Ci．

Output

 

    共N行，每行一个整数，表示T次加密之后的Ci．

Sample Input

3 4
1
0
4

INPUT DETAILS:

Three cows, with starting numbers 1, 0, and 4; four repetitions of the
encryption algorithm.

Sample Output

26
25
29

OUTPUT DETAILS:

The following is a table of the cows' numbers for each turn:

Cows' numbers
Turn Cow1 Cow2 Cow3
0 1 0 4
1 4 5 1
2 6 5 9
3 14 15 11
4 26 25 29

HINT

 

 N<=50000

 

Source

Gold

 

Analysis

瞬间想到矩阵快速幂

但是令人沮丧的是： n <= 500000

就算时间复杂度压下来了，空间复杂度也会爆的

所以翻了一发题解

发现可以根据单个元素进行构造矩阵

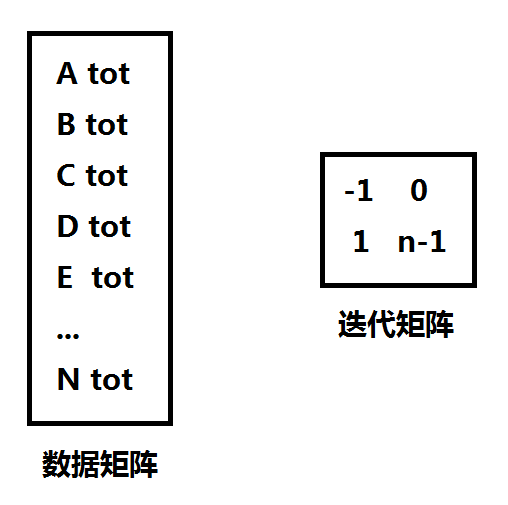
之所以这样是因为经过模拟发现tot有这样的性质

（tot指当前所有数之和）

每次迭代后 tot 为原来的 n-1 倍

其余细节不难思考

 

Code

#include<cstdio>
#include<iostream>
#define mod 98765431
using namespace std;

long long arr[100000][3],brr[100000][3],n,t,tot;

struct MAT{
    long long mat[10][10];
    MAT(){
        for(int i = 0;i < 10;i++)
        for(int j = 0;j < 10;j++)
            mat[i][j] = 0;
    }
};

MAT mul(MAT A,MAT B){
    MAT C;
    for(int i = 1;i <= 2;i++)
        for(int j = 1;j <= 2;j++)
            for(int k = 1;k <= 2;k++)
                C.mat[i][j] = (C.mat[i][j]+A.mat[i][k]*B.mat[k][j])%mod;
    return C;
}

MAT ksm(MAT A,long long k){
    if(k == 1) return A;
    MAT B = A; k--;
    while(k){
        if(k&1) B = mul(B,A);
        A = mul(A,A);
        k >>= 1;
    }return B;
}

int main(){
    scanf("%lld%lld",&n,&t);
    for(int i = 1;i <= n;i++)
        scanf("%lld",&arr[i][1]);
    MAT BUF;
    BUF.mat[1][1] = -1;
    BUF.mat[1][2] = 0;
    BUF.mat[2][1] = 1;
    BUF.mat[2][2] = n-1;
    
    BUF = ksm(BUF,t);
    
    for(int i = 1;i <= n;i++) tot += arr[i][1];
    for(int i = 1;i <= n;i++) arr[i][2] = tot%mod;
    
    for(int i = 1;i <= n;i++)
    for(int j = 1;j <= 2;j++)
    for(int k = 1;k <= 2;k++)
        brr[i][j] = (brr[i][j]+arr[i][k]*BUF.mat[k][j])%mod;
        
    for(int i = 1;i <= n;i++)
        printf("%lld\n",brr[i][1]);
    
    return 0;
}