POJ 1279 Art Gallery (半平面交求多边形的核,求核的面积)
Art Gallery
| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions: 5705 | Accepted: 2410 |
Description
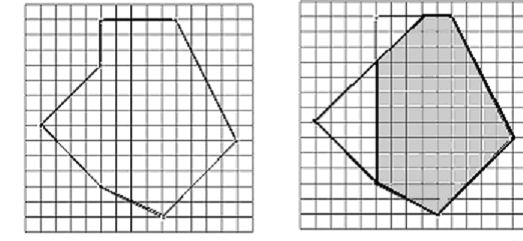
The art galleries of the new and very futuristic building of the Center for Balkan Cooperation have the form of polygons (not necessarily convex). When a big exhibition is organized, watching over all of the pictures is a big security concern. Your task is that for a given gallery to write a program which finds the surface of the area of the floor, from which each point on the walls of the gallery is visible. On the figure 1. a map of a gallery is given in some co-ordinate system. The area wanted is shaded on the figure 2.

Input
The number of tasks T that your program have to solve will be on the first row of the input file. Input data for each task start with an integer N, 5 <= N <= 1500. Each of the next N rows of the input will contain the co-ordinates of a vertex of the polygon ? two integers that fit in 16-bit integer type, separated by a single space. Following the row with the co-ordinates of the last vertex for the task comes the line with the number of vertices for the next test and so on.
Output
For each test you must write on one line the required surface - a number with exactly two digits after the decimal point (the number should be rounded to the second digit after the decimal point).
Sample Input
1 7 0 0 4 4 4 7 9 7 13 -1 8 -6 4 -4
Sample Output
80.00
题意:求多边形的核
定义-多边形的核:多边形的核平面简单多边形的核是该多边形内部的一个点集,该点集中任意一点与多边形边界上一点的连线都处于这个多,判断方法是用多边形所有的边切割原来的多边形,得到的新区域就是多边形的核。
思路:半平面交,这题默认输入是顺时针。
代码:
View Code#include<stdio.h>
#include<math.h>
#include<map>
#include<iostream>
#include<string.h>
using namespace std;
const int N = 1505;
const double eps = 1e-8;
const double PI = acos(-1.0);
int sgn(double x)
{
if(fabs(x) < eps)return 0;
if(x < 0)return -1;
else return 1;
}
struct Point
{
double x,y;
Point() {}
Point(double _x,double _y)
{
x = _x;
y = _y;
}
Point operator -(const Point &b)const
{
return Point(x - b.x,y - b.y);
}
double operator ^(const Point &b)const
{
return x*b.y - y*b.x;
}
double operator *(const Point &b)const
{
return x*b.x + y*b.y;
}
};
//计算多边形面积
double CalcArea(Point p[],int n)
{
double res = 0;
for(int i = 0; i < n; i++)
res += (p[i]^p[(i+1)%n]);
return fabs(res/2);
}
//通过两点,确定直线方程
void Get_equation(Point p1,Point p2,double &a,double &b,double &c)
{
a = p2.y - p1.y;
b = p1.x - p2.x;
c = p2.x*p1.y - p1.x*p2.y;
}
//求交点
Point Intersection(Point p1,Point p2,double a,double b,double c)
{
double u = fabs(a*p1.x + b*p1.y + c);
double v = fabs(a*p2.x + b*p2.y + c);
Point t;
t.x = (p1.x*v + p2.x*u)/(u+v);
t.y = (p1.y*v + p2.y*u)/(u+v);
return t;
}
Point tp[N],p[N],q[N];
void Cut(double a,double b,double c,Point p[],int &cnt)
{
int tmp = 0;
for(int i = 1; i <= cnt; i++)
{
//当前点在左侧,逆时针的点
if(a*p[i].x + b*p[i].y + c < eps)tp[++tmp] = p[i];
else
{
if(a*p[i-1].x + b*p[i-1].y + c < -eps)//如果p[i-1]在直线的左侧的话,
//则将p[i],p[i-1]形成的直线与已知直线的交点作为核的一个顶点(这样的话,由于精度的问题,核的面积可能会有所减少)
tp[++tmp] = Intersection(p[i-1],p[i],a,b,c);
if(a*p[i+1].x + b*p[i+1].y + c < -eps)
tp[++tmp] = Intersection(p[i],p[i+1],a,b,c);
}
}
for(int i = 1; i <= tmp; i++)
p[i] = tp[i];
p[0] = p[tmp];
p[tmp+1] = p[1];
cnt = tmp;
}
void solve(Point p[],int n)
{
p[n+1]=p[1];
int cnt=n;
for(int i=0;i<=n+1;i++) q[i]=p[i];
for(int i=1; i<=n; i++)
{
double a,b,c;
Get_equation(q[i+1],q[i],a,b,c);//这里由于原题输入顺时针,我模版是逆时针处理的,就交换了q[i+1],q[i]的位置
Cut(a,b,c,p,cnt);
}
printf("%.2lf\n",CalcArea(p,cnt));
}
int main()
{
//freopen("in.txt","r",stdin);
int t,n;
scanf("%d",&t);
while(t--)
{
scanf("%d",&n);
for(int i=1; i<=n; i++)
{
scanf("%lf%lf",&p[i].x,&p[i].y);
}
solve(p,n);
}
}






