B. Mr. Kitayuta's Colorful Graph

 B. Mr. Kitayuta's Colorful Graph

 time limit per test

 1 second

Mr. Kitayuta has just bought an undirected graph consisting of n vertices and m edges. The vertices of the graph are numbered from 1 to n. Each edge, namely edge i, has a color c_i, connecting vertex a_i and b_i.

Mr. Kitayuta wants you to process the following q queries.

In the i-th query, he gives you two integers — u_i and v_i.

Find the number of the colors that satisfy the following condition: the edges of that color connect vertex u_i and vertex v_i directly or indirectly.

Input

The first line of the input contains space-separated two integers — n and m (2 ≤ n ≤ 100, 1 ≤ m ≤ 100), denoting the number of the vertices and the number of the edges, respectively.

The next m lines contain space-separated three integers — a_i, b_i (1 ≤ a_i < b_i ≤ n) and c_i (1 ≤ c_i ≤ m). Note that there can be multiple edges between two vertices. However, there are no multiple edges of the same color between two vertices, that is, if i ≠ j, (a_i, b_i, c_i) ≠ (a_j, b_j, c_j).

The next line contains a integer — q (1 ≤ q ≤ 100), denoting the number of the queries.

Then follows q lines, containing space-separated two integers — u_i and v_i (1 ≤ u_i, v_i ≤ n). It is guaranteed that u_i ≠ v_i.

Output

For each query, print the answer in a separate line.

Sample test(s)

Input

4 5
1 2 1
1 2 2
2 3 1
2 3 3
2 4 3
3
1 2
3 4
1 4

Output

2
1
0

Input

5 7
1 5 1
2 5 1
3 5 1
4 5 1
1 2 2
2 3 2
3 4 2
5
1 5
5 1
2 5
1 5
1 4

Output

1
1
1
1
2

Note

Let's consider the first sample.

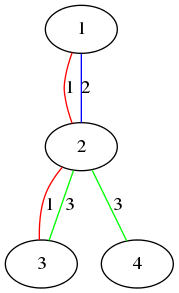The figure above shows the first sample.

• Vertex 1 and vertex 2 are connected by color 1 and 2.
• Vertex 3 and vertex 4 are connected by color 3.
• Vertex 1 and vertex 4 are not connected by any single color.

 

#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
#include <cmath>
#include <map>
#include <set>
#include <stack>
#include <queue>
#include <string>
#include <vector>
using namespace std;
const double EXP=1e-8;
const double PI=acos(-1.0);
const int INF=0x7fffffff;
const int MS=105;

int fa[MS][MS];
void init()
{
    for(int i=0;i<MS;i++)
        for(int j=0;j<MS;j++)
            fa[i][j]=j;
}
int find(int c,int a)
{
    if(fa[c][a]==a)
        return a;
    return fa[c][a]=find(c,fa[c][a]);
}
void make_union(int c,int a,int b)
{
    a=find(c,a);
    b=find(c,b);
    if(a==b)
        return ;
    fa[c][b]=fa[c][a];
}
int main()
{
    int n,m,q,a,b,c;
    init();
    cin>>n>>m;
    for(int i=0;i<m;i++)
    {
        cin>>a>>b>>c;
        make_union(c,a,b);
    }
    cin>>q;
    while(q--)
    {
        int ans=0;
        cin>>a>>b;
        for(c=1;c<=m;c++)
            if(find(c,a)==find(c,b))
                ans++;
        cout<<ans<<endl;
    }
    return 0;
}