RSA总结 from La佬
心血来潮想整理一下RSA来着,忽然想到la佬的这篇就当收藏了,la佬勿怪qaq!

常用工具
-
分解大素数
factordb (http://www.factordb.com)
yafu(p,q相差过大或过小yafu可分解成功)
sage (divisors(n))(小素数)
Pollard’s p−1 (python -m primefac -vs -m=p-1 xxxxxxx)(光滑数)
Williams’s p+1(python -m primefac -vs -m=p+1 xxxxxxx)(光滑数) -
在线sage环境:https://sagecell.sagemath.org/
-
Openssl
解析加密密钥:openssl rsa -pubin -text -modulus -in pub.key生成解密密钥:
python rsatool.py -f PEM -o key.key -p 1 -q 1 -e 1 openssl rsautl -decrypt -inkey key.pem -in flag.enc -out flag openssl rsautl -decrypt -oaep -inkey key.pem -in flag.enc -out flag (OAEP方式)脚本生成解密密钥:
# coding=utf-8 import math import sys from Crypto.PublicKey import RSA keypair = RSA.generate(1024) keypair.p = keypair.q = keypair.e = keypair.n = keypair.p * keypair.q Qn = long((keypair.p - 1) * (keypair.q - 1)) i = 1 while (True): x = (Qn * i) + 1 if (x % keypair.e == 0): keypair.d = x / keypair.e break i += 1 private = open('private.pem', 'w') private.write(keypair.exportKey()) private.close() -
脚本集
-
https://github.com/Ganapati/RsaCtfTool
#用法一:已知公钥(自动求私钥) $ python3 RsaCtfTool.py --publickey 公钥文件 --uncipherfile 加密文件 #用法二:已知公钥求私钥 $ python3 RsaCtfTool.py --publickey 公钥文件 --private #用法三:密钥格式转换 #把PEM格式的公钥转换为n,e $ python3 RsaCtfTool.py --dumpkey --key 公钥文件 #把n,e转换为PEM格式 $ python3 RsaCtfTool.py --createpub -n 782837482376192871287312987398172312837182 -e 65537
-
常见类型
给p,q,e,c
import gmpy2 as gp
import binascii
p =
q =
e =
c =
n = p*q
phi = (p-1)*(q-1)
d = gp.invert(e,phi)
m = pow(c,d,n)
print(m)
print(bytes.fromhex(hex(m)[2:]))
给n,e,dp,c
\(dp\equiv d \pmod {(p-1)}\)
\(\because dp\cdot e\equiv d\cdot e\equiv 1 \pmod {(p-1)}\)
\(\therefore dp\cdot e-1=k\cdot (p-1)\)
\(\therefore (dp\cdot e-1)\cdot d\cdot e=k’\cdot (p-1),\quad k’=k\cdot d\cdot e \\\Leftrightarrow d\cdot e=-k’\cdot (p-1)+dp\cdot e\cdot d\cdot e\equiv 1 \pmod{\varphi(n)}\\\Leftrightarrow -k’\cdot (p-1)+dp\cdot e\equiv 1\pmod{\varphi(n)}\)
\(\therefore k_{1}\cdot (p-1)+dp\cdot e-1=k_{2}\cdot (p-1)\cdot (q-1)\\\Leftrightarrow (p-1)\cdot (k_{2}\cdot (q-1)-k_{1})+1=dp\cdot e\)
\(\because dp<p-1\quad \therefore (k_{2}\cdot (q-1)-k_{1})\in (0, e)\)
therefore 遍历 (\(1, e\)),当同时满足 \((dp\cdot e-1)\bmod i==0\) 和 \(n\bmod((dp\cdot e-1)//i+1)==0\) 时,\(N\) 成功分解。
import gmpy2 as gp
e =
n =
dp =
c =
for x in range(1, e):
if(e*dp%x==1):
p=(e*dp-1)//x+1
if(n%p!=0):
continue
q=n//p
phin=(p-1)*(q-1)
d=gp.invert(e, phin)
m=gp.powmod(c, d, n)
if(len(hex(m)[2:])%2==1):
continue
print('--------------')
print(m)
print(hex(m)[2:])
print(bytes.fromhex(hex(m)[2:]))
-
变种1:给 \(p,e,d_p,c,b\) ,其中 \(n=p^bq\) 。
Hensel lifting for Takagi’s scheme(p.189):
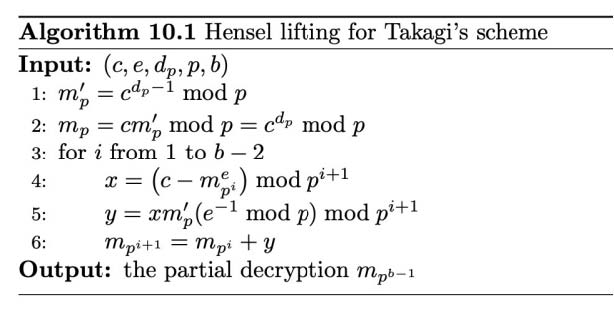
from Crypto.Util.number import * import gmpy2 p = dp = c = b = e = mp1 = pow(c, dp, p) mp = pow(c, dp - 1, p) for i in range(1, b - 2): x = pow(c - pow(mp1, e), 1, p**(i + 1)) y = pow(x * mp * (gmpy2.invert(e, p)), 1, p**(i + 1)) mp1 = mp1 + y print(long_to_bytes(mp1)) -
变种2:给 \(n,e,dp_0,c,k\),其中 \(dp_0\) 为 \(dp\) 高 \((n\text{bits}-k)\) 位,即 \(dp_0=dp>>k\)。
(Coppersmith攻击,已知dp高位攻击)\(e\cdot dp \equiv e\cdot d\equiv 1 \pmod {(p-1)} \\\Leftrightarrow e \cdot dp=k(p-1)+1=kp-k+1 \\\Leftrightarrow e\cdot dp+k-1 \equiv 0 \pmod p\)
\(\because dp<p-1\),\(\therefore k<e\)
\(\therefore e\cdot (dp_0<<k+x)+k-1 \equiv 0 \pmod p\)#Sage dp0 = e = n = F.<x> = PolynomialRing(Zmod(n)) d = inverse_mod(e, n) for k in range(1, e): f = (secret << 200) + x + (k - 1) * d x0 = f.small_roots(X=2 ** (200 + 1), beta=0.44, epsilon=1/32) if len(x0) != 0: dp = x0[0] + (secret << 200) for i in range(2, e): p = (e * Integer(dp) - 1 + i) // i if n % p == 0: break if p < 0: continue else: print('k = ',k) print('p = ',p) print('dp = ',dp) break -
变种3:给 \(n,e,dp,c\),其中 \(dp\)很小,\(e\) 很大。
枚举 \(dp\),因 \(e\cdot dp \equiv 1 \pmod {(p-1)}\)
又由费马小定理,对任意 \(r\),有 \(m^{e \cdot dp}\equiv m \pmod p\)
即 \(p \mid (m^{e \cdot dp}-m)\) 又 \(p \mid n\)很大概率 \(p=\gcd(m^{e \cdot dp}-m,n)\)
给p,q,dp,dq,c
\(dp=d \bmod (p-1),dq=d \bmod (q-1)\)
\(\because d=k_{1}(p-1)+dp=k_{2}(q-1)+dq\\\Leftrightarrow k_{1}(p-1)=(dq-dp)+k_{2}(q-1)\\\Leftrightarrow k_{1}\frac{p-1}{\gcd(p-1,q-1)}=\frac{dq-dp}{\gcd(p-1,q-1)}+k_{2}\frac{q-1}{\gcd(p-1,q-1)}\\\Rightarrow k_{1}\frac{p-1}{\gcd(p-1,q-1)}\equiv\frac{dq-dp}{\gcd(p-1,q-1)} \pmod {\frac{q-1}{\gcd(p-1,q-1)}}\\\Leftrightarrow k_{1}\equiv \text{inv}(\frac{p-1}{\gcd(p-1,q-1)},\frac{q-1}{\gcd(p-1,q-1)})\cdot \frac{dq-dp}{\gcd(p-1,q-1)} \pmod {\frac{q-1}{\gcd(p-1,q-1)}}\)
将 \(k_{1}=k_{3}\frac{q-1}{\gcd(p-1,q-1)}+\text{inv}(\frac{p-1}{\gcd(p-1,q-1)},\frac{q-1}{\gcd(p-1,q-1)})\cdot \frac{dq-dp}{\gcd(p-1,q-1)}\) 代入 \(d=k_{1}(p-1)+dp\)
\(d=k_{3}\frac{(p-1)(q-1)}{\gcd(p-1,q-1)}+\text{inv}(\frac{p-1}{\gcd(p-1,q-1)},\frac{q-1}{\gcd(p-1,q-1)})\cdot \frac{(dq-dp)(p-1)}{\gcd(p-1,q-1)}+dp\\\Rightarrow d\equiv \text{inv}(\frac{p-1}{\gcd(p-1,q-1)},\frac{q-1}{\gcd(p-1,q-1)})\cdot \frac{(dq-dp)(p-1)}{\gcd(p-1,q-1)}+dp \pmod{\frac{(p-1)(q-1)}{\gcd(p-1,q-1)}}\)
import gmpy2 as gp
p =
q =
dp =
dq =
c =
n = p*q
phin = (p-1)*(q-1)
dd = gp.gcd(p-1, q-1)
d=(dp-dq)//dd * gp.invert((q-1)//dd, (p-1)//dd) * (q-1) +dq
print(d)
m = gp.powmod(c, d, n)
print('-------------------')
print(m)
print(hex(m)[2:])
print(bytes.fromhex(hex(m)[2:]))
低解密指数攻击/低私钥指数攻击(e长度较大,d小,Wiener Attack)
适用情况:已知 \(N,e\) ,且 \(e\) 过大或过小。
\(\varphi(n) = (p-1)(q-1)=pq - (p + q) + 1=N - (p + q) + 1\)
\(\because p, q 非常大\) ,
\(\therefore\,pq\gg p+q\) ,
\(\therefore\varphi(n)\approx N\) ,
\(\because ed\equiv1\,mod\,\varphi(n)\) ,
\(\therefore ed-1=k\varphi(n),这个式子两边同除\) ,
\(d\varphi(n)\) 可得:
\(\cfrac{e}{\varphi(n)}-\cfrac{k}{d}=\cfrac{1}{d\varphi(n)}\)
\(\because \varphi(n)\approx N,\\\therefore \cfrac{e}{N}-\cfrac{k}{d}=\cfrac{1}{d\varphi(n)}\) ,
同样 \(d\varphi(n)\) 是一个很大的数,所以 \(\cfrac{e}{N}\) 略大于 \(\cfrac{k}{d}\)
因为 \(e\) 和 \(N\) 是知道的,所以计算出 \(\cfrac{e}{N}\) 后,比它略小的 \(\cfrac{k}{d}\) ,可以通过计算 \(\cfrac{e}{N}\) 的连分数展开,依次算出这个分数每一个渐进分数,由于 \(\cfrac{e}{N}\) 略大于 \(\cfrac{k}{d}\) ,Wiener 证明了,该攻击能精确的覆盖 \(\cfrac{k}{d}\)
在 \(e\) 过大或过小的情况下,可使用算法从 \(e\) 中快速推断出 \(d\) 的值。可以解决 \(q<p<2q,d<\cfrac{1}{3}N^{\frac{1}{4}}\) 的问题。
RSAWienerHacker工具:https://github.com/pablocelayes/rsa-wiener-attack
#脚本1(带工具)
#python2
import RSAwienerHacker
n =
e =
d = RSAwienerHacker.hack_RSA(e,n)
if d:
print(d)
import hashlib
flag = "flag{" + hashlib.md5(hex(d)).hexdigest() + "}"
print flag
#脚本2
#sage
def rational_to_contfrac(x,y):
# Converts a rational x/y fraction into a list of partial quotients [a0, ..., an]
a = x // y
pquotients = [a]
while a * y != x:
x, y = y, x - a * y
a = x // y
pquotients.append(a)
return pquotients
def convergents_from_contfrac(frac):
# computes the list of convergents using the list of partial quotients
convs = [];
for i in range(len(frac)): convs.append(contfrac_to_rational(frac[0 : i]))
return convs
def contfrac_to_rational (frac):
# Converts a finite continued fraction [a0, ..., an] to an x/y rational.
if len(frac) == 0: return (0,1)
num = frac[-1]
denom = 1
for _ in range(-2, -len(frac) - 1, -1): num, denom = frac[_] * num + denom, num
return (num, denom)
n =
e =
c =
def egcd(a, b):
if a == 0: return (b, 0, 1)
g, x, y = egcd(b % a, a)
return (g, y - (b // a) * x, x)
def mod_inv(a, m):
g, x, _ = egcd(a, m)
return (x + m) % m
def isqrt(n):
x = n
y = (x + 1) // 2
while y < x:
x = y
y = (x + n // x) // 2
return x
def crack_rsa(e, n):
frac = rational_to_contfrac(e, n)
convergents = convergents_from_contfrac(frac)
for (k, d) in convergents:
if k != 0 and (e * d - 1) % k == 0:
phi = (e * d - 1) // k
s = n - phi + 1
# check if x*x - s*x + n = 0 has integer roots
D = s * s - 4 * n
if D >= 0:
sq = isqrt(D)
if sq * sq == D and (s + sq) % 2 == 0: return d
d = crack_rsa(e, n)
m = hex(pow(c, d, n))[2:]
print(bytes.fromhex(m))
#脚本3
from Crypto.Util.number import long_to_bytes
e =
n =
c =
#将分数x/y展开为连分数的形式
def transform(x,y):
arr=[]
while y:
arr+=[x//y]
x,y=y,x%y
return arr
#求解渐进分数
def sub_fraction(k):
x=0
y=1
for i in k[::-1]:
x,y=y,x+i*y
return (y,x)
data=transform(e,n)
for x in range(1,len(data)+1):
data1=data[:x]
d = sub_fraction(data1)[1]
m = pow(c,d,n)
flag = long_to_bytes(m)
if b'flag{' in flag:
print(flag)
break
-
变种1:
\(\cfrac{N_1}{N_2}<\cfrac{q_1}{q_2}<1\)参考:2020年羊城杯 - RRRRRRRSA
Paper: https://eprint.iacr.org/2015/399.pdf尝试对 \(\cfrac{N_1}{N_2}\) 进行连分数展开并求其各项渐进分数,记为 \(\cfrac{t_i}{s_i}\) 并验证 \(N_1\% {t_k}==0\)
是否成立,如果成立,那么 \(q_1=t_k,q_2=s_k\)
连分数逼近:
def transform(x,y): #使用辗转相除将分数x/y转为连分数的形式
res=[]
while y:
res.append(x//y)
x,y=y,x%y
return res
def continued_fraction(sub_res):
numerator,denominator=1,0
for i in sub_res[::-1]: #从sublist的后面往前循环
denominator,numerator=numerator,i*numerator+denominator
return denominator,numerator #得到渐进分数的分母和分子,并返回
#求解每个渐进分数
def sub_fraction(x,y):
res=transform(x,y)
res=list(map(continued_fraction,(res[0:i] for i in range(1,len(res))))) #将连分数的结果逐一截取以求渐进分数
return res
def wienerAttack(n1,n2):
for (q2,q1) in sub_fraction(n1,n2): #用一个for循环来注意试探n1/n2的连续函数的渐进分数,直到找到一个满足条件的渐进分数
if q1==0: #可能会出现连分数的第一个为0的情况,排除
continue
if n1%q1==0 and q1!=1: #成立条件
return (q1,q2)
print("该方法不适用")
N1=60143104944034567859993561862949071559877219267755259679749062284763163484947626697494729046430386559610613113754453726683312513915610558734802079868190554644983911078936369464590301246394586190666760362763580192139772729890492729488892169933099057105842090125200369295070365451134781912223048179092058016446222199742919885472867511334714233086339832790286482634562102936600597781342756061479024744312357407750731307860842457299116947352106025529309727703385914891200109853084742321655388368371397596144557614128458065859276522963419738435137978069417053712567764148183279165963454266011754149684758060746773409666706463583389316772088889398359242197165140562147489286818190852679930372669254697353483887004105934649944725189954685412228899457155711301864163839538810653626724347
N2=60143104944034567859993561862949071559877219267755259679749062284763163484947626697494729046430386559610613113754453726683312513915610558734802079868195633647431732875392121458684331843306730889424418620069322578265236351407591029338519809538995249896905137642342435659572917714183543305243715664380787797562011006398730320980994747939791561885622949912698246701769321430325902912003041678774440704056597862093530981040696872522868921139041247362592257285423948870944137019745161211585845927019259709501237550818918272189606436413992759328318871765171844153527424347985462767028135376552302463861324408178183842139330244906606776359050482977256728910278687996106152971028878653123533559760167711270265171441623056873903669918694259043580017081671349232051870716493557434517579121
print(wienerAttack(N1,N2))
低加密指数广播攻击(Hastad攻击)
适用情况: \(n,c\) 不同,\(m,e\) 相同。一般会是 \(e=k\) ,然后给 \(k\) 组数据。
如果一个用户使用同一个加密指数 \(e\) 加密了同一个密文,并发送给了其他 \(e\) 个用户。那么就会产生广播攻击。这一攻击由 Håstad 提出。
使用不同的模数 \(n\),相同的公钥指数 \(e\) 加密相同的信息,就会得到多个 \(m^e \equiv c_i \pmod {n_i}\)
将 \(m (e)\) 视为一个整体 \(M\),这就是典型的中国剩余定理适用情况。容易求得 \(m (e)\) 的值,当 \(e\) 较小时直接开 \(e\) 方即可,可使用 \(gmpy2.iroot(M,e)\) 方法。
更一般情况(\(k\) 组数据的 \(N\) 不同)见15。
#sage
def chinese_remainder(modulus, remainders):
Sum = 0
prod = reduce(lambda a, b: a*b, modulus)
for m_i, r_i in zip(modulus, remainders):
p = prod // m_i
Sum += r_i * (inverse_mod(p,m_i)*p)
return Sum % prod
chinese_remainder([3,5,7],[2,3,2]) #23
#sage
crt([2,3,2],[3,5,7])
共模攻击(\(n,m\)相同,\(c,e\)不同)
当\(n\)不变的情况下,知道\(n,e_1,e_2,c_1,c_2\)可以在不知道\(d_1,d_2\)的情况下,解出\(m\) 。
首先假设 \(e_1,e_2\) 互质,即 \(\gcd(e_1,e_2)=1\) 此时则有 \(e_1s_1+e_2s_2 = 1\) 式中,\(s_1,s_2\) 皆为整数,但是一正一负。
通过扩展欧几里德算法,我们可以得到该式子的一组解(\(s_1,s_2\)),假设\(s_1\)为正数,\(s_2\)为负数。
因为 \(c_1 = m^{e_1}\bmod n, c_2 = m^{e_2}\bmod n\)
所以 \((c_1^{s_1}c_2^{s_2})\bmod n = ((m^{e_1}\bmod n)^{s_1}(m^{e_2}\bmod n)^{s_2})\bmod n\)
根据模运算性质,可以化简为 \((c_1^{s_1}c_2^{s_2})\bmod n = ((m^{e_1})^{s_1}(m^{e_2})^{s_2})\bmod n\)
即 \((c_1^{s_1}c_2^{s_2})\bmod n = (m^{e_1s_1+e_2s_2})\bmod n\)
又前面提到 \(e_1s_1+e_2s_2 = 1\)
所以 \((c_1^{s_1}c_2^{s_2})\bmod n = m\bmod n\)
即 \(c_1^{s_1}c_2^{s_2}= m\)
import gmpy2 as gp
def egcd(a, b):
if a == 0:
return (b, 0, 1)
else:
g, y, x = egcd(b % a, a)
return (g, x - (b // a) * y, y)
n =
c1 =
c2 =
e1 =
e2 =
s = egcd(e1, e2)
s1 = s[1]
s2 = s[2]
if s1<0:
s1 = - s1
c1 = gp.invert(c1, n)
elif s2<0:
s2 = - s2
c2 = gp.invert(c2, n)
m = pow(c1,s1,n)*pow(c2,s2,n) % n
print(hex(m)[2:])
print(bytes.fromhex(hex(m)[2:]))
e,m相同,多个n中存在两个n有GCD(模不互素)
适用情况:存在两个或更多模数 ,且 \(\gcd(n_1,n_2)\ne 1\)
多个模数 \(n\) 共用质数,则可以很容易利用欧几里得算法求得他们的质因数之一 \(\gcd(n_1,n_2)\)
然后这个最大公约数可用于分解模数分别得到对应的 \(p\) 和 \(q\),即可进行解密。
import gmpy2 as gp
n=[]
for i in n:
for j in n:
if (i<>j):
pub_p=gp.gcdext(i,j)
if (pub_p[0]<>1)&(i>j):
print(i)
print(j)
print(pub_p[0])
a=i,p=pub_p[0]
q=a//p
p =
q =
e =
c =
n = p*q
phi = (p-1) * (q-1)
d = gp.invert(e, phi)
m = pow(c, d, n)
print(hex(m)[2:])
print(bytes.fromhex(hex(m)[2:]))
Rabin加密
适用情况:\(e=2\)。
一般先通过其他方法分解得到 \(p,q\) ,然后解密。
函数返回四个数,这其中只有一个是我们想要的明文,需要通过其他方式验证。
import gmpy2
def rabin_decrypt(c, p, q, e=2):
n = p * q
mp = pow(c, (p + 1) // 4, p)
mq = pow(c, (q + 1) // 4, q)
yp = gmpy2.invert(p, q)
yq = gmpy2.invert(q, p)
r = (yp * p * mq + yq * q * mp) % n
rr = n - r
s = (yp * p * mq - yq * q * mp) % n
ss = n - s
return (r, rr, s, ss)
c =
p =
q =
m = rabin_decrypt(c,p,q)
for i in range(4):
try:
print(bytes.fromhex(hex(m[i])[2:]))
except:
pass
Boneh and Durfee attack
\(e\) 非常大接近于\(N\) ,即 \(d\) 较小时。与低解密指数攻击类似,比低解密指数攻击(Wiener Attack)更强,可以解决 \(\cfrac{1}{3}N^{\frac{1}{4}} \leq d \leq N^{0.292}\) 的问题。
参考 https://github.com/mimoo/RSA-and-LLL-attacks 。
2k [(N + 1)/2 + (-p -q)/2] + 1 = 0 mod e
Boneh and Durfee attack:
f(x,y) = 1 + x * (A + y)
e d = x [(N + 1)/2 + y] + 1
故:
x = 2k
y = (-p-q)/2
Coppersmith定理指出在一个 \(e\) 阶的 \(\bmod n\) 多项式 \(f(x)\) 中,如果有一个根小于 \(n^{\frac{1}{e}}\),就可以运用一个 \(O(\log n)\) 的算法求出这些根。
Coppersmith攻击(已知p的高位攻击)
知道 \(p\) 的高位为 \(p\) 的位数的约 \(\frac12\) 时即可。
#Sage
from sage.all import *
n =
p4 =
#p去0的剩余位
e =
pbits = 1024
kbits = pbits - p4.nbits()
print(p4.nbits())
p4 = p4 << kbits
PR.<x> = PolynomialRing(Zmod(n))
f = x + p4
roots = f.small_roots(X=2^kbits, beta=0.4)
#经过以上一些函数处理后,n和p已经被转化为10进制
if roots:
p = p4+int(roots[0])
print("n: "+str(n))
print("p: "+str(p))
print("q: "+str(n//p))
Coppersmith攻击(已知m的高位攻击)
这里我们假设我们首先加密了消息 \(m\) ,如下
\(C\equiv m^e \bmod N\)
并且我们假设我们知道消息 \(m\) 的很大的一部分 \(m_0\),即 \(m=m_0+x\) ,但是我们不知道 \(x\)。那么我们就有可能通过该方法进行恢复消息。这里我们不知道的 \(x\) 其实就是多项式的根,需要满足 Coppersmith 的约束。
可以参考 https://github.com/mimoo/RSA-and-LLL-attacks 。
\(e\) 足够小,且部分明文泄露时,可以采用Coppersmith单变量模等式的攻击,如下:
\(c=m^{e}\bmod n=(mbar+x_{0})^{e}\bmod n\)
其中 \(mbar = (m >> k\text{bits}) << k\text{bits}\)
当 \(\vert x_{0}\vert\leq N^{\frac{1}{e}}\) 时,可以在 log N 和 e 的多项式时间内求出 \(x_0\) 。
#Sage
n =
e =
c =
mbar =
kbits =
beta = 1
nbits = n.nbits()
print("upper {} bits of {} bits is given".format(nbits - kbits, nbits))
PR.<x> = PolynomialRing(Zmod(n))
f = (mbar + x)^e - c
x0 = f.small_roots(X=2^kbits, beta=1)[0] # find root < 2^kbits with factor = n
print("m:", mbar + x0)
Coppersmith攻击(已知d的低位攻击)
如果知道 \(d\) 的低位,低位约为 \(n\) 的位数的 \(\frac14 (\frac{n.n\text{bits}()}{4})\) 就可以恢复 \(d\)。
#Sage
def partial_p(p0, kbits, n):
PR.<x> = PolynomialRing(Zmod(n))
nbits = n.nbits()
f = 2^kbits*x + p0
f = f.monic()
roots = f.small_roots(X=2^(nbits//2-kbits), beta=0.4) # find root < 2^(nbits//2-kbits) with factor >= n^0.4
if roots:
x0 = roots[0]
p = gcd(2^kbits*x0 + p0, n)
return ZZ(p)
def find_p(d0, kbits, e, n):
X = var('X')
for k in range(1, e+1):
results = solve_mod([e*d0*X - k*X*(n-X+1) + k*n == X], 2^kbits)
for x in results:
p0 = ZZ(x[0])
p = partial_p(p0, kbits, n)
if p and p != 1:
return p
if __name__ == '__main__':
n =
e =
c =
d0 =
beta = 0.5
nbits = n.nbits()
kbits = d0.nbits()
print("lower %d bits (of %d bits) is given" % (kbits, nbits))
p = int(find_p(d0, kbits, e, n))
print("found p: %d" % p)
q = n//int(p)
print("d:", inverse_mod(e, (p-1)*(q-1)))
-
变种1:
\(n=p\cdot q\cdot r\)
已知 \(n,p,d=\text{inv}(e,\varphi(n)),e,c\)\(k(p-1)\rightarrow k’,qr\rightarrow n’,q+r\rightarrow s\)
\(ed_{0}\equiv 1+k’(n’-s+1) \pmod {2^{d_{0}.n\text{bits}()}}\quad (1)\)
\(q^{2}-sq+n’\equiv 0 \pmod {2^{d_{0}.n\text{bits}()}}\quad (2)\)
联立可得
\((ed_{0}-1-k’n’-k’)q+k’q^{2}+k’n’\equiv 0 \pmod {2^{d_{0}.n\text{bits}()}}\)即求解同余方程可得 \(q\) 的低 \(size(d0)\) 位,本来是个partial d的coppersmith问题,但因为step1求解同余方程后得到的 \(q\) 已是完整的 \(q\) ,所以无需后续的coppersmith。
参考:Dragon CTF 2019 - RSA Chained
#Sage def find_p(d0, kbits, e, n, p): X = var('X') for k in range(1, e + 1): k_dot = k * (p - 1) results = solve_mod([e * d0 * X - k_dot * X * (n - X + 1) + k_dot * n == X], 2^kbits) for x in results: q = ZZ(x[0]) if n % q == 0: return q return None n = ... # q * r p = c = d0 = e = kbits = d0.nbits() q = find_p(d0, kbits, e, n, p) phi = (p - 1) * (q - 1) * (n // q - 1) d = inverse_mod(e, phi) print(bytes.fromhex(hex(pow(c, d, p * n))[2:]))
Coppersmith攻击(已知N一个因子的高位,部分p)
当我们知道一个公钥中模数 \(N\) 的一个因子的较高位时,我们就有一定几率来分解 \(N\) 。
参考 https://github.com/mimoo/RSA-and-LLL-attacks 。
关注下面的代码:
beta = 0.5
dd = f.degree()
epsilon = beta / 7
mm = ceil(beta**2 / (dd * epsilon))
tt = floor(dd * mm * ((1/beta) - 1))
XX = ceil(N**((beta**2/dd) - epsilon)) + 1000000000000000000000000000000000
roots = coppersmith_howgrave_univariate(f, N, beta, mm, tt, XX)
其中,
-
必须满足
\(q\ge N^{beta}\)
所以这里给出了 \(beta=0.5\),显然两个因数中必然有一个是大于的。
-
XX 是 \(f(x)=q′+x\) 在模 \(q\) 意义下的根的上界,自然我们可以选择调整它,这里其实也表明了我们已知的 \(q′\) 与因数 \(q\) 之间可能的差距
#Sage
n =
e =
c =
pbar =
kbits =
print("upper %d bits (of %d bits) is given" % (pbar.nbits()-kbits, pbar.nbits()))
PR.<x> = PolynomialRing(Zmod(n))
f = x + pbar
x0 = f.small_roots(X=2^kbits, beta=0.4)[0] # find root < 2^kbits with factor >= n^0.4
p = x0 + pbar
print("p:", p)
q = n // int(p)
d = inverse_mod(e, (p-1)*(q-1))
print("m:", pow(c, d, n))
注:
sage的small_root传参 \(X\) 不能过大,需自行判断阈值并调整(如果 \(X\) 过大,即使存在 \(X\) 内的解,也无法求出);
比如 \(p\) 的低位泄露时因为不确定缺失高位的具体比特数,所以要在 \(2^{\frac{n.n\text{bits}()}{2}−k\text{bits}}\) 附近作X的阈值估计;
无法确定拿到的 \(p\) 是否大于 \(q\),所以对 \(\beta=0.5\)
进行调整至 \(0.4\) 。
Coppersmith’s Short-pad Attack & Related Message Attack(Franklin-Reiter攻击)
目前在大部分消息加密之前都会进行 padding,但是如果 padding 的长度过短
\(m \in (0,\lfloor\frac{n.n\text{bits}()}{e^2}\rfloor]\)
也有可能被很容易地攻击。
这里所谓 padding 过短,其实就是对应的多项式的根会过小。
当 Alice 使用同一公钥对两个具有某种线性关系的消息 \(M_1\) 与 \(M_2\) 进行加密,并将加密后的消息 \(C_1,C_2\) 发送给了 Bob 时,我们就可能可以获得对应的消息 \(M_1\) 与 \(M_2\) 。这里我们假设模数为 \(N\) ,两者之间的线性关系如下:
\(M_1 \equiv f(M_2) \bmod N\)
其中 \(f\) 为一个线性函数,比如说 \(f=ax+b\) 。
在具有较小错误概率下的情况下,其复杂度为 \(O(e\log^2N)\)
这一攻击由 Franklin与Reiter 提出。
#脚本1
#Sage
import binascii
def attack(c1, c2, n, e):
PR.<x>=PolynomialRing(Zmod(n))
# replace a,b,c,d
g1 = (a*x+b)^e - c1
g2 = (c*x+d)^e - c2
def gcd(g1, g2):
while g2:
g1, g2 = g2, g1 % g2
return g1.monic()
return -gcd(g1, g2)[0]
c1 =
c2 =
n =
e =
m1 = attack(c1, c2, n, e)
print(binascii.unhexlify("%x" % int(m1)))
#脚本2
#Sage
def short_pad_attack(c1, c2, e, n):
PRxy.<x,y> = PolynomialRing(Zmod(n))
PRx.<xn> = PolynomialRing(Zmod(n))
PRZZ.<xz,yz> = PolynomialRing(Zmod(n))
g1 = x^e - c1
g2 = (x+y)^e - c2
q1 = g1.change_ring(PRZZ)
q2 = g2.change_ring(PRZZ)
h = q2.resultant(q1)
h = h.univariate_polynomial()
h = h.change_ring(PRx).subs(y=xn)
h = h.monic()
kbits = n.nbits()//(2*e*e)
diff = h.small_roots(X=2^kbits, beta=0.4)[0] # find root < 2^kbits with factor >= n^0.4
return diff
def related_message_attack(c1, c2, diff, e, n):
PRx.<x> = PolynomialRing(Zmod(n))
g1 = x^e - c1
g2 = (x+diff)^e - c2
def gcd(g1, g2):
while g2:
g1, g2 = g2, g1 % g2
return g1.monic()
return -gcd(g1, g2)[0]
if __name__ == '__main__':
n =
e =
c1 =
c2 =
diff = short_pad_attack(c1, c2, e, n)
print("difference of two messages is %d" % diff)
m1 = related_message_attack(c1, c2, diff, e, n)
print("m1:", m1)
print("m2:", m1 + diff)
RSA Hastad Attack with non-linear padding and different public keys(带非线性padding和不同公钥的广播攻击)
适用情况:\(m\) 经 \(k\) 次非线性padding处理后,分别用 \(k\) 组 (\(N_i,e_i\)) 加密后得 \(k\) 组 \(c_i\)。
参考:2020年羊城杯 - Invitation
#Sage
#e=3, padding: m²+(3^431)k
def linearPaddingHastads(cArray,nArray,aArray,bArray,eArray,eps):
if(len(cArray) == len(nArray) == len(aArray) == len(bArray) == len(eArray)):
for i in range(4):
cArray[i] = Integer(cArray[i])
nArray[i] = Integer(nArray[i])
aArray[i] = Integer(aArray[i])
bArray[i] = Integer(bArray[i])
eArray[i] = Integer(eArray[i])
TArray = [-1]*4
for i in range(4):
arrayToCRT = [0]*4
arrayToCRT[i] = 1
TArray[i] = crt(arrayToCRT,nArray)
P.<x> = PolynomialRing(Zmod(prod(nArray)))
gArray = [-1]*4
for i in range(4):
gArray[i] = TArray[i]*(pow(aArray[i]*x**2 + bArray[i],eArray[i]) - cArray[i])
g = sum(gArray)
g = g.monic()
roots = g.small_roots(epsilon=eps)
if(len(roots)== 0):
print("No Solutions found!")
return -1
return roots
else:
print("Input error!")
def nonLinearPadding():
eArr = [3 for i in range(4)]
nArr = [146694460234280339612721415368435987068740712812770728817136582256341063038147863645902264969297892447333024201649306207442798919845916187823646745721109151386096190207317810424580842120750075213595282979568495342617919336417068886973047979116994072272482630372638964064972815256237040541007947708358680368391,65031485534704406281490718325237831433086480239135617407356760819741796565231283220528137697949585150709734732370203390254643835828984376427852793969716489016520923272675090536677771074867975287284694860155903327351119710765174437247599498342292671117884858621418276613385329637307269711179183430246951756029,126172075578367446151297289668746433680600889845504078949758568698284471307000358407453139846282095477016675769468273204536898117467559575203458221600341760844973676129445394999861380625435418853474246813202182316736885441120197888145039130477114127079444939102267586634051045795627433724810346460217871661901,75691424835079457343374072990750986689075078863640186724151061449621926239051140991748483370587430224317778303489124525034113533087612981452189061743589227565099659070008017454957304620495920813121234552401715857719372861565651204968408267740732475458128601061676264465241188491988485848198323410127587280471]
cArr = [129274519334082165644106292383763271862424981496822335330342328217347928093592453953990448827969549377883054831490973006383371688359344675312001881631556371220779971357039899721241880304156884612458373310254854821837978876725801047977081900824202659636258168216028784656056334358157381820784576207338479493823,8140023566779187828652447593867705813386781164538611122714708931585587727699213769519135028841126072130625547328311301696554048174772606261707345115571968105138543476580875347239912760797035694220505996377127309341770427102697008350472060971360460756799310951343070384766137332401117333917901167639276168214,25434511525127530194830986592289179576070740435049947678930286998924519588985583799757299734846614343604661534391991096353170465467791358514448923161460366596251448937540153262731348684727026598527904328268639060306102090278287818149679940661579357649191023269947102746200467430583428889484549034314463114080,9435583236354598287661880148272717764447540972316605192855157484524753847806158586224733743434644389385148450722945845355791145016665856388503878165725148745517696840251674049929524448078129458846254866804153080766917319923905682824180976106679633180818527967145571143203594244851742143986040226240019541346]
aArr = [1 for i in range(4)]
bArr = [i * 3 ** 431 for i in [3,8,10,11]]
msg = linearPaddingHastads(cArr,nArr,aArr,bArr,eArr,eps=1/20)
for i in msg:
print(bytes.fromhex(hex(i)[2:]))
if __name__ == '__main__':
nonLinearPadding()
Least Significant Bit Oracle Attack (LSB Oracle Attack / Parity Oracle)
适用情况:可以选择密文并泄露明文的最低位(奇偶性)。
假设存在一个oracle,能对给定密文进行解密并给出对应明文的奇偶信息,则我们只需要 log N次就能解密任意密文。
在一次RSA加密中,明文为 \(m\),模数为 \(n\),加密指数为 \(e\),密文为 \(c\)。我们可以构造出
\(c’=((2^e)\cdot c)\%n=((2^e)\cdot(m^e))\%n=((2\cdot m)^e)\%n\)
因为 \(m\) 的两倍可能大于 \(n\) ,所以经过解密得到的明文是 \(m’=(2\cdot m)\%n\)
我们还能够知道 \(m’\) 的最低位 lsb 是 1 还是 0 。 因为 \(n\) 是奇数,而 \(2·m\) 是偶数,所以如果 lsb 是 \(0\) ,说明\((2·m)%n\) 是偶数,没有超过 \(n\)
即 \(m\lt \cfrac{n}{2}\) 反之则 \(m\gt \cfrac{n}{2}\)
举个例子就能明白 \(2%3=2\) 是偶数,而 \(4%3=1\) 是奇数。
以此类推,构造密文 \(c’’=((4^e)\cdot c)\%n\) 使其解密后为 \(m’’=(4\cdot m)\%n\)
判断 的奇偶性可以知道 \(m’’\) 和 \(n/4\) 的大小关系。所以我们就有了一个二分算法,可以在对数时间内将 \(m\) 的范围逼近到一个足够狭窄的空间。
更多信息可参考:RSA Least-Significant-Bit Oracle Attack 和 RSA least significant bit oracle attack 。
import decimal
def oracle():
return lsb == 'odd'
def partial(c, e, n):
k = n.bit_length()
decimal.getcontext().prec = k # for 'precise enough' floats
lo = decimal.Decimal(0)
hi = decimal.Decimal(n)
for i in range(k):
if not oracle(c):
hi = (lo + hi) / 2
else:
lo = (lo + hi) / 2
c = (c * pow(2, e, n)) % n
# print i, int(hi - lo)
return int(hi)
Common Private Exponent(共私钥指数攻击,d相同)
加密用同样的私钥并且私钥比较短,从而导致了加密系统被破解。
假定:\(\begin{cases} e_1d=1+k_1\varphi(N_1) \newline e_2d=1+k_2\varphi(N_2) \newline {\vdots} \newline e_rd=1+k_r\varphi(N_r) \end{cases}\)
其中 \(N_1 \lt N_2 \lt \cdots \lt N_r \lt 2N_1\)
构造格:
\(B_r\)=\(\begin{bmatrix}{M}&{e_1}&{e_2}&{\cdots}&{e_{r}}\newline
{0}&{-N_1}&{0}&{\cdots}&{0}\newline{0}&{0}&{-N_2}&{\cdots}&{0}\newline{\vdots}&{\vdots}&{\vdots}&{\ddots}&{\vdots}\newline{0}&{0}&{0}&{\cdots}&{-N_r}\newline\end{bmatrix}\)
其中 \(M=\lfloor N_r^{\frac{1}{2}} \rfloor\)
再利用LLL算法进行规约得到 \(\vert b_1\vert=Md\),则 \(d=\cfrac{\vert b_1 \vert}{M}\) 从而解密密文得到明文。
-
使用条件:
\(d \lt N_r^{\delta_r},\delta_r \lt \cfrac{1}{2}-\cfrac{1}{2(r+1)}-\log_{N_r}{(6)}\) -
参考:
Lattice Based Attack on Common Private Exponent RSASCTF 2020 - RSA
#Sage
from gmpy2 import *
e0=
n0=
c0=
e1=
n1=
c1=
e2=
n2=
c2=
M=iroot(int(n2),int(2))[0]
a=[0]*4
a[0]=[M,e0,e1,e2]
a[1]=[0,-n0,0,0]
a[2]=[0,0,-n1,0]
a[3]=[0,0,0,-n2]
Mat = matrix(ZZ,a)
Mat_LLL=Mat.LLL()
d = abs(Mat_LLL[0][0])/M
print(bytes.fromhex(hex(pow(c1,int(d),int(n1)))[2:]))
多组低解密指数攻击
适用情况:\(2-4\) 组 \(e\) ,且 \(d\) 较小
-
给定2组
\(g=\gcd(p-1,q-1),\lambda(n)=\frac{\varphi(n)}{g},s=1-p-q\)
且有 \(ed-k\lambda(n)=1\) 得到 \(edg-kn=g+ks\quad (1)\)
设 \(e_1\) 对应 \(k_1,e_2\) 对应 \(k_2\)
则有 \(k_{2}d_{1}e{1}-k_{1}d_{2}e_{2}=k_{2}-k_{1}\quad (2)\)
由(1)(2)有:
\(\left\{ \begin{matrix} e_{1}d_{1}g-k_{1}n=g+k_{1}s \newline k_{2}d_{1}e{1}-k_{1}d_{2}e_{2}=k_{2}-k_{1} \newline e_{1}e_{2}d_{1}d_{2}g_{2}-e_{1}d_{1}gk_{2}n-e_{2}d_{2}gk_{1}n+k_{1}k_{2}n^{2}=(g+k_{1}s)(g+k_{2}s) \end{matrix} \right.\)
上述等式组也可表示为
\(bL_2 =[k_{1}k_{2},k_{2}d_{1}g,k_{1}d_{2}g,d_{1}d_{2}g^{2}]\cdot\left[ \begin{matrix} n & -M_{1}n & 0 & n^{2} \newline 0 & M_{1}e_{1} & M_{2}e_{1} & -e_{1}n \newline 0 & 0 & -M_{2}e_{2} & -e_{2}n \newline 0 & 0 & 0 & e_{1}e_{2} \end{matrix} \right] =[k_{1}k_{2}n,M_{1}k_{2}(g+k_{1}s),M_{2}g(k_{2}-k_{1}),(g+k_{1}s)(g+k_{2}s)]\)其中 \(M_{1}=n^{1/2},M_{2}=n^{1+\alpha_{2}},d\approx n^{\alpha_{2}}\)
对部分参数进行上界估计,\(k\) 上界近似于 \(d\approx N^{\alpha_{2}}\)
, \(|s|\)上界 \(\approx N^{1/2}\) g 一般相对极小因此上面的矩阵表示 \(BA=C\) 中,\(C\) 的每个元的 size 都近似 \(n^{1+2\alpha_{2}}\)
所以 \(|C|\approx 2\cdot n^{1+2\alpha_{2}}\)
B 作为格基的格中,最短向量由Minkowski Bounds知 \(\approx \sqrt{4}\det(B)^{1/4}\approx 2\cdot n^{(13/2+\alpha_{2})/4}\)
因此只要满足 \(n^{1+2\alpha_{2}}<n^{(13/2+\alpha_{2})/4}\)
即可将问题转化为SVP \(\alpha_{2}<\frac{5}{14}\)
#Sage n = e1 = e2 = c = from Crypto.Util.number import * for i in range(731, 682, -1): print(i) alpha2 = i / 2048 M1 = round(n ^ 0.5) M2 = round(n ^ (1 + alpha2)) A = Matrix(ZZ, [ [n, -M1*n, 0, n^2], [0, M1*e1, -M2*e1, -e1*n], [0, 0, M2*e2, -e2*n], [0, 0, 0, e1*e2] ]) AL = A.LLL() C = Matrix(ZZ, AL[0]) B = A.solve_left(C)[0] phi1 = floor(e1 * B[1] / B[0]) phi2 = floor(e2 * B[2] / B[0]) d1 = inverse(e1, phi1) d2 = inverse(e2, phi2) m1 = long_to_bytes(pow(c, d1, n)) m2 = long_to_bytes(pow(c, d2, n)) if b"De1" in m1 or b"De1" in m2: print(m1) print(m2) break -
给定3组
类似2组情况,其中
\(b=[k_1k_2k_3,d_1gk_2k_3,k_1d_2gk_3,d_1d_2g^2k_3,k_1k_2d_3g,k_1d_3g,k_2d_3g,d_1d_2d_3g^3]\)\(L_3=\left[\begin{matrix} 1-N & 0 & N^2 & 0 & 0 & 0 & -N^3 \newline e_1 & -e_1 & -e_1N & -e & 0 & e_1N & e_1N^2 \newline 0 & e_2 & -e_2N & 0 & e_2N & 0 & e_2N^2 \newline 0 & 0 & e_1e_2 & 0 & -e_1e_2 & -e_1e_2 & -e_1e_2N \newline 0 & 0 & 0 & e_3 & -e_3N & -e_3N & e_3N^3 \newline 0 & 0 & 0 & 0 & e_1e_3 & 0 & -e_1e_3N \newline 0 & 0 & 0 & 0 & 0 & e_2e_3 & -e_2e_3N \newline 0 & 0 & 0 & 0 & 0 & 0 & e_1e_2e_3 \end{matrix}\right] \times D\)
其中 \(D={\rm diag}(N^{3/2},N,N^{(3/2)+\alpha_3},N^{1/2},N^{(3/2)+\alpha_3},N^{1+\alpha_3},N^{1+\alpha_3},1)\)
-
参考Paper
Common Modulus Attacks on Small Private Exponent RSA and Some Fast Variants (in Practice)
Extending Wiener’s Attack in the Presence of Many Decrypting Exponents
多项式RSA
在整数RSA原理基础上将多项式代入分析:
在有限域上选取两个不可约多项式 \(g(p),g(q)\)
\(g(n)=g(p) \cdot g(q)\)
计算出 \(g(n)\) 的欧拉函数 \(\varphi(g(n))=\varphi\)
选取一个整数 \(e\) 作为公钥,\(e\) 与 \(\varphi\) 是互素的,那么对于明文 \(g(m)\) ,加密过程为 \(g(m)^e \equiv g(c) \pmod {g(n)}\)
计算私钥 d 满足 \(ed \equiv 1 \pmod \varphi\)
则\(g(c)^d \equiv (g(m)^e)^d \equiv g(m)^{ed} \equiv g(m)^{\varphi+1} \pmod {g(n)}\)
同样考虑 \(g(n)\) 与 \(g(m)\) 互素,欧拉定理对于多项式亦成立,
得到 \(g(m)^{\varphi+1} \equiv g(m) \pmod {g(n)}\)
所以 \(g(c)^d \equiv g(m) \pmod {g(n)}\)
显然RSA对于整数的体制可以适用于有限域上的多项式。
★注意:
对于素数 \(x\) , \(\varphi(x)=x-1\)
但是对于不可约多项式 \(g(x)\) , \(\varphi(g(x))=p^n-1\)
(此 \(p\) 为 \(GF(p)\) 的模,此 \(n\) 为多项式最高项次数)
原因:
由欧拉函数定义本身,欧拉函数是小于 \(n\) 的所有与 \(n\) 互质的数的个数。
多项式的欧拉函数则类似,表示不高于 \(g(x)\) 幂级的环内所有多项式中,与 \(g(x)\) 无公因式(非1)的其他多项式的个数,所以每一个不高于 \(g(x)\) 幂级的环内多项式(除了它自己)均满足此条件。
#脚本1
#Sage
#已知p,n,m^e
p=
P = PolynomialRing(Zmod(p), name = 'x')
x = P.gen()
e =
n =
c =
#分解N
q1, q2 = n.factor()
q1, q2 = q1[0], q2[0]
#求φ,注意求法,
phi = (p**q1.degree() - 1) * (p**q2.degree() - 1)
assert gcd(e, phi) == 1
d = inverse_mod(e, phi)
m = pow(c,d,n)
#取多项式系数
flag = bytes(m.coefficients())
print("Flag: ", flag.decode())
#脚本2
#Sage
#已知p=2,n,e,c
p =
P = PolynomialRing(GF(p), name = 'x')
x = P.gen()
e =
n =
R.<a> = GF(2^2049)
c = []
q1, q2 = n.factor()
q1, q2 = q1[0], q2[0]
phi = (p**q1.degree() - 1) * (p**q2.degree() - 1)
assert gcd(e, phi) == 1
d = inverse_mod(e, phi)
ans = ''
for cc in c:
cc = P(R.fetch_int(cc))
m = pow(cc,d,n)
m = R(P(m)).integer_representation()
print(m)
ans += chr(m)
print(ans)
参考:
Weak prime factors (p具线性特征)
适用情况:\(p\) 满足 \(ap=u_0+M_1u_1+\cdots+M_ku_k\)
先根据 \(n\) 确定 \(M\) 的大小,再根据 \(M\) 选取符合要求的 \(k\) 和 \(c\) ,然后构造一个格如下:
\(M(\mathcal{L})=\begin{bmatrix}{1}&{0}&{0}&{\cdots}&{0}&{CM^{2k}} \newline {0}&{1}&{0}&{\cdots}&{0}&{CM^{2k-1}} \newline {\vdots}&{\vdots}&{\vdots}&{\ddots}&{\vdots}&{\vdots} \newline {0}&{0}&{0}&{\cdots}&{1}&{CM} \newline {0}&{0}&{0}&{\cdots}&{0}&{-CN} \newline \end{bmatrix}\)
用LLL算法进行格基规约,将规约后的某个向量作为多项式系数,再对多项式进行分解,即可完成对 \(n\) 的分解。
- 参考
Factoring RSA moduli with weak prime factors
N1CTF2020 - easyRSA
from tqdm import tqdm
import gmpy2
class success(Exception):
pass
def attack_weak_prime(basenum, exp, n):
m = basenum^exp
k = len(n.str(base=basenum))//(2*exp) + 1
c = gmpy2.iroot(2*k^3, int(2))
# assert c[1] == True
tmp = int(c[0])
try:
for c in tqdm(range(1, tmp)):
amount = 2*k+1
M = Matrix(RationalField(), amount, amount)
for i in range(amount):
M[i, i] = 1
M[i, amount-1] = c*m^(2*k-i)
M[amount-1, amount-1] = -c*n
new_basis = M.LLL(delta=0.75)
for j in range(amount):
last_row = list(new_basis[j])
last_row[-1] = last_row[-1]//(-c)
poly = sum(e * x^(k*2-i) for i,e in enumerate(last_row))
fac = poly.factor_list()
if len(fac) == 2:
p_poly, q_poly = fac
p_coefficient = p_poly[0].list()
q_coefficient = q_poly[0].list()
ap = sum(m^i * j for i,j in enumerate(p_coefficient))
bq = sum(m^i * j for i,j in enumerate(q_coefficient))
p = gcd(ap, n)
q = gcd(bq, n)
if (p*q == n) and (p != 1) and (q != 1):
raise success
except:
print ('n =', n)
print ('p =', p)
print ('q =', q)
print ('p*q == n ?', bool(p*q == n))
if __name__ == '__main__':
print ('[+] Weak Prime Factorization Start!')
print ('-------------------------------------------------------------------------------------------------------------------------------')
basenum, exp = (3, 66)
n = 32846178930381020200488205307866106934814063650420574397058108582359767867168248452804404660617617281772163916944703994111784849810233870504925762086155249810089376194662501332106637997915467797720063431587510189901
其他特别情形
-
多素数因子(Multi-prime RSA)
\(n=p_1^{k_1}p_2^{k_2}\cdots p_m^{k_m} \\ \Rightarrow \begin{eqnarray}\varphi(n) &=&\varphi(p_1^{k_1})\varphi(p_2^{k_2}) \cdots \varphi(p_m^{k_m}) \\ &=&(p_1^{k_1-1}\cdot(p_1-1))(p_2^{k_2-1}\cdot(p_2-1)) \cdots (p_m^{k_m-1}\cdot(p_m-1)) \end{eqnarray}\)
-
\(next_prime()\)
根据素数定理,素数的平均间隔为:\(\cfrac{x}{\pi(x)} \approx \ln(x)\),因此常见的下一个素数比当前素数大一点,一般不会超过1500。- 变种1:\(n=p \cdot q \cdot \text{nextprime}(p) \cdot \text{nextprime}(q)\) 费马因式分解。
-
给 \(e,p,c\)
\(c \equiv m^e \pmod n \\\Leftrightarrow c_1 \equiv c \pmod p \equiv m^e \pmod p\)
令 \(ed_1 \equiv 1 \pmod {(p-1)}\),有 \(m \equiv c^d \pmod n \equiv c_1^{d_1} \pmod p\) -
给 \(e,d,modinv(q,p),c\)
已知:\(p,q\) 同比特位数。
令 \(cf=q^{-1} \bmod p\) 有 \(q\cdot cf=1 \pmod p\)
-
\(ed=1+k(p-1)(q-1)\)
比较比特位数,\(k\) 与 \(e\) 同长,可爆破 \(k\),得\(\varphi(n)=(p-1)(q-1)=\cfrac{ed-1}{k}\)
-
上式
\(\varphi(n) =(p-1)(q-1) \pmod p=-(q-1) \pmod p\)结合 \(q\cdot cf=1 \pmod p\),即 \(q\cdot cf-1=0 \pmod p\)
联立:
\(\begin{eqnarray} \varphi(n)&=&(p-1)(q-1)\\&=&pq-p-q+1\\&=&n-p-q+1 \end{eqnarray}\)\(\begin{eqnarray} cf\cdot \varphi(n)&=&cf\cdot(n-p-q+1)\\&=&cf\cdot n-cf\cdot p-cf\cdot q+cf \end{eqnarray}\)
\(\begin{eqnarray} cf\cdot \varphi(n) \bmod p&=&(cf\cdot n-cf\cdot p-cf\cdot q+cf) \bmod p\\&=&0-0-(cf\cdot q)+cf \bmod p\\&=&-1+cf \bmod p \end{eqnarray}\)
有 \(1+cf\cdot \varphi(n)-cf=0\pmod p\)
即 \(x=1+cf\cdot \varphi(n)-cf\)能被 \(p\) 整除;
-
由费马小定理,存在 \(r\) 满足 \(r^{p-1}=1 \pmod p\)
\(\begin{eqnarray}r^{\varphi(n)}&=&(r^{(p-1)})^{(q-1)}\\&=&1^{(q-1)} \pmod p\\&=&1 \pmod p \end{eqnarray}\)
因对于任意 \(r,k_1,k_2\),当 \(k_2\) 为 \(k_1\) 因子时,
\(r \bmod k_2=(r \bmod k_1) \bmod k_2\)
故 \(r^{\varphi(n)} \bmod p=(r^{\varphi(n)} \bmod x) \bmod p=1 \bmod p=kp\)
已知 \(\varphi(n)\) 由 \((r^{\varphi(n)} \bmod x) \bmod p=kp\)
可得到多组 \(p\) 的乘积,计算 gcd 可得到 \(p\);
-
由 \(q\cdot cf=1 \pmod p\) 求模逆可得 \(q\),再用 \(c\) 计算出 \(m\)。
-
-
gcd(e,φ(n)) ≠ 1
\(\gcd(e,\varphi(n))\neq 1\) 时,\(e\) 与 \(\varphi(n)\) 不互素,
\(m^e \equiv (m^{\gcd(e,\varphi(n))})^{\frac{e}{\gcd(e,\varphi(n))}} \equiv c \pmod n\)计算 \(\frac{e}{\gcd(e,\varphi(n))}\) 的模逆 \(d’\)
则 \(c^{d’}\equiv m^{\gcd(e,\varphi(n))}\pmod n\)
当 \(\gcd(e,\varphi(n))\)较小时,可以直接对 \(c\) 开根,有两种情况:
-
\(m ( ( ( ( (e)))))= c<n\),这种情况直接对 \(c\) 开 \(e\) 次方即可;
-
\(m ( ( ( ( (e))))) = c>n\),这种情况需要在有限域下对 \(c\) 开方,一般先计算 \(c_p=c \bmod p\) , \(c_q=c \bmod q\)
分别求出 \(c_p,c_q\) 在 \(c\) 下的 \(e\) 次根(可能有多个),然后使用CRT遍历所有组合,分别check得出明文。
当 \(\gcd(e,\varphi(n))\) 较大时,求 \(p,q\) 的 \(e\) 次根步骤需要替换为一些有限域开根的高效算法(如AMM算法等)进行计算。
参考:
[De1CTF2019 - Baby RSA](https://github.com/De1ta-team/De1CTF2019/blob/master/writeup/crypto/Baby Rsa/README_zh.md "De1CTF2019 - Baby RSA")
0ctf 2016 - RSA? -
-
\(e|(p-1), e|(q-1)\)
上面的 \(\gcd(e,\varphi(n))\neq 1\) 情况不针对 \(\gcd(e,\varphi(n))= e\)这里对 \(e\mid (p-1),e\mid (q-1)\) 的特殊情况进行讨论。
解题思路即求解 m mod p 和 m mod q ,再通过CRT还原 m mod n。主要难点则是在 GF(p) 上求 e 次根。
在有限域上求r-th root有两个常见算法(Adleman-Manders-Miller algorithm和Cipolla-Lehmer algorithm),Namhun Koo提出一种更具一般性的开根算法,且在 s 足够小的时候更高效
r^{s}\mid (p-1),r^{s}\nmid (p-1)★参考:NCTF 2019 - easyRSA (Adleman-Manders-Miller rth Root Extraction Method)
本题则为 \(e\) 和 \(p-1\) (或 \(q-1\) )的最大公约数就是 \(e\) 本身,也就是说 \(e | (p-1)\) ,只有对 \(c\) 开 \(e\) 次方根才行。
可以将同余方程 \(m^e \equiv c \pmod n\) 化成 \(\begin{cases} m^e \equiv c \pmod p \\ m^e \equiv c \pmod q \end{cases}\)
然后分别在 \(GF(p)\) 和 \(GF(q)\) 上对 \(c\) 开 \(e\) 次方根,再用CRT组合一下即可得到在 mod n 下的解。
问题是,如何在有限域内开根?
这里 \(e\) 与 \(p-1\) 和 \(q-1\) 都不互素,不能简单地求个逆元就完事。
这种情况下,开平方根可以用Tonelli–Shanks algorithm,Wiki说这个算法可以扩展到开n次方根。
在这篇paper里给出了具体的算法:Adleman-Manders-Miller rth Root Extraction Method。这个算法只能开出一个根,实际上开 \(e\) 次方,最多会有 \(e\) 个根(这题的情况下有
0x1337个根)。
如何找到其他根?
StackOverflow – Cube root modulo P 给出了方法。
如何找到所有的primitive 0x1337th root of 1?
StackExchange – Finding the n-th root of unity in a finite field 给出了方法。
Exploit(以e=0x1337为例)- 先用
Adleman-Manders-Miller rth Root Extraction Method在 \(GF(p)\) 和 \(GF(q)\) 上对 \(c\) 开 \(e\) 次方根,分别得到一个解。大概不到10秒。 - 然后去找到所有的
0x1336个primitive nth root of 1,乘以上面那个解,得到所有的0x1337个解。大概1分钟。 - 再用CRT对 GF(p)和 GF(q) 上的两组0x1337个解组合成 mod n 下的解,可以得到\(0x1337**2=24196561\)个 mod n 的解。最后能通过check()的即为flag。大概十几分钟。
- 先用
#脚本1
#Sage
import random
import time
# About 3 seconds to run
def AMM(o, r, q):
start = time.time()
print('\n----------------------------------------------------------------------------------')
print('Start to run Adleman-Manders-Miller Root Extraction Method')
print('Try to find one {:#x}th root of {} modulo {}'.format(r, o, q))
g = GF(q)
o = g(o)
p = g(random.randint(1, q))
while p ^ ((q-1) // r) == 1:
p = g(random.randint(1, q))
print('[+] Find p:{}'.format(p))
t = 0
s = q - 1
while s % r == 0:
t += 1
s = s // r
print('[+] Find s:{}, t:{}'.format(s, t))
k = 1
while (k * s + 1) % r != 0:
k += 1
alp = (k * s + 1) // r
print('[+] Find alp:{}'.format(alp))
a = p ^ (r**(t-1) * s)
b = o ^ (r*alp - 1)
c = p ^ s
h = 1
for i in range(1, t):
d = b ^ (r^(t-1-i))
if d == 1:
j = 0
else:
print('[+] Calculating DLP...')
j = - discrete_log(a, d)
print('[+] Finish DLP...')
b = b * (c^r)^j
h = h * c^j
c = c ^ r
result = o^alp * h
end = time.time()
print("Finished in {} seconds.".format(end - start))
print('Find one solution: {}'.format(result))
return result
def findAllPRoot(p, e):
print("Start to find all the Primitive {:#x}th root of 1 modulo {}.".format(e, p))
start = time.time()
proot = set()
while len(proot) < e:
proot.add(pow(random.randint(2, p-1), (p-1)//e, p))
end = time.time()
print("Finished in {} seconds.".format(end - start))
return proot
def findAllSolutions(mp, proot, cp, p):
print("Start to find all the {:#x}th root of {} modulo {}.".format(e, cp, p))
start = time.time()
all_mp = set()
for root in proot:
mp2 = mp * root % p
assert(pow(mp2, e, p) == cp)
all_mp.add(mp2)
end = time.time()
print("Finished in {} seconds.".format(end - start))
return all_mp
c = 10562302690541901187975815594605242014385201583329309191736952454310803387032252007244962585846519762051885640856082157060593829013572592812958261432327975138581784360302599265408134332094134880789013207382277849503344042487389850373487656200657856862096900860792273206447552132458430989534820256156021128891296387414689693952047302604774923411425863612316726417214819110981605912408620996068520823370069362751149060142640529571400977787330956486849449005402750224992048562898004309319577192693315658275912449198365737965570035264841782399978307388920681068646219895287752359564029778568376881425070363592696751183359
p = 199138677823743837339927520157607820029746574557746549094921488292877226509198315016018919385259781238148402833316033634968163276198999279327827901879426429664674358844084491830543271625147280950273934405879341438429171453002453838897458102128836690385604150324972907981960626767679153125735677417397078196059
q = 112213695905472142415221444515326532320352429478341683352811183503269676555434601229013679319423878238944956830244386653674413411658696751173844443394608246716053086226910581400528167848306119179879115809778793093611381764939789057524575349501163689452810148280625226541609383166347879832134495444706697124741
e = 0x1337
cp = c % p
cq = c % q
mp = AMM(cp, e, p)
mq = AMM(cq, e, q)
p_proot = findAllPRoot(p, e)
q_proot = findAllPRoot(q, e)
mps = findAllSolutions(mp, p_proot, cp, p)
mqs = findAllSolutions(mq, q_proot, cq, q)
print(mps, mqs)
def check(m):
h = m.hex()
if len(h) & 1:
return False
if bytes.fromhex(h).startswith(b'NCTF'):
print(bytes.fromhex(h))
return True
else:
return False
# About 16 mins to run 0x1337^2 == 24196561 times CRT
start = time.time()
print('Start CRT...')
for mpp in mps:
for mqq in mqs:
solution = CRT_list([int(mpp), int(mqq)], [p, q])
if check(solution):
print(solution)
print(time.time() - start)
end = time.time()
print("Finished in {} seconds.".format(end - start))
#脚本2
#Sage
c = 346925245648012783854132941104554194717281878370806475831055718275298366664505658836564073456294047402009856656647760
p = 21122913513992623721920275602985463699928507831138027
q = 16471885912035642894544190467774867069446937372970845578732298073
e = 239
P.<a>=PolynomialRing(Zmod(p),implementation='NTL')
f=a^e-c
mps=f.monic().roots()
P.<a>=PolynomialRing(Zmod(q),implementation='NTL')
g=a^e-c
mqs=g.monic().roots()
for mpp in mps:
x=mpp[0]
for mqq in mqs:
y=mqq[0]
solution = hex(CRT_list([int(x), int(y)], [p, q]))[2:]
if solution.startswith('666c'):
print(solution)
-
SMUPE 问题(不同\(N,e\)加密线性关系明文)
a system of univariate polynomial equations problem = 一元多项式方程组求解问题-
定义
k 是一个整数,N 为满足RSA算法的模数,\(\delta\) 是多项式的阶。有 \(N_i<N_{i+1},\delta_i \in N\quad(i=1,2,\cdots,k)\)多项式方程组表示如下, 目的是求解 \(x\):\(\begin{cases} f_1(x)\equiv 0 \pmod {N_1}\newline f_2(x)\equiv 0 \pmod {N_2} \newline {\vdots} \newline f_k(x)\equiv 0 \pmod {N_k} \end{cases}\)
-
求解条件
Alexander May, Maike Ritzenhofent提出一种求解方法,简单地说当多项式的阶 \(\delta\)
满足以下情况时可解(\(\delta\)是多项式的阶):\(\sum\limits_{i=1}^k \cfrac{1}{\delta_i} \geq 1\)
具体描述:
令 \((f_i,\delta_i,N_i) \quad(i=1,2,\cdots,k)\) 作为SMUPE问题的首一多项式组,定义 \(M=\prod\limits_{i=1}^k N_i^{\frac{\delta}{\delta_i}},\delta=\text{lcm}(\delta_i) \quad (i=1,2,\cdots,k)\)
则SMUPE问题可以在 \(O(\delta^6\cdot \log_2M)\) 复杂度解决。
参考:2019红帽杯 - 精明的Alice
-
-
反素数(emirp数)
已知:\(q=\text{reverse_x}(p)\) , \(x\) 为进制数。
爆破思路类似RSA parity oracle。
\(p,q\) 是bit翻转关系,已知 \(p\) 最低的 \(k\) 位,则已知 \(q\) 最高的 \(k\) 位。 假设已知 \(k\) 位的 \(p,q\),记为 \(ph,qh\),利用不等式
\(ph\cdot qh\cdot 2^{1024-2k}<=n<(ph+1)\cdot(qh+1)\cdot 2^{1024-2k}\)逐位向低地址爆破,不断收缩不等式的范围,最终可求得 \(n\) 值。
参考:
ASIS 2015 Finals: RSASR
Midnight Sun CTF 2020 Quals
RoarCTF 2020 - Reverse
#python2
#x=10
n = 6528060431134312098979986223024580864611046696815854430382374273411300418237131352745191078493977589108885811759425485490763751348287769344905469074809576433677010568815441304709680418296164156409562517530459274464091661561004894449297362571476259873657346997681362092440259333170797190642839587892066761627543
def t(a, b, k):
# sqrt(n) has 155 digits, so we need to figure out 77 digits on each side
if k == 77:
if a*b == n:
print a, b
return
for i in xrange(10):
for j in xrange(10):
# we try to guess the last not-already-guessed digits of both primes
a1 = a + i*(10**k) + j*(10**(154-k))
b1 = b + j*(10**k) + i*(10**(154-k))
if a1*b1 > n:
# a1 and b1 are too large
continue
if (a1+(10**(154-k)))*(b1+(10**(154-k))) < n:
# a1 and b1 are too small
continue
if ((a1*b1)%(10**(k+1))) != (n%(10**(k+1))):
# The last digits of a1*b1 (which won't change later) doesn't match n
continue
# this a1 and b1 seem to be a possible match, try to guess remaining digits
t(a1, b1, k+1)
# the primes have odd number of digits (155), so we try all possible middle digits (it simplifies the code)
for i in xrange(10):
t(i*(10**77), i*(10**77), 0)
-
4p-1 method
对使用一类特定素数乘积的模数的分解。
当一类特殊的素数用在 RSA 模数中时,可以轻易的将该素数从 n 中分解出来。由于这一类素数都形如 4p−1=Ds ( ( (2))),因此又被称为4p-1 method。此外,有些人也会将其视为 RSA 的后门之一,称之为RSA backdoor。- QiCheng Prime
Ds=
- QiCheng Prime
import sys
sys.setrecursionlimit(10^6)
def QiCheng(n):
R = Integers(n)
attempts = 20
js = [0, (-2^5)^3, (-2^5*3)^3, (-2^5*3*5)^3, (-2^5*3*5*11)^3, (-2^6*3*5*23*29)^3]
for _ in range(attempts):
for j in js:
if j == 0:
a = R.random_element()
E = EllipticCurve([0, a])
else:
a = R(j)/(R(1728)-R(j))
c = R.random_element()
E = EllipticCurve([3*a*c^2, 2*a*c^3])
x = R.random_element()
z = E.division_polynomial(n, x)
g = gcd(z, n)
if g > 1:
return g
n =
p = int(QiCheng(Integer(n)))
-
Masaaki Shirase & Vladimir Sedlacek Improvement
更多 Ds 值。
CM-based factorization
参考:
浅谈 QiCheng Prime
NCTF 2020 - RSA_revenge
CryptoHack Challenge - RSA Backdoor Viability -
Common Prime RSA
情形:gcd(p-1,q-1)=g
分解的n方法有四种:
(1)修改Pollard’s rho方法分解n;
(2)知道a、b的值分解n;
(3)知道g的值分解n;
(4)分解N-1。
# Pollard’s rho
def f(x, n):
return (pow(x, n - 1, n) + 3) % n
def rho(n):
i = 1
print 'Factorizing'
while True:
x1 = getRandomRange(2, n)
x2 = f(x1, n)
j = 1
while True:
p = gmpy2.gcd(abs(x1 - x2), n)
if p == n:
break
elif p > 1 and isPrime(p):
print 'Found!'
return (p, n // p)
else:
x1 = f(x1, n)
x2 = f(f(x2, n), n)
j += 1
i += 1
详细原理
[Cryptanalysis of RSA and It’s Variants](http://index-of.es/Varios-2/Cryptanalysis of RSA and It's Variants.pdf)


 浙公网安备 33010602011771号
浙公网安备 33010602011771号