概要
上一章通过C语言实现了AVL树,本章将介绍AVL树的C++版本,算法与C语言版本的一样。
目录
1. AVL树的介绍
2. AVL树的C++实现
3. AVL树的C++测试程序
转载请注明出处:http://www.cnblogs.com/skywang12345/p/3577360.html
更多内容: 数据结构与算法系列 目录
(01) AVL树(一)之 图文解析 和 C语言的实现
(02) AVL树(二)之 C++的实现
(03) AVL树(三)之 Java的实现
AVL树的介绍
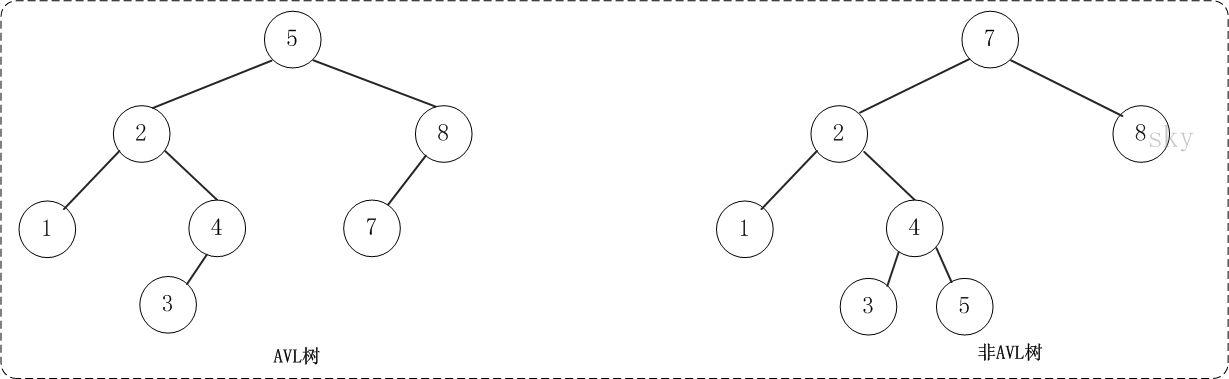
AVL树是高度平衡的而二叉树。它的特点是:AVL树中任何节点的两个子树的高度最大差别为1。
上面的两张图片,左边的是AVL树,它的任何节点的两个子树的高度差别都<=1;而右边的不是AVL树,因为7的两颗子树的高度相差为2(以2为根节点的树的高度是3,而以8为根节点的树的高度是1)。
AVL树的C++实现
1. 节点
1.1 AVL树节点
template <class T> class AVLTreeNode{ public: T key; // 关键字(键值) int height; // 高度 AVLTreeNode *left; // 左孩子 AVLTreeNode *right; // 右孩子 AVLTreeNode(T value, AVLTreeNode *l, AVLTreeNode *r): key(value), height(0),left(l),right(r) {} };
AVLTreeNode是AVL树的节点类,它包括的几个组成对象:
(01) key -- 是关键字,是用来对AVL树的节点进行排序的。
(02) left -- 是左孩子。
(03) right -- 是右孩子。
(04) height -- 是高度。
1.2 AVL树
template <class T> class AVLTree { private: AVLTreeNode<T> *mRoot; // 根结点 public: AVLTree(); ~AVLTree(); // 获取树的高度 int height(); // 获取树的高度 int max(int a, int b); // 前序遍历"AVL树" void preOrder(); // 中序遍历"AVL树" void inOrder(); // 后序遍历"AVL树" void postOrder(); // (递归实现)查找"AVL树"中键值为key的节点 AVLTreeNode<T>* search(T key); // (非递归实现)查找"AVL树"中键值为key的节点 AVLTreeNode<T>* iterativeSearch(T key); // 查找最小结点:返回最小结点的键值。 T minimum(); // 查找最大结点:返回最大结点的键值。 T maximum(); // 将结点(key为节点键值)插入到AVL树中 void insert(T key); // 删除结点(key为节点键值) void remove(T key); // 销毁AVL树 void destroy(); // 打印AVL树 void print(); private: // 获取树的高度 int height(AVLTreeNode<T>* tree) ; // 前序遍历"AVL树" void preOrder(AVLTreeNode<T>* tree) const; // 中序遍历"AVL树" void inOrder(AVLTreeNode<T>* tree) const; // 后序遍历"AVL树" void postOrder(AVLTreeNode<T>* tree) const; // (递归实现)查找"AVL树x"中键值为key的节点 AVLTreeNode<T>* search(AVLTreeNode<T>* x, T key) const; // (非递归实现)查找"AVL树x"中键值为key的节点 AVLTreeNode<T>* iterativeSearch(AVLTreeNode<T>* x, T key) const; // 查找最小结点:返回tree为根结点的AVL树的最小结点。 AVLTreeNode<T>* minimum(AVLTreeNode<T>* tree); // 查找最大结点:返回tree为根结点的AVL树的最大结点。 AVLTreeNode<T>* maximum(AVLTreeNode<T>* tree); // LL:左左对应的情况(左单旋转)。 AVLTreeNode<T>* leftLeftRotation(AVLTreeNode<T>* k2); // RR:右右对应的情况(右单旋转)。 AVLTreeNode<T>* rightRightRotation(AVLTreeNode<T>* k1); // LR:左右对应的情况(左双旋转)。 AVLTreeNode<T>* leftRightRotation(AVLTreeNode<T>* k3); // RL:右左对应的情况(右双旋转)。 AVLTreeNode<T>* rightLeftRotation(AVLTreeNode<T>* k1); // 将结点(z)插入到AVL树(tree)中 AVLTreeNode<T>* insert(AVLTreeNode<T>* &tree, T key); // 删除AVL树(tree)中的结点(z),并返回被删除的结点 AVLTreeNode<T>* remove(AVLTreeNode<T>* &tree, AVLTreeNode<T>* z); // 销毁AVL树 void destroy(AVLTreeNode<T>* &tree); // 打印AVL树 void print(AVLTreeNode<T>* tree, T key, int direction); };
AVLTree是AVL树对应的类。它包含AVL树的根节点mRoot和AVL树的基本操作接口。需要说明的是:AVLTree中重载了许多函数。重载的目的是区分内部接口和外部接口,例如insert()函数而言,insert(tree, key)是内部接口,而insert(key)是外部接口。
1.2 树的高度
/* * 获取树的高度 */ template <class T> int AVLTree<T>::height(AVLTreeNode<T>* tree) { if (tree != NULL) return tree->height; return 0; } template <class T> int AVLTree<T>::height() { return height(mRoot); }
关于高度,有的地方将"空二叉树的高度是-1",而本文采用维基百科上的定义:树的高度为最大层次。即空的二叉树的高度是0,非空树的高度等于它的最大层次(根的层次为1,根的子节点为第2层,依次类推)。
1.3 比较大小
/* * 比较两个值的大小 */ template <class T> int AVLTree<T>::max(int a, int b) { return a>b ? a : b; }
2. 旋转
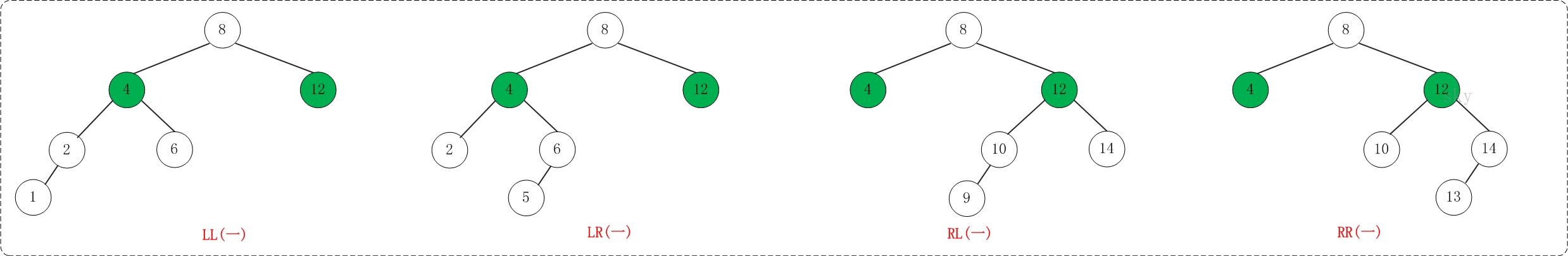
如果在AVL树中进行插入或删除节点后,可能导致AVL树失去平衡。这种失去平衡的可以概括为4种姿态:LL(左左),LR(左右),RR(右右)和RL(右左)。下面给出它们的示意图:
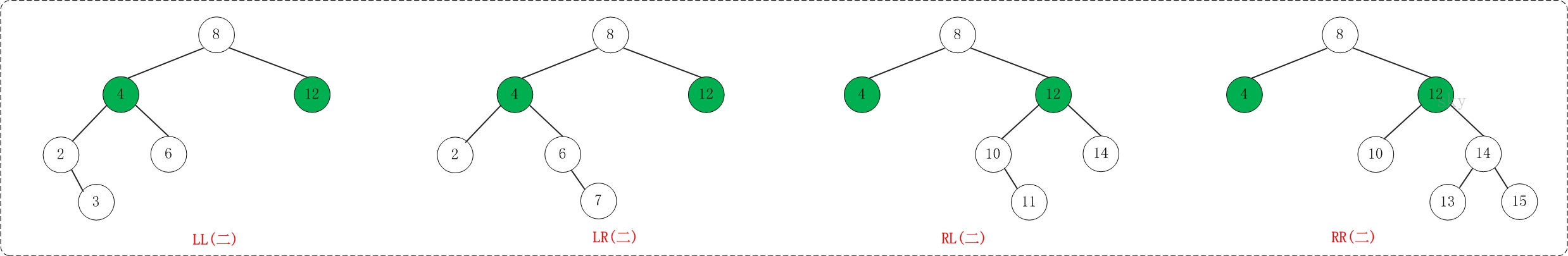
上图中的4棵树都是"失去平衡的AVL树",从左往右的情况依次是:LL、LR、RL、RR。除了上面的情况之外,还有其它的失去平衡的AVL树,如下图:
上面的两张图都是为了便于理解,而列举的关于"失去平衡的AVL树"的例子。总的来说,AVL树失去平衡时的情况一定是LL、LR、RL、RR这4种之一,它们都由各自的定义:
(1) LL:LeftLeft,也称为"左左"。插入或删除一个节点后,根节点的左子树的左子树还有非空子节点,导致"根的左子树的高度"比"根的右子树的高度"大2,导致AVL树失去了平衡。
例如,在上面LL情况中,由于"根节点(8)的左子树(4)的左子树(2)还有非空子节点",而"根节点(8)的右子树(12)没有子节点";导致"根节点(8)的左子树(4)高度"比"根节点(8)的右子树(12)"高2。
(2) LR:LeftRight,也称为"左右"。插入或删除一个节点后,根节点的左子树的右子树还有非空子节点,导致"根的左子树的高度"比"根的右子树的高度"大2,导致AVL树失去了平衡。
例如,在上面LR情况中,由于"根节点(8)的左子树(4)的左子树(6)还有非空子节点",而"根节点(8)的右子树(12)没有子节点";导致"根节点(8)的左子树(4)高度"比"根节点(8)的右子树(12)"高2。
(3) RL:RightLeft,称为"右左"。插入或删除一个节点后,根节点的右子树的左子树还有非空子节点,导致"根的右子树的高度"比"根的左子树的高度"大2,导致AVL树失去了平衡。
例如,在上面RL情况中,由于"根节点(8)的右子树(12)的左子树(10)还有非空子节点",而"根节点(8)的左子树(4)没有子节点";导致"根节点(8)的右子树(12)高度"比"根节点(8)的左子树(4)"高2。
(4) RR:RightRight,称为"右右"。插入或删除一个节点后,根节点的右子树的右子树还有非空子节点,导致"根的右子树的高度"比"根的左子树的高度"大2,导致AVL树失去了平衡。
例如,在上面RR情况中,由于"根节点(8)的右子树(12)的右子树(14)还有非空子节点",而"根节点(8)的左子树(4)没有子节点";导致"根节点(8)的右子树(12)高度"比"根节点(8)的左子树(4)"高2。
前面说过,如果在AVL树中进行插入或删除节点后,可能导致AVL树失去平衡。AVL失去平衡之后,可以通过旋转使其恢复平衡,下面分别介绍"LL(左左),LR(左右),RR(右右)和RL(右左)"这4种情况对应的旋转方法。
2.1 LL的旋转
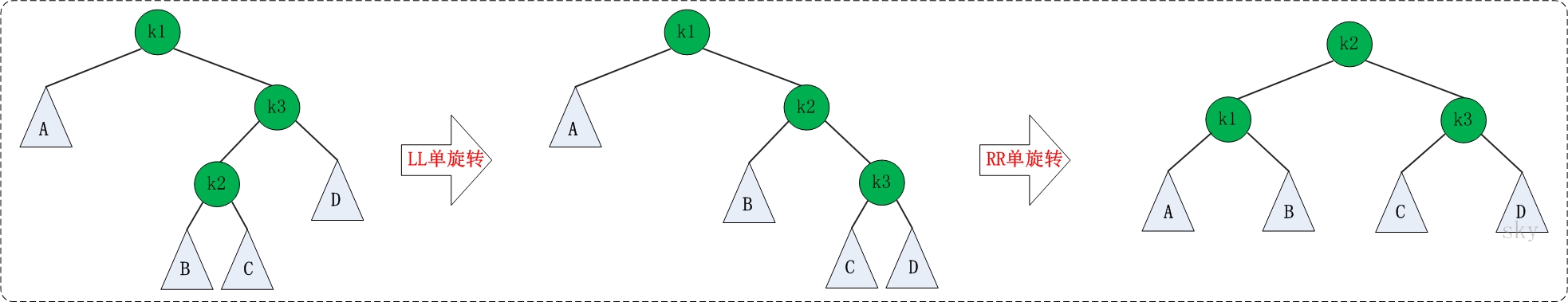
LL失去平衡的情况,可以通过一次旋转让AVL树恢复平衡。如下图:
图中左边是旋转之前的树,右边是旋转之后的树。从中可以发现,旋转之后的树又变成了AVL树,而且该旋转只需要一次即可完成。
对于LL旋转,你可以这样理解为:LL旋转是围绕"失去平衡的AVL根节点"进行的,也就是节点k2;而且由于是LL情况,即左左情况,就用手抓着"左孩子,即k1"使劲摇。将k1变成根节点,k2变成k1的右子树,"k1的右子树"变成"k2的左子树"。
LL的旋转代码
/* * LL:左左对应的情况(左单旋转)。 * * 返回值:旋转后的根节点 */ template <class T> AVLTreeNode<T>* AVLTree<T>::leftLeftRotation(AVLTreeNode<T>* k2) { AVLTreeNode<T>* k1; k1 = k2->left; k2->left = k1->right; k1->right = k2; k2->height = max( height(k2->left), height(k2->right)) + 1; k1->height = max( height(k1->left), k2->height) + 1; return k1; }
2.2 RR的旋转
理解了LL之后,RR就相当容易理解了。RR是与LL对称的情况!RR恢复平衡的旋转方法如下:
图中左边是旋转之前的树,右边是旋转之后的树。RR旋转也只需要一次即可完成。
RR的旋转代码
/* * RR:右右对应的情况(右单旋转)。 * * 返回值:旋转后的根节点 */ template <class T> AVLTreeNode<T>* AVLTree<T>::rightRightRotation(AVLTreeNode<T>* k1) { AVLTreeNode<T>* k2; k2 = k1->right; k1->right = k2->left; k2->left = k1; k1->height = max( height(k1->left), height(k1->right)) + 1; k2->height = max( height(k2->right), k1->height) + 1; return k2; }
2.3 LR的旋转
LR失去平衡的情况,需要经过两次旋转才能让AVL树恢复平衡。如下图:
第一次旋转是围绕"k1"进行的"RR旋转",第二次是围绕"k3"进行的"LL旋转"。
LR的旋转代码
/* * LR:左右对应的情况(左双旋转)。 * * 返回值:旋转后的根节点 */ template <class T> AVLTreeNode<T>* AVLTree<T>::leftRightRotation(AVLTreeNode<T>* k3) { k3->left = rightRightRotation(k3->left); return leftLeftRotation(k3); }
2.4 RL的旋转
RL是与LR的对称情况!RL恢复平衡的旋转方法如下:
第一次旋转是围绕"k3"进行的"LL旋转",第二次是围绕"k1"进行的"RR旋转"。
RL的旋转代码
/* * RL:右左对应的情况(右双旋转)。 * * 返回值:旋转后的根节点 */ template <class T> AVLTreeNode<T>* AVLTree<T>::rightLeftRotation(AVLTreeNode<T>* k1) { k1->right = leftLeftRotation(k1->right); return rightRightRotation(k1); }
3. 插入
插入节点的代码
/* * 将结点插入到AVL树中,并返回根节点 * * 参数说明: * tree AVL树的根结点 * key 插入的结点的键值 * 返回值: * 根节点 */ template <class T> AVLTreeNode<T>* AVLTree<T>::insert(AVLTreeNode<T>* &tree, T key) { if (tree == NULL) { // 新建节点 tree = new AVLTreeNode<T>(key, NULL, NULL); if (tree==NULL) { cout << "ERROR: create avltree node failed!" << endl; return NULL; } } else if (key < tree->key) // 应该将key插入到"tree的左子树"的情况 { tree->left = insert(tree->left, key); // 插入节点后,若AVL树失去平衡,则进行相应的调节。 if (height(tree->left) - height(tree->right) == 2) { if (key < tree->left->key) tree = leftLeftRotation(tree); else tree = leftRightRotation(tree); } } else if (key > tree->key) // 应该将key插入到"tree的右子树"的情况 { tree->right = insert(tree->right, key); // 插入节点后,若AVL树失去平衡,则进行相应的调节。 if (height(tree->right) - height(tree->left) == 2) { if (key > tree->right->key) tree = rightRightRotation(tree); else tree = rightLeftRotation(tree); } } else //key == tree->key) { cout << "添加失败:不允许添加相同的节点!" << endl; } tree->height = max( height(tree->left), height(tree->right)) + 1; return tree; } template <class T> void AVLTree<T>::insert(T key) { insert(mRoot, key); }
4. 删除
删除节点的代码
/* * 删除结点(z),返回根节点 * * 参数说明: * tree AVL树的根结点 * z 待删除的结点 * 返回值: * 根节点 */ template <class T> AVLTreeNode<T>* AVLTree<T>::remove(AVLTreeNode<T>* &tree, AVLTreeNode<T>* z) { // 根为空 或者 没有要删除的节点,直接返回NULL。 if (tree==NULL || z==NULL) return NULL; if (z->key < tree->key) // 待删除的节点在"tree的左子树"中 { tree->left = remove(tree->left, z); // 删除节点后,若AVL树失去平衡,则进行相应的调节。 if (height(tree->right) - height(tree->left) == 2) { AVLTreeNode<T> *r = tree->right; if (height(r->left) > height(r->right)) tree = rightLeftRotation(tree); else tree = rightRightRotation(tree); } } else if (z->key > tree->key)// 待删除的节点在"tree的右子树"中 { tree->right = remove(tree->right, z); // 删除节点后,若AVL树失去平衡,则进行相应的调节。 if (height(tree->left) - height(tree->right) == 2) { AVLTreeNode<T> *l = tree->left; if (height(l->right) > height(l->left)) tree = leftRightRotation(tree); else tree = leftLeftRotation(tree); } } else // tree是对应要删除的节点。 { // tree的左右孩子都非空 if ((tree->left!=NULL) && (tree->right!=NULL)) { if (height(tree->left) > height(tree->right)) { // 如果tree的左子树比右子树高; // 则(01)找出tree的左子树中的最大节点 // (02)将该最大节点的值赋值给tree。 // (03)删除该最大节点。 // 这类似于用"tree的左子树中最大节点"做"tree"的替身; // 采用这种方式的好处是:删除"tree的左子树中最大节点"之后,AVL树仍然是平衡的。 AVLTreeNode<T>* max = maximum(tree->left); tree->key = max->key; tree->left = remove(tree->left, max); } else { // 如果tree的左子树不比右子树高(即它们相等,或右子树比左子树高1) // 则(01)找出tree的右子树中的最小节点 // (02)将该最小节点的值赋值给tree。 // (03)删除该最小节点。 // 这类似于用"tree的右子树中最小节点"做"tree"的替身; // 采用这种方式的好处是:删除"tree的右子树中最小节点"之后,AVL树仍然是平衡的。 AVLTreeNode<T>* min = maximum(tree->right); tree->key = min->key; tree->right = remove(tree->right, min); } } else { AVLTreeNode<T>* tmp = tree; tree = (tree->left!=NULL) ? tree->left : tree->right; delete tmp; } } return tree; } template <class T> void AVLTree<T>::remove(T key) { AVLTreeNode<T>* z; if ((z = search(mRoot, key)) != NULL) mRoot = remove(mRoot, z); }
注意:关于AVL树的"前序遍历"、"中序遍历"、"后序遍历"、"最大值"、"最小值"、"查找"、"打印"、"销毁"等接口与"二叉查找树"基本一样,这些操作在"二叉查找树"中已经介绍过了,这里就不再单独介绍了。当然,后文给出的AVL树的完整源码中,有给出这些API的实现代码。这些接口很简单,Please RTFSC(Read The Fucking Source Code)!
完整的实现代码
AVL树的实现文件(AVRTree.h)

1 #ifndef _AVL_TREE_HPP_ 2 #define _AVL_TREE_HPP_ 3 4 #include <iomanip> 5 #include <iostream> 6 using namespace std; 7 8 template <class T> 9 class AVLTreeNode{ 10 public: 11 T key; // 关键字(键值) 12 int height; // 高度 13 AVLTreeNode *left; // 左孩子 14 AVLTreeNode *right; // 右孩子 15 16 AVLTreeNode(T value, AVLTreeNode *l, AVLTreeNode *r): 17 key(value), height(0),left(l),right(r) {} 18 }; 19 20 template <class T> 21 class AVLTree { 22 private: 23 AVLTreeNode<T> *mRoot; // 根结点 24 25 public: 26 AVLTree(); 27 ~AVLTree(); 28 29 // 获取树的高度 30 int height(); 31 // 获取树的高度 32 int max(int a, int b); 33 34 // 前序遍历"AVL树" 35 void preOrder(); 36 // 中序遍历"AVL树" 37 void inOrder(); 38 // 后序遍历"AVL树" 39 void postOrder(); 40 41 // (递归实现)查找"AVL树"中键值为key的节点 42 AVLTreeNode<T>* search(T key); 43 // (非递归实现)查找"AVL树"中键值为key的节点 44 AVLTreeNode<T>* iterativeSearch(T key); 45 46 // 查找最小结点:返回最小结点的键值。 47 T minimum(); 48 // 查找最大结点:返回最大结点的键值。 49 T maximum(); 50 51 // 将结点(key为节点键值)插入到AVL树中 52 void insert(T key); 53 54 // 删除结点(key为节点键值) 55 void remove(T key); 56 57 // 销毁AVL树 58 void destroy(); 59 60 // 打印AVL树 61 void print(); 62 private: 63 // 获取树的高度 64 int height(AVLTreeNode<T>* tree) ; 65 66 // 前序遍历"AVL树" 67 void preOrder(AVLTreeNode<T>* tree) const; 68 // 中序遍历"AVL树" 69 void inOrder(AVLTreeNode<T>* tree) const; 70 // 后序遍历"AVL树" 71 void postOrder(AVLTreeNode<T>* tree) const; 72 73 // (递归实现)查找"AVL树x"中键值为key的节点 74 AVLTreeNode<T>* search(AVLTreeNode<T>* x, T key) const; 75 // (非递归实现)查找"AVL树x"中键值为key的节点 76 AVLTreeNode<T>* iterativeSearch(AVLTreeNode<T>* x, T key) const; 77 78 // 查找最小结点:返回tree为根结点的AVL树的最小结点。 79 AVLTreeNode<T>* minimum(AVLTreeNode<T>* tree); 80 // 查找最大结点:返回tree为根结点的AVL树的最大结点。 81 AVLTreeNode<T>* maximum(AVLTreeNode<T>* tree); 82 83 // LL:左左对应的情况(左单旋转)。 84 AVLTreeNode<T>* leftLeftRotation(AVLTreeNode<T>* k2); 85 86 // RR:右右对应的情况(右单旋转)。 87 AVLTreeNode<T>* rightRightRotation(AVLTreeNode<T>* k1); 88 89 // LR:左右对应的情况(左双旋转)。 90 AVLTreeNode<T>* leftRightRotation(AVLTreeNode<T>* k3); 91 92 // RL:右左对应的情况(右双旋转)。 93 AVLTreeNode<T>* rightLeftRotation(AVLTreeNode<T>* k1); 94 95 // 将结点(z)插入到AVL树(tree)中 96 AVLTreeNode<T>* insert(AVLTreeNode<T>* &tree, T key); 97 98 // 删除AVL树(tree)中的结点(z),并返回被删除的结点 99 AVLTreeNode<T>* remove(AVLTreeNode<T>* &tree, AVLTreeNode<T>* z); 100 101 // 销毁AVL树 102 void destroy(AVLTreeNode<T>* &tree); 103 104 // 打印AVL树 105 void print(AVLTreeNode<T>* tree, T key, int direction); 106 }; 107 108 /* 109 * 构造函数 110 */ 111 template <class T> 112 AVLTree<T>::AVLTree():mRoot(NULL) 113 { 114 } 115 116 /* 117 * 析构函数 118 */ 119 template <class T> 120 AVLTree<T>::~AVLTree() 121 { 122 destroy(mRoot); 123 } 124 125 /* 126 * 获取树的高度 127 */ 128 template <class T> 129 int AVLTree<T>::height(AVLTreeNode<T>* tree) 130 { 131 if (tree != NULL) 132 return tree->height; 133 134 return 0; 135 } 136 137 template <class T> 138 int AVLTree<T>::height() 139 { 140 return height(mRoot); 141 } 142 /* 143 * 比较两个值的大小 144 */ 145 template <class T> 146 int AVLTree<T>::max(int a, int b) 147 { 148 return a>b ? a : b; 149 } 150 151 /* 152 * 前序遍历"AVL树" 153 */ 154 template <class T> 155 void AVLTree<T>::preOrder(AVLTreeNode<T>* tree) const 156 { 157 if(tree != NULL) 158 { 159 cout<< tree->key << " " ; 160 preOrder(tree->left); 161 preOrder(tree->right); 162 } 163 } 164 165 template <class T> 166 void AVLTree<T>::preOrder() 167 { 168 preOrder(mRoot); 169 } 170 171 /* 172 * 中序遍历"AVL树" 173 */ 174 template <class T> 175 void AVLTree<T>::inOrder(AVLTreeNode<T>* tree) const 176 { 177 if(tree != NULL) 178 { 179 inOrder(tree->left); 180 cout<< tree->key << " " ; 181 inOrder(tree->right); 182 } 183 } 184 185 template <class T> 186 void AVLTree<T>::inOrder() 187 { 188 inOrder(mRoot); 189 } 190 191 /* 192 * 后序遍历"AVL树" 193 */ 194 template <class T> 195 void AVLTree<T>::postOrder(AVLTreeNode<T>* tree) const 196 { 197 if(tree != NULL) 198 { 199 postOrder(tree->left); 200 postOrder(tree->right); 201 cout<< tree->key << " " ; 202 } 203 } 204 205 template <class T> 206 void AVLTree<T>::postOrder() 207 { 208 postOrder(mRoot); 209 } 210 211 /* 212 * (递归实现)查找"AVL树x"中键值为key的节点 213 */ 214 template <class T> 215 AVLTreeNode<T>* AVLTree<T>::search(AVLTreeNode<T>* x, T key) const 216 { 217 if (x==NULL || x->key==key) 218 return x; 219 220 if (key < x->key) 221 return search(x->left, key); 222 else 223 return search(x->right, key); 224 } 225 226 template <class T> 227 AVLTreeNode<T>* AVLTree<T>::search(T key) 228 { 229 return search(mRoot, key); 230 } 231 232 /* 233 * (非递归实现)查找"AVL树x"中键值为key的节点 234 */ 235 template <class T> 236 AVLTreeNode<T>* AVLTree<T>::iterativeSearch(AVLTreeNode<T>* x, T key) const 237 { 238 while ((x!=NULL) && (x->key!=key)) 239 { 240 if (key < x->key) 241 x = x->left; 242 else 243 x = x->right; 244 } 245 246 return x; 247 } 248 249 template <class T> 250 AVLTreeNode<T>* AVLTree<T>::iterativeSearch(T key) 251 { 252 return iterativeSearch(mRoot, key); 253 } 254 255 /* 256 * 查找最小结点:返回tree为根结点的AVL树的最小结点。 257 */ 258 template <class T> 259 AVLTreeNode<T>* AVLTree<T>::minimum(AVLTreeNode<T>* tree) 260 { 261 if (tree == NULL) 262 return NULL; 263 264 while(tree->left != NULL) 265 tree = tree->left; 266 return tree; 267 } 268 269 template <class T> 270 T AVLTree<T>::minimum() 271 { 272 AVLTreeNode<T> *p = minimum(mRoot); 273 if (p != NULL) 274 return p->key; 275 276 return (T)NULL; 277 } 278 279 /* 280 * 查找最大结点:返回tree为根结点的AVL树的最大结点。 281 */ 282 template <class T> 283 AVLTreeNode<T>* AVLTree<T>::maximum(AVLTreeNode<T>* tree) 284 { 285 if (tree == NULL) 286 return NULL; 287 288 while(tree->right != NULL) 289 tree = tree->right; 290 return tree; 291 } 292 293 template <class T> 294 T AVLTree<T>::maximum() 295 { 296 AVLTreeNode<T> *p = maximum(mRoot); 297 if (p != NULL) 298 return p->key; 299 300 return (T)NULL; 301 } 302 303 /* 304 * LL:左左对应的情况(左单旋转)。 305 * 306 * 返回值:旋转后的根节点 307 */ 308 template <class T> 309 AVLTreeNode<T>* AVLTree<T>::leftLeftRotation(AVLTreeNode<T>* k2) 310 { 311 AVLTreeNode<T>* k1; 312 313 k1 = k2->left; 314 k2->left = k1->right; 315 k1->right = k2; 316 317 k2->height = max( height(k2->left), height(k2->right)) + 1; 318 k1->height = max( height(k1->left), k2->height) + 1; 319 320 return k1; 321 } 322 323 /* 324 * RR:右右对应的情况(右单旋转)。 325 * 326 * 返回值:旋转后的根节点 327 */ 328 template <class T> 329 AVLTreeNode<T>* AVLTree<T>::rightRightRotation(AVLTreeNode<T>* k1) 330 { 331 AVLTreeNode<T>* k2; 332 333 k2 = k1->right; 334 k1->right = k2->left; 335 k2->left = k1; 336 337 k1->height = max( height(k1->left), height(k1->right)) + 1; 338 k2->height = max( height(k2->right), k1->height) + 1; 339 340 return k2; 341 } 342 343 /* 344 * LR:左右对应的情况(左双旋转)。 345 * 346 * 返回值:旋转后的根节点 347 */ 348 template <class T> 349 AVLTreeNode<T>* AVLTree<T>::leftRightRotation(AVLTreeNode<T>* k3) 350 { 351 k3->left = rightRightRotation(k3->left); 352 353 return leftLeftRotation(k3); 354 } 355 356 /* 357 * RL:右左对应的情况(右双旋转)。 358 * 359 * 返回值:旋转后的根节点 360 */ 361 template <class T> 362 AVLTreeNode<T>* AVLTree<T>::rightLeftRotation(AVLTreeNode<T>* k1) 363 { 364 k1->right = leftLeftRotation(k1->right); 365 366 return rightRightRotation(k1); 367 } 368 369 /* 370 * 将结点插入到AVL树中,并返回根节点 371 * 372 * 参数说明: 373 * tree AVL树的根结点 374 * key 插入的结点的键值 375 * 返回值: 376 * 根节点 377 */ 378 template <class T> 379 AVLTreeNode<T>* AVLTree<T>::insert(AVLTreeNode<T>* &tree, T key) 380 { 381 if (tree == NULL) 382 { 383 // 新建节点 384 tree = new AVLTreeNode<T>(key, NULL, NULL); 385 if (tree==NULL) 386 { 387 cout << "ERROR: create avltree node failed!" << endl; 388 return NULL; 389 } 390 } 391 else if (key < tree->key) // 应该将key插入到"tree的左子树"的情况 392 { 393 tree->left = insert(tree->left, key); 394 // 插入节点后,若AVL树失去平衡,则进行相应的调节。 395 if (height(tree->left) - height(tree->right) == 2) 396 { 397 if (key < tree->left->key) 398 tree = leftLeftRotation(tree); 399 else 400 tree = leftRightRotation(tree); 401 } 402 } 403 else if (key > tree->key) // 应该将key插入到"tree的右子树"的情况 404 { 405 tree->right = insert(tree->right, key); 406 // 插入节点后,若AVL树失去平衡,则进行相应的调节。 407 if (height(tree->right) - height(tree->left) == 2) 408 { 409 if (key > tree->right->key) 410 tree = rightRightRotation(tree); 411 else 412 tree = rightLeftRotation(tree); 413 } 414 } 415 else //key == tree->key) 416 { 417 cout << "添加失败:不允许添加相同的节点!" << endl; 418 } 419 420 tree->height = max( height(tree->left), height(tree->right)) + 1; 421 422 return tree; 423 } 424 425 template <class T> 426 void AVLTree<T>::insert(T key) 427 { 428 insert(mRoot, key); 429 } 430 431 /* 432 * 删除结点(z),返回根节点 433 * 434 * 参数说明: 435 * tree AVL树的根结点 436 * z 待删除的结点 437 * 返回值: 438 * 根节点 439 */ 440 template <class T> 441 AVLTreeNode<T>* AVLTree<T>::remove(AVLTreeNode<T>* &tree, AVLTreeNode<T>* z) 442 { 443 // 根为空 或者 没有要删除的节点,直接返回NULL。 444 if (tree==NULL || z==NULL) 445 return NULL; 446 447 if (z->key < tree->key) // 待删除的节点在"tree的左子树"中 448 { 449 tree->left = remove(tree->left, z); 450 // 删除节点后,若AVL树失去平衡,则进行相应的调节。 451 if (height(tree->right) - height(tree->left) == 2) 452 { 453 AVLTreeNode<T> *r = tree->right; 454 if (height(r->left) > height(r->right)) 455 tree = rightLeftRotation(tree); 456 else 457 tree = rightRightRotation(tree); 458 } 459 } 460 else if (z->key > tree->key)// 待删除的节点在"tree的右子树"中 461 { 462 tree->right = remove(tree->right, z); 463 // 删除节点后,若AVL树失去平衡,则进行相应的调节。 464 if (height(tree->left) - height(tree->right) == 2) 465 { 466 AVLTreeNode<T> *l = tree->left; 467 if (height(l->right) > height(l->left)) 468 tree = leftRightRotation(tree); 469 else 470 tree = leftLeftRotation(tree); 471 } 472 } 473 else // tree是对应要删除的节点。 474 { 475 // tree的左右孩子都非空 476 if ((tree->left!=NULL) && (tree->right!=NULL)) 477 { 478 if (height(tree->left) > height(tree->right)) 479 { 480 // 如果tree的左子树比右子树高; 481 // 则(01)找出tree的左子树中的最大节点 482 // (02)将该最大节点的值赋值给tree。 483 // (03)删除该最大节点。 484 // 这类似于用"tree的左子树中最大节点"做"tree"的替身; 485 // 采用这种方式的好处是:删除"tree的左子树中最大节点"之后,AVL树仍然是平衡的。 486 AVLTreeNode<T>* max = maximum(tree->left); 487 tree->key = max->key; 488 tree->left = remove(tree->left, max); 489 } 490 else 491 { 492 // 如果tree的左子树不比右子树高(即它们相等,或右子树比左子树高1) 493 // 则(01)找出tree的右子树中的最小节点 494 // (02)将该最小节点的值赋值给tree。 495 // (03)删除该最小节点。 496 // 这类似于用"tree的右子树中最小节点"做"tree"的替身; 497 // 采用这种方式的好处是:删除"tree的右子树中最小节点"之后,AVL树仍然是平衡的。 498 AVLTreeNode<T>* min = maximum(tree->right); 499 tree->key = min->key; 500 tree->right = remove(tree->right, min); 501 } 502 } 503 else 504 { 505 AVLTreeNode<T>* tmp = tree; 506 tree = (tree->left!=NULL) ? tree->left : tree->right; 507 delete tmp; 508 } 509 } 510 511 return tree; 512 } 513 514 template <class T> 515 void AVLTree<T>::remove(T key) 516 { 517 AVLTreeNode<T>* z; 518 519 if ((z = search(mRoot, key)) != NULL) 520 mRoot = remove(mRoot, z); 521 } 522 523 /* 524 * 销毁AVL树 525 */ 526 template <class T> 527 void AVLTree<T>::destroy(AVLTreeNode<T>* &tree) 528 { 529 if (tree==NULL) 530 return ; 531 532 if (tree->left != NULL) 533 destroy(tree->left); 534 if (tree->right != NULL) 535 destroy(tree->right); 536 537 delete tree; 538 } 539 540 template <class T> 541 void AVLTree<T>::destroy() 542 { 543 destroy(mRoot); 544 } 545 546 /* 547 * 打印"二叉查找树" 548 * 549 * key -- 节点的键值 550 * direction -- 0,表示该节点是根节点; 551 * -1,表示该节点是它的父结点的左孩子; 552 * 1,表示该节点是它的父结点的右孩子。 553 */ 554 template <class T> 555 void AVLTree<T>::print(AVLTreeNode<T>* tree, T key, int direction) 556 { 557 if(tree != NULL) 558 { 559 if(direction==0) // tree是根节点 560 cout << setw(2) << tree->key << " is root" << endl; 561 else // tree是分支节点 562 cout << setw(2) << tree->key << " is " << setw(2) << key << "'s " << setw(12) << (direction==1?"right child" : "left child") << endl; 563 564 print(tree->left, tree->key, -1); 565 print(tree->right,tree->key, 1); 566 } 567 } 568 569 template <class T> 570 void AVLTree<T>::print() 571 { 572 if (mRoot != NULL) 573 print(mRoot, mRoot->key, 0); 574 } 575 #endif
AVL树的测试程序(AVLTreeTest.cpp)

1 /** 2 * C 语言: AVL树 3 * 4 * @author skywang 5 * @date 2013/11/07 6 */ 7 8 #include <iostream> 9 #include "AVLTree.h" 10 using namespace std; 11 12 static int arr[]= {3,2,1,4,5,6,7,16,15,14,13,12,11,10,8,9}; 13 #define TBL_SIZE(a) ( (sizeof(a)) / (sizeof(a[0])) ) 14 15 int main() 16 { 17 int i,ilen; 18 AVLTree<int>* tree=new AVLTree<int>(); 19 20 cout << "== 依次添加: "; 21 ilen = TBL_SIZE(arr); 22 for(i=0; i<ilen; i++) 23 { 24 cout << arr[i] <<" "; 25 tree->insert(arr[i]); 26 } 27 28 cout << "\n== 前序遍历: "; 29 tree->preOrder(); 30 31 cout << "\n== 中序遍历: "; 32 tree->inOrder(); 33 34 cout << "\n== 后序遍历: "; 35 tree->postOrder(); 36 cout << endl; 37 38 cout << "== 高度: " << tree->height() << endl; 39 cout << "== 最小值: " << tree->minimum() << endl; 40 cout << "== 最大值: " << tree->maximum() << endl; 41 cout << "== 树的详细信息: " << endl; 42 tree->print(); 43 44 i = 8; 45 cout << "\n== 删除根节点: " << i; 46 tree->remove(i); 47 48 cout << "\n== 高度: " << tree->height() ; 49 cout << "\n== 中序遍历: " ; 50 tree->inOrder(); 51 cout << "\n== 树的详细信息: " << endl; 52 tree->print(); 53 54 // 销毁二叉树 55 tree->destroy(); 56 57 return 0; 58 }
AVL树的C++测试程序
AVL树的测试程序代码(AVLTreeTest.cpp)在前面已经给出。在测试程序中,首先新建一棵AVL树,然后依次添加"3,2,1,4,5,6,7,16,15,14,13,12,11,10,8,9" 到AVL树中;添加完毕之后,再将8从AVL树中删除。AVL树的添加和删除过程如下图:
(01) 添加3,2
添加3,2都不会破坏AVL树的平衡性。
(02) 添加1
添加1之后,AVL树失去平衡(LL),此时需要对AVL树进行旋转(LL旋转)。旋转过程如下:
(03) 添加4
添加4不会破坏AVL树的平衡性。
(04) 添加5
添加5之后,AVL树失去平衡(RR),此时需要对AVL树进行旋转(RR旋转)。旋转过程如下:
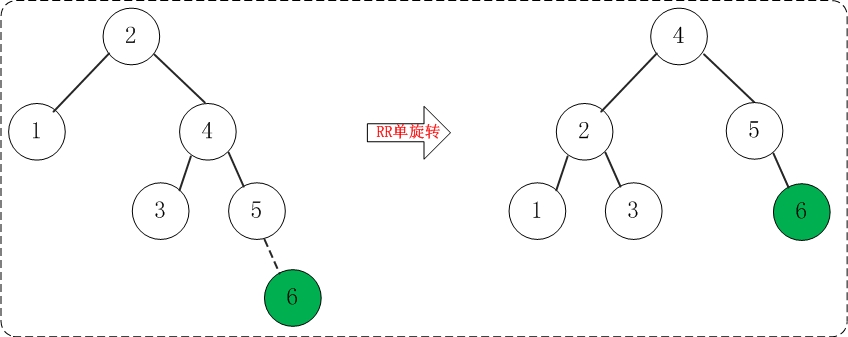
(05) 添加6
添加6之后,AVL树失去平衡(RR),此时需要对AVL树进行旋转(RR旋转)。旋转过程如下:
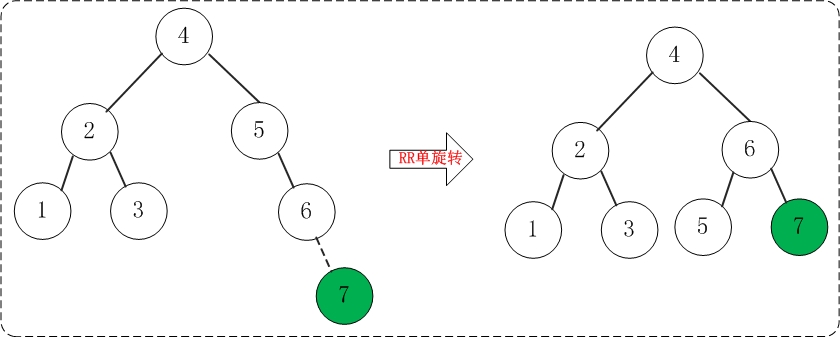
(06) 添加7
添加7之后,AVL树失去平衡(RR),此时需要对AVL树进行旋转(RR旋转)。旋转过程如下:
(07) 添加16
添加16不会破坏AVL树的平衡性。
(08) 添加15
添加15之后,AVL树失去平衡(RR),此时需要对AVL树进行旋转(RR旋转)。旋转过程如下:
(09) 添加14
添加14之后,AVL树失去平衡(RL),此时需要对AVL树进行旋转(RL旋转)。旋转过程如下:
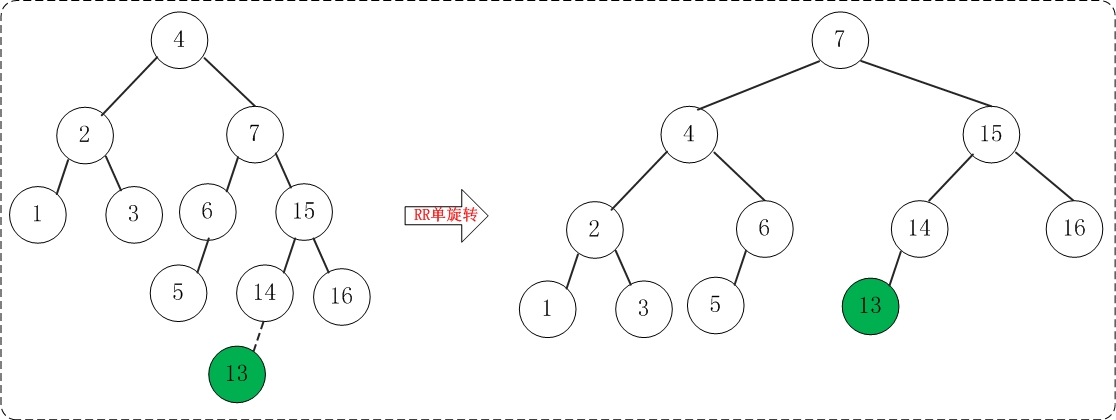
(10) 添加13
添加13之后,AVL树失去平衡(RR),此时需要对AVL树进行旋转(RR旋转)。旋转过程如下:
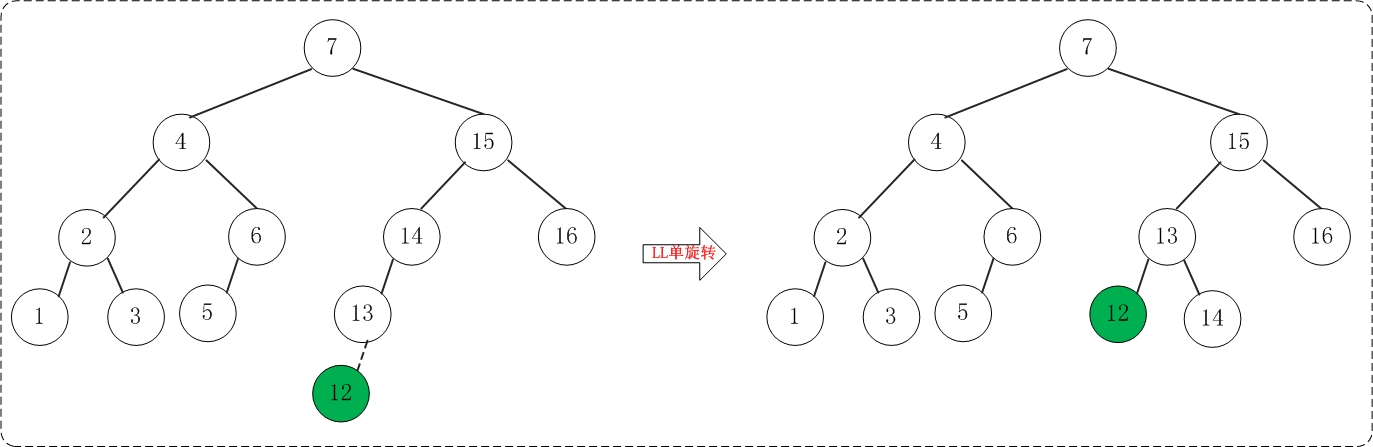
(11) 添加12
添加12之后,AVL树失去平衡(LL),此时需要对AVL树进行旋转(LL旋转)。旋转过程如下:
(12) 添加11
添加11之后,AVL树失去平衡(LL),此时需要对AVL树进行旋转(LL旋转)。旋转过程如下:
(13) 添加10
添加10之后,AVL树失去平衡(LL),此时需要对AVL树进行旋转(LL旋转)。旋转过程如下:
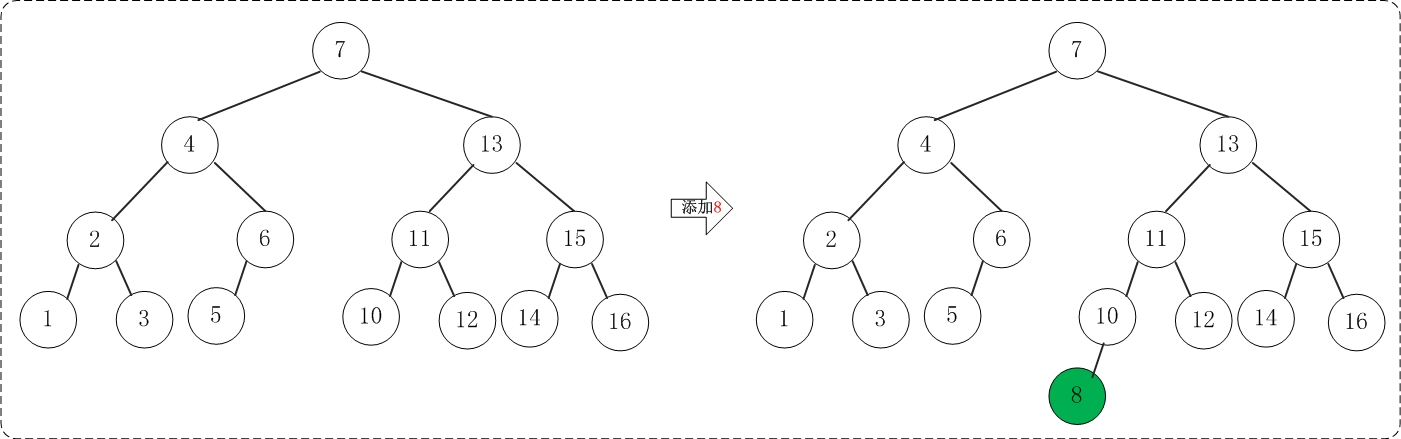
(14) 添加8
添加8不会破坏AVL树的平衡性。
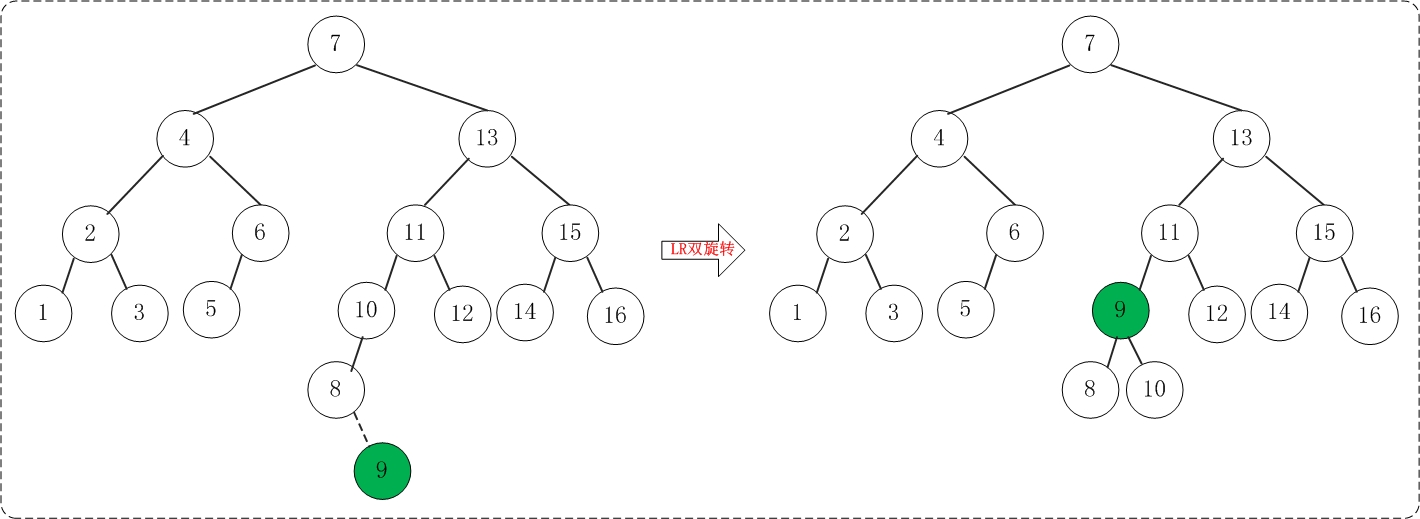
(15) 添加9
但是添加9之后,AVL树失去平衡(LR),此时需要对AVL树进行旋转(LR旋转)。旋转过程如下:
添加完所有数据之后,得到的AVL树如下:
接着,删除节点8.删除节点8并不会造成AVL树的不平衡,所以不需要旋转,操作示意图如下:
程序运行结果如下:
== 依次添加: 3 2 1 4 5 6 7 16 15 14 13 12 11 10 8 9 == 前序遍历: 7 4 2 1 3 6 5 13 11 9 8 10 12 15 14 16 == 中序遍历: 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 == 后序遍历: 1 3 2 5 6 4 8 10 9 12 11 14 16 15 13 7 == 高度: 5 == 最小值: 1 == 最大值: 16 == 树的详细信息: is root is 7's left child is 4's left child is 2's left child is 2's right child is 4's right child is 6's left child is 7's right child is 13's left child is 11's left child is 9's left child is 9's right child is 11's right child is 13's right child is 15's left child is 15's right child == 删除根节点: 8 == 高度: 5 == 中序遍历: 1 2 3 4 5 6 7 9 10 11 12 13 14 15 16 == 树的详细信息: is root is 7's left child is 4's left child is 2's left child is 2's right child is 4's right child is 6's left child is 7's right child is 13's left child is 11's left child is 9's right child is 11's right child is 13's right child is 15's left child is 15's right child