Codeforces Round #319 (Div. 1) C. Points on Plane 分块
C. Points on Plane
Time Limit: 1 Sec
Memory Limit: 256 MB
题目连接
http://codeforces.com/contest/576/problem/CDescription
On a plane are n points (xi, yi) with integer coordinates between 0 and 106. The distance between the two points with numbers a and bis said to be the following value: 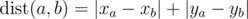 (the distance calculated by such formula is called Manhattan distance).
(the distance calculated by such formula is called Manhattan distance).
We call a hamiltonian path to be some permutation pi of numbers from 1 to n. We say that the length of this path is value 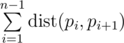 .
.
Find some hamiltonian path with a length of no more than 25 × 108. Note that you do not have to minimize the path length.
Input
The first line contains integer n (1 ≤ n ≤ 106).
The i + 1-th line contains the coordinates of the i-th point: xi and yi (0 ≤ xi, yi ≤ 106).
It is guaranteed that no two points coincide.
Output
Print the permutation of numbers pi from 1 to n — the sought Hamiltonian path. The permutation must meet the inequality 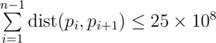 .
.
If there are multiple possible answers, print any of them.
It is guaranteed that the answer exists.
Sample Input
5
0 7
8 10
3 4
5 0
9 12
Sample Output
4 3 1 2 5
HINT
In the sample test the total distance is:
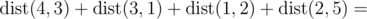
(|5 - 3| + |0 - 4|) + (|3 - 0| + |4 - 7|) + (|0 - 8| + |7 - 10|) + (|8 - 9| + |10 - 12|) = 2 + 4 + 3 + 3 + 8 + 3 + 1 + 2 = 26
题意
给你一个曼哈顿距离的图,然后要求你找到一个链,链穿了所有的点
然后要求这链的长度<=25*10e8
题解:
就分块咯,分成1000块,每个块内y坐标最多走10e6长度,x坐标最多走n*10e3个,n表示一块内的点数
n是一个二次函数维护的东西……所以大概答案最后就是10e3(10e6+10e6) = 2*10e9
所以大概看看脸,就能把这道题AC了
代码:
//qscqesze #include <cstdio> #include <cmath> #include <cstring> #include <ctime> #include <iostream> #include <algorithm> #include <set> #include <bitset> #include <vector> #include <sstream> #include <queue> #include <typeinfo> #include <fstream> #include <map> #include <stack> typedef long long ll; using namespace std; //freopen("D.in","r",stdin); //freopen("D.out","w",stdout); #define sspeed ios_base::sync_with_stdio(0);cin.tie(0) #define maxn 1000500 #define mod 1001 #define eps 1e-9 #define pi 3.1415926 int Num; //const int inf=0x7fffffff; const ll inf=999999999; inline ll read() { ll x=0,f=1;char ch=getchar(); while(ch<'0'||ch>'9'){if(ch=='-')f=-1;ch=getchar();} while(ch>='0'&&ch<='9'){x=x*10+ch-'0';ch=getchar();} return x*f; } //************************************************************************************* pair<int,pair<int,int> > p[maxn]; int main() { int n=read(); for(int i=0;i<n;i++) { p[i].first = read();p[i].first/=1050; p[i].second.first = read();p[i].second.second = i; } sort(p,p+n); for(int i=0;i<n;i++) printf("%d ",p[i].second.second+1); }



