【初识——树状数组】 区间求和

说树状数组其实是一个索引表，但是是一个特殊的，树状的索引表，它利用了二进制的一些特性。

 

就区间求和的要求来说：

首先我们用a[]数组来存储原始数据。然后在a[]之上构造c[]数组来作为树状数组。

如图

 

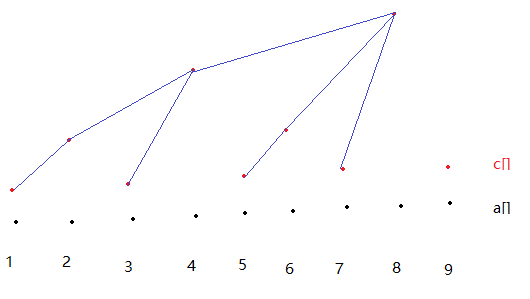
这个图表示，当i为奇数时，c[i]中保存的都是a[i]本身。然后，c[2]中保存了a[1], a[2]，共2个，c[4]中保存的是a[1], a[2], a[3], a[4]，c[6]又是保存两个，c[5]和c[6]。c[8]保存8个,c[1], c[2], c[3], c[4], c[5], c[6], c[7], c[8]。

看出什么规律没有？

如果将这些下标差成2进制序列就容易看出来规律了——

如果从右向左看，第一个是1的数位是第1位，那么c[i]中只有1个数，如果第一个是1的数位式第2位，那么c[i]中有2个数，如果是3，那么c[i]中有4个数，以此类推。

但是怎么代码怎么实现呢？

我们可以这样做——

首先取这个数的下标i，然后取这个数按位数最右边的1加在i上，所得就是下一个需要加当前数的下标，以此类推，直到取到下标大于等于最大下标n。

代码——

void Add(int x, int y)
{
    a[x] += y;
    while(x <= n)
    {
        c[x] += y;
        x += lowbit(x);
    }
}

 

 

当然，给某个数加上减上多少也可以用这个做。

 

区间求和的方法：

取从i到j之间的区间和

一种方法是取从1到j的和，然后减去从1到i的和。

取的方法——

从j开始，然后j减去j按位数最右端的1，得到的就是下一个需要的下标，减到0时结束。

 

int Sum(int x)
{
    int rt = 0;
    while(x > 0)
    {
        rt += c[x];
        x -= lowbit(x);
    }
    return rt;
}

printf("%d\n", Sum(b)-Sum(a-1));

 

另一种方法是从j开始，如果j减去从右往左第一个1的差小于i，则结果加a[j]，然后j -= 1；否则结果加c[j], j减去从右向左第一个1。

 

int Summ(int l, int r)
{
    int rt = 0;
    while(r >= l)
    {
        if(r-lowbit(r) < l)
        {
            rt += a[r];
            r -= 1;
        }
        else
        {
            rt += c[r];
            r -= lowbit(r);
        }
    }
    return rt;
}

 

不过这个貌似较慢……

 

完整代码——

#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
using namespace std;

const int N = 50005;

int t, n;
int a[N];
int c[N];
char s[10];
int x, y;

int lowbit(int x)
{
    return x&(-x);
}

void Add(int x, int y)
{
    a[x] += y;
    while(x <= n)
    {
        c[x] += y;
        x += lowbit(x);
    }
}

int Sum(int x)
{
    int rt = 0;
    while(x > 0)
    {
        rt += c[x];
        x -= lowbit(x);
    }
    return rt;
}

int Summ(int l, int r)
{
    int rt = 0;
    while(r >= l)
    {
        if(r-lowbit(r) < l)
        {
            rt += a[r];
            r -= 1;
        }
        else
        {
            rt += c[r];
            r -= lowbit(r);
        }
    }
    return rt;
}

void Query(int a, int b)
{
    printf("%d\n", Sum(b)-Sum(a-1));
    //printf("%d\n", Summ(a, b));
}

int main()
{
    //freopen("test.in", "r", stdin);
    scanf("%d", &t);
    for(int tm = 1; tm <= t; tm++)
    {
        scanf("%d", &n);
        memset(c, 0, sizeof(c));
        memset(a, 0, sizeof(a));
        int y;
        for(int i = 1; i <= n; i++)
        {
            scanf("%d", &y);
            Add(i, y);
        }
        printf("Case %d:\n", tm);

        scanf("%s", s);
        while(s[0] != 'E')
        {
            scanf("%d%d", &x, &y);
            if(s[0] == 'A') Add(x, y);
            if(s[0] == 'Q') Query(x, y);
            if(s[0] == 'S') Add(x, -y);
            scanf("%s", s);
        }
    }
}