Ural 1519 Formula 1 (插头DP）

1519. Formula 1

Time Limit: 1.0 second
Memory Limit: 16 MB

Background

Regardless of the fact, that Vologda could not get rights to hold the Winter Olympic games of 20**, it is well-known, that the city will conduct one of the Formula 1 events. Surely, for such an important thing a new race circuit should be built as well as hotels, restaurants, international airport - everything for Formula 1 fans, who will flood the city soon. But when all the hotels and a half of the restaurants were built, it appeared, that at the site for the future circuit a lot of gophers lived in their holes. Since we like animals very much, ecologists will never allow to build the race circuit over the holes. So now the mayor is sitting sadly in his office and looking at the map of the circuit with all the holes plotted on it.

Problem

Who will be smart enough to draw a plan of the circuit and keep the city from inevitable disgrace? Of course, only true professionals - battle-hardened programmers from the first team of local technical university!.. But our heroes were not looking for easy life and set much more difficult problem: "Certainly, our mayor will be glad, if we find how many ways of building the circuit are there!" - they said.

It should be said, that the circuit in Vologda is going to be rather simple. It will be a rectangle N*M cells in size with a single circuit segment built through each cell. Each segment should be parallel to one of rectangle's sides, so only right-angled bends may be on the circuit. At the picture below two samples are given for N = M = 4 (gray squares mean gopher holes, and the bold black line means the race circuit). There are no other ways to build the circuit here.

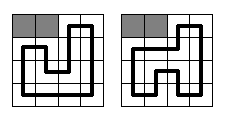
Input

The first line contains the integer numbers N and M (2 ≤ N, M ≤ 12). Each of the next N lines contains M characters, which are the corresponding cells of the rectangle. Character "." (full stop) means a cell, where a segment of the race circuit should be built, and character "*" (asterisk) - a cell, where a gopher hole is located.

Output

You should output the desired number of ways. It is guaranteed, that it does not exceed 2⁶³-1.

Samples

input	output
4 4 **.. .... .... ....	2
4 4 .... .... .... ....	6

 

 

具体看cdq的论文：http://wenku.baidu.com/view/ed2b3e23482fb4daa58d4b74.html

 

插头DP入门题：

/*
最小表示法
*/

#include<stdio.h>
#include<iostream>
#include<string.h>
#include<algorithm>
using namespace std;

const int MAXD=15;
const int HASH=30007;
const int STATE=1000010;
using namespace std;
int N,M;
int maze[MAXD][MAXD];
int code[MAXD];
int ch[MAXD];//最小表示法使用
int ex,ey;//最后一个非障碍格子的坐标
struct HASHMAP
{
    int head[HASH],next[STATE],size;
    long long state[STATE];
    long long f[STATE];
    void init()
    {
        size=0;
        memset(head,-1,sizeof(head));
    }
    void push(long long st,long long ans)
    {
        int i;
        int h=st%HASH;
        for(i=head[h];i!=-1;i=next[i])//这里要注意是next
          if(state[i]==st)
          {
              f[i]+=ans;
              return;
          }
        state[size]=st;
        f[size]=ans;
        next[size]=head[h];
        head[h]=size++;
    }
}hm[2];
void decode(int *code,int m,long long  st)
{
    for(int i=m;i>=0;i--)
    {
        code[i]=st&7;
        st>>=3;
    }
}
long long encode(int *code,int m)//最小表示法
{
    int cnt=1;
    memset(ch,-1,sizeof(ch));
    ch[0]=0;
    long long st=0;
    for(int i=0;i<=m;i++)
    {
        if(ch[code[i]]==-1)ch[code[i]]=cnt++;
        code[i]=ch[code[i]];
        st<<=3;
        st|=code[i];
    }
    return st;
}
void shift(int *code,int m)
{
    for(int i=m;i>0;i--)code[i]=code[i-1];
    code[0]=0;
}
void dpblank(int i,int j,int cur)
{
    int k,left,up;
    for(k=0;k<hm[cur].size;k++)
    {
        decode(code,M,hm[cur].state[k]);
        left=code[j-1];
        up=code[j];
        if(left&&up)
        {
            if(left==up)//只能出现在最后一个非障碍格子
            {
                if(i==ex&&j==ey)
                {
                    code[j-1]=code[j]=0;
                    if(j==M)shift(code,M);
                    hm[cur^1].push(encode(code,M),hm[cur].f[k]);
                }
            }
            else//不在同一个连通分量则合并
            {
                code[j-1]=code[j]=0;
                for(int t=0;t<=M;t++)
                  if(code[t]==up)
                    code[t]=left;
                if(j==M)shift(code,M);
                hm[cur^1].push(encode(code,M),hm[cur].f[k]);
            }
        }
        else if((left&&(!up))||((!left)&&up))
        {
            int t;
            if(left)t=left;
            else t=up;
            if(maze[i][j+1])
            {
                code[j-1]=0;
                code[j]=t;
                hm[cur^1].push(encode(code,M),hm[cur].f[k]);
            }
            if(maze[i+1][j])
            {
                code[j-1]=t;
                code[j]=0;
                if(j==M)shift(code,M);
                hm[cur^1].push(encode(code,M),hm[cur].f[k]);
            }
        }
        else//无插头，则构造新的连通块
        {
            if(maze[i][j+1]&&maze[i+1][j])
            {
                code[j-1]=code[j]=13;
                hm[cur^1].push(encode(code,M),hm[cur].f[k]);
            }
        }
    }
}
void dpblock(int i,int j,int cur)
{
    int k;
    for(k=0;k<hm[cur].size;k++)
    {
        decode(code,M,hm[cur].state[k]);
        code[j-1]=code[j]=0;
        if(j==M)shift(code,M);
        hm[cur^1].push(encode(code,M),hm[cur].f[k]);
    }
}
char str[MAXD];
void init()
{
    memset(maze,0,sizeof(maze));

    ex=0;
    for(int i=1;i<=N;i++)
    {
        scanf("%s",&str);
        for(int j=0;j<M;j++)
        {
            if(str[j]=='.')
            {
                ex=i;
                ey=j+1;
                maze[i][j+1]=1;
            }
        }
    }
}
void solve()
{
    int i,j,cur=0;
    long long ans=0;
    hm[cur].init();
    hm[cur].push(0,1);
    for(i=1;i<=N;i++)
      for(j=1;j<=M;j++)
      {
          hm[cur^1].init();
          if(maze[i][j])dpblank(i,j,cur);
          else  dpblock(i,j,cur);
          cur^=1;
      }
    for(i=0;i<hm[cur].size;i++)
      ans+=hm[cur].f[i];
    printf("%I64d\n",ans);
}
int main()
{
    freopen("in.txt","r",stdin);
    freopen("out.txt","w",stdout);
    while(scanf("%d%d",&N,&M)!=EOF)
    {
        init();
        if(ex==0)//没有空的格子
        {
            printf("0\n");
            continue;
        }
        solve();
    }
    return 0;
}