codeforces 651C Watchmen

Watchmen are in a danger and Doctor Manhattan together with his friend Daniel Dreiberg should warn them as soon as possible. There are n watchmen on a plane, the i-th watchman is located at point (x_i, y_i).

They need to arrange a plan, but there are some difficulties on their way. As you know, Doctor Manhattan considers the distance between watchmen i and j to be |x_i - x_j| + |y_i - y_j|. Daniel, as an ordinary person, calculates the distance using the formula .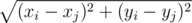

The success of the operation relies on the number of pairs (i, j) (1 ≤ i < j ≤ n), such that the distance between watchman i and watchmen j calculated by Doctor Manhattan is equal to the distance between them calculated by Daniel. You were asked to compute the number of such pairs.

题目大意：发现有一些点的欧氏距离和曼哈顿距离是相等的,他认为这个现象特别有趣。为了发现一些规律和性质,他给出了 n 个点,想知道这些点中有多少对点的欧氏距离与曼哈顿距离相等

Input

The first line of the input contains the single integer n (1 ≤ n ≤ 200 000) — the number of watchmen.

Each of the following n lines contains two integers x_i and y_i (|x_i|, |y_i| ≤ 10⁹).

Some positions may coincide.

Output

Print the number of pairs of watchmen such that the distance between them calculated by Doctor Manhattan is equal to the distance calculated by Daniel.

Examples

Input

3
1 1
7 5
1 5

Output

2

Input

6
0 0
0 1
0 2
-1 1
0 1
1 1

Output

11

Note

In the first sample, the distance between watchman 1 and watchman 2 is equal to |1 - 7| + |1 - 5| = 10 for Doctor Manhattan and 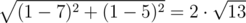for Daniel. For pairs (1, 1), (1, 5) and (7, 5), (1, 5) Doctor Manhattan and Daniel will calculate the same distances.

 

比较容易发现,两个点 A 和 B 能够贡献答案当且仅当 A 和 B 有至少一维坐标相等。于是
我们离散化以后对于每个相同的 x 和 y 坐标统计一下答案就好了。
注意可能有坐标相同的点,这些点对会在 x 坐标和 y 坐标上都贡献一次答案,所以要去重。
时间复杂度 O(n log n) 。

 

#include<iostream>
#include<cstdio>
#include<cstring>
#include<algorithm>
#include<map>
using namespace std;
struct Pair
{
  int x,y;
  bool operator <(const Pair &z)
  const
  {
    return (x<z.x||(x==z.x&&y<z.y));  
  }
};
map<Pair,int>Map;
int X[400005],Y[400005],a[400005],x[200005],y[200005],n,sz;
long long ans;
int main()
{int i;
  cin>>n;
  for (i=1;i<=n;i++)
    {
      scanf("%d%d",&x[i],&y[i]);
      a[i]=x[i];a[n+i]=y[i];
    }
  sz=unique(a+1,a+2*n+1)-(a+1);
  sort(a+1,a+sz+1);
  for (i=1;i<=n;i++)
    {
      x[i]=lower_bound(a+1,a+sz+1,x[i])-a;
      y[i]=lower_bound(a+1,a+sz+1,y[i])-a;
    }
  for (i=1;i<=n;i++)
    {
      if (Map.count((Pair){x[i],y[i]})) ans-=Map[(Pair){x[i],y[i]}];
    ans+=X[x[i]]+Y[y[i]];
      if (Map.count((Pair){x[i],y[i]}))
    Map[(Pair){x[i],y[i]}]++;
    else Map[(Pair){x[i],y[i]}]=1;
      X[x[i]]++;Y[y[i]]++;
    }
  cout<<ans;
}