Codefoeces-689D Friends and Subsequences

Friends and Subsequences

time limit per test

2 seconds

memory limit per test

512 megabytes

input

standard input

output

standard output

Mike and !Mike are old childhood rivals, they are opposite in everything they do, except programming. Today they have a problem they cannot solve on their own, but together (with you) — who knows?

Every one of them has an integer sequences a and b of length n. Being given a query of the form of pair of integers (l, r), Mike can instantly tell the value of 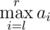 while !Mike can instantly tell the value of .

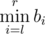Now suppose a robot (you!) asks them all possible different queries of pairs of integers (l, r) (1 ≤ l ≤ r ≤ n) (so he will make exactly n(n + 1) / 2 queries) and counts how many times their answers coincide, thus for how many pairs 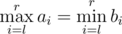 is satisfied.

How many occasions will the robot count?

Input

The first line contains only integer n (1 ≤ n ≤ 200 000).

The second line contains n integer numbers a1, a2, ..., an ( - 109 ≤ ai ≤ 109) — the sequence a.

The third line contains n integer numbers b1, b2, ..., bn ( - 109 ≤ bi ≤ 109) — the sequence b.

Output

Print the only integer number — the number of occasions the robot will count, thus for how many pairs 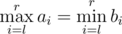 is satisfied.

Examples

input

Copy

6
1 2 3 2 1 4
6 7 1 2 3 2

output

Copy

2

input

Copy

3
3 3 3
1 1 1

output

Copy

0

Note

The occasions in the first sample case are:

1.l = 4,r = 4 since max{2} = min{2}.

2.l = 4,r = 5 since max{2, 1} = min{2, 3}.

There are no occasions in the second sample case since Mike will answer 3 to any query pair, but !Mike will always answer 1.

题意:给出两个长度为n的序列，求有哪些区间相同的  a区间最大值==b区间最小值

思路：预处理出ST表,然后我们来遍历1~n每次我们查询最左的符合要求的右端点，再查询出最右的符合要求的右端点。这样最右-最左就为符合的区间，累加即可。我们在查询端点时，二分查询就行。

#include<bits/stdc++.h>
using namespace std;
typedef long long ll;
const int maxn = 201000;
ll Max[maxn][30],Min[maxn][30],b[maxn],a[maxn],n;
void GetST()
{
    for(int i=1; i<=n; i++)
    {
        Max[i][0]=a[i];
        Min[i][0]=b[i];
    }
    for(int j=1; (1<<j)<=n; j++)
        for(int i=1; i+(1<<j)-1<=n; i++)
        {
            int k=j-1;
            Max[i][j]=max(Max[i][k],Max[i+(1<<k)][k]);
            Min[i][j]=min(Min[i][k],Min[i+(1<<k)][k]);
        }
}
ll getmax(int l,int r)
{
    int k=(int)(log(r-l+1)/log(2.0));
    return max(Max[l][k],Max[r-(1<<k)+1][k]);
}
ll getmin(int l,int r)
{
    int k=(int)(log(r-l+1)/log(2.0));
    return min(Min[l][k],Min[r-(1<<k)+1][k]);
}
int main()
{
    scanf("%d",&n);
    for(int i=1; i<=n; i++)
        scanf("%lld",&a[i]);
    for(int i=1; i<=n; i++)
        scanf("%lld",&b[i]);
    GetST();
    ll ans=0;
    int l,r,m;
    int idl,idr;
    for(int i=1; i<=n; i++)
    {
        l=i;
        r=n;
        idl=0,idr=0;
        while(l<=r)
        {
            m=(l+r)>>1;
            ll Maxx=getmax(i,m);
            ll Minx=getmin(i,m);
            if(Maxx==Minx)
            {
                idr=m;
                l=m+1;
            }//查询最右
            else if(Maxx<Minx)
                l=m+1;
            else r=m-1;
        }
        if(idr)
        {
            l=i;
            r=n;
            while(l<=r)
            {
                m=(l+r)>>1;
                ll Maxx=getmax(i,m);
                ll Minx=getmin(i,m);
                if(Maxx==Minx)
                {
                    idl=m;
                    r=m-1;
                }//查询最左
                else if(Maxx<Minx)
                    l=m+1;
                else r=m-1;
            }
            ans+=idr-idl+1;
        }
    }
    printf("%lld\n",ans);
}

 

 

 

PS：摸鱼怪的博客分享，欢迎感谢各路大牛的指点~